
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(2x^2+100x-10000=0\)
\(\Leftrightarrow2x^2+200x-100x-10000=0\)
\(\Leftrightarrow2x\left(x+100\right)-100\left(x+100\right)=0\)
\(\Leftrightarrow\left(2x-100\right)\left(x+100\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}2x-100=0\\x+100=0\end{cases}}\)\(\Leftrightarrow\orbr{\begin{cases}x=50\\x=-100\end{cases}}\)


\(\Rightarrow x^8-1250x^4+390625-100x^2-1=0\)
\(\Rightarrow x^8-1250x^4-100x^2+390624=0\)
\(\Rightarrow\left(x^2-26\right)\left(x^2-24\right)\left(x^4+50x^2+626\right)=0\)
Vì x4 + 50x2 + 626 > 0
\(\Rightarrow x^2-26=0\Rightarrow x=+-\sqrt{26}\)
hoặc \(x^2-24=0\Rightarrow x=+-\sqrt{24}\)
Vậy pt có 4 nghiệm .............................

x^3-99x^2-100x
<=>x^3-x^2+100x^2-100x=0
<=>x^2(x-1)+100x(x-1)=0
<=>(x-1)(x^2+100x)=0
<=> giải tiếp ra hoặc x=1;x=0;x=-100
Bài này bấm nghiệm trên máy tính cx ra luôn mà
x^3+99x^2-100x=0
=>x(x^2+99x-100)=0
=>
- x=0
- x^2+99x-100=0 dùng đen ta giải ra


a: \(A=\dfrac{1}{x-1}\cdot5\sqrt{3}\cdot\left|x-1\right|\cdot\sqrt{x-1}\)
\(=\dfrac{5\sqrt{3}}{x-1}\cdot\left(x-1\right)\cdot\sqrt{x-1}=5\sqrt{3}\cdot\sqrt{x-1}\)
b: \(B=10\sqrt{x}-3\cdot\dfrac{10\sqrt{x}}{3}-\dfrac{4}{x}\cdot\dfrac{x\sqrt{x}}{2}\)
\(=10\sqrt{x}-10\sqrt{x}-\dfrac{4\sqrt{x}}{2}=-2\sqrt{x}\)
c: \(C=x-4+\left|x-4\right|\)
=x-4+x-4
=2x-8

Do VT là tổng của các giá trị tuyệt đối nên \(\ge0\Rightarrow100x\ge0\Rightarrow x\ge0\)
\(PT\Leftrightarrow\left(x+x+x+...+x\right)+\left(1+2+3+...+99\right)=100x\) (có 99x số x)
\(\Leftrightarrow99x+4950=100x\Leftrightarrow100x-99x=x=4950\)
Vậy \(x=4950\)
Dễ thấy \(x\ge0\)
\(\Rightarrow x+1+x+2+x+3+...+x+99=100x\)
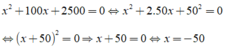
\(PT\Leftrightarrow\left(x-1\right)\left(x+101\right)=0\Leftrightarrow\orbr{\begin{cases}x=1\\x=-101\end{cases}}\)
\(x^2+100x-101=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+101\right)=0\)
\(\Rightarrow\hept{\begin{cases}x-1=0\hept{x=1}\\x+101=0\hept{x=-101}\end{cases}}\)
\(\Rightarrow\hept{\begin{cases}x=1\\x=101\end{cases}}\)