
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(=\dfrac{x+2+x-\sqrt{x}-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{2}{\sqrt{x}-1}\)
\(=\dfrac{2}{x+\sqrt{x}+1}\)

Câu 21:
1: \(\Leftrightarrow5\sqrt{x-1}=10\)
=>căn x-1=2
=>x-1=4
=>x=5
2: Để hai đường song song thì -2m=3m-5
=>-5m=-5
=>m=1

\(=\sqrt{3\left(x^2-2x+1\right)+25}\supseteq\sqrt{3\left(x+1\right)^2+25}\supseteq5\)
min=5 <=>x=-1
\(\text{Đặt }A=\sqrt{3x^2-6x+28}=\sqrt{3x^2-6x+3+25}\)
\(=\sqrt{3.\left(x^2-2x+1\right)+25}=\sqrt{3.\left(x-1\right)^2+25}\)
\(\Rightarrow A^2=3.\left(x-1\right)^2+25\ge25\Rightarrow A\ge\sqrt{25}=5\)
Dấu "=" xảy ra khi : x=1
Vậy GTNN của A là 5 tại x=1

Đặt \(\frac{3m^2-2m+1}{\left(m+1\right)^2}=a\)\(\Leftrightarrow3m^2-2m-1=a\left(m+1\right)^2=am^2+2am+a\)
\(\Leftrightarrow3m^2-am^2-2m-2am+1-a=0\)
\(\Leftrightarrow\left(3-a\right)m^2-\left(2+2a\right)m+1-a=0\)
\(\Delta=\left(2+2a\right)^2-4\left(1-a\right)\left(3-a\right)=24a-8\)
Để pt có nghiệm:\(24a-8\ge0\Leftrightarrow a\ge\frac{1}{3}\)
Vậy bt ban đầu đạt GTNN là 1/3 khi m=1/2


Nếu đề bài là giải phương trình thì :
\(\sqrt{x+3}=\sqrt{x-3}\)
Đk : \(x\ge3\)
Bình phương hai vế :
\(\Rightarrow x+3=x-3\)
\(x+3-x+3=0\)
\(0x=-6\)
\(\Rightarrow\)phương trình vô nghiệm

Bài 2
a, bạn tự vẽ
b, Hoành độ giao điểm tm pt
\(2x^2-2x+3=0\)
\(\Delta'=1-3.2=-5< 0\)
Vậy pt vô nghiệm hay (d) ko cắt (P)
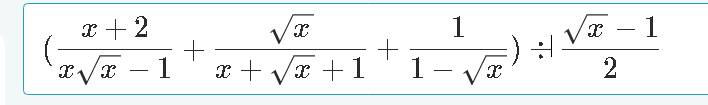 giúp mjk vs mjk đg cần gấp ạ
giúp mjk vs mjk đg cần gấp ạ 
