
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\Rightarrow4^x-3.2^{x+1}+2=\sqrt{2}^{2\left(x+2\right)}\)
\(\Leftrightarrow4^x-6.2^x+2=2^{x+2}=4.2^x\)
Đặt \(2^x=a>0\Rightarrow a^2-6a+2=4a\)
\(\Leftrightarrow a^2-10a+2=0\Rightarrow\left[{}\begin{matrix}a=5+\sqrt{23}\\a=5-\sqrt{23}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}2^x=5+\sqrt{23}\\2^x=5-\sqrt{23}\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=log_2\left(5+\sqrt{23}\right)\\x=log_2\left(5-\sqrt{23}\right)\end{matrix}\right.\)

a) Đặt t = 13x > 0 ta được phương trình:
13t2 – t – 12 = 0 ⇔ (t – 1)(13t + 12) = 0
⇔ t = 1 ⇔ 13x = 1 ⇔ x = 0
b)
Chia cả hai vế phương trình cho 9x ta được phương trình tương đương
(1+(23)x)(1+3.(23)x)=8.(23)x(1+(23)x)(1+3.(23)x)=8.(23)x
Đặt t=(23)xt=(23)x (t > 0) , ta được phương trình:
(1 + t)(1 + 3t) = 8t ⇔ 3t2 – 4t + 1 = 0 ⇔ t∈{13,1}t∈{13,1}
Với t=13t=13 ta được nghiệm x=log2313x=log2313
Với t = 1 ta được nghiệm x = 0
c) Điều kiện: x > 2
Vì nên phương trình đã cho tương đương với:
[log3(x−2)=0log5x=1⇔[x=3x=5[log3(x−2)=0log5x=1⇔[x=3x=5
d) Điều kiện: x > 0
log22x – 5log2x + 6 = 0
⇔(log2x – 2)(log2x – 3) = 0
⇔ x ∈ {4, 8}



d) Phương trình đã cho tương đương với :
\(2^{3x}+2^x.3^{2x}=2.3^{2x}\Leftrightarrow\left(\frac{2}{3}\right)^{2x}+\left(\frac{2}{3}\right)^x-2=0\)
Đặt \(t=\left(\frac{2}{3}\right)^x,\left(t>0\right)\) Phương trình trở thành
\(t^3+t-2=0\) hay \(\left(t-1\right)\left(t^2+t+2\right)=0\)
Do \(t^2+t+2=\left(t+\frac{1}{2}\right)^2+\frac{7}{4}>0\) nên \(t-1=0\) hay t=1
Từ đó suy ra \(\left(\frac{2}{3}\right)^x=1=\left(\frac{2}{3}\right)^0\Leftrightarrow x=0\)
Vậy phương trình có nghiệm duy nhất \(x=0\)
c) Điều kiện \(x\ne0\). Chia cả 2 vế của phương trình cho \(6^{\frac{1}{x}}>0\), ta có :
\(6.\left(\frac{3}{2}\right)^{\frac{1}{x}}-13.1+6\left(\frac{2}{3}\right)^{\frac{1}{x}}=0\)
Đặt \(t=\left(\frac{3}{2}\right)^{\frac{1}{x}},\left(t>0\right)\)
Phương trình trở thành
\(6t-13+\frac{6}{t}=0\) hay \(6t^2-13t+6=0\)
Phương trình bậc 2 trên có 2 nghiệm dương \(t=\frac{3}{2},t=\frac{2}{3}\)
Với \(t=\frac{3}{2}\) thì \(\left(\frac{3}{2}\right)^{\frac{1}{x}}=\frac{3}{2}\Leftrightarrow\frac{1}{x}=1\Leftrightarrow x=1\)
Với \(t=\frac{2}{3}\) thì \(\left(\frac{3}{2}\right)^{\frac{1}{x}}=\frac{2}{3}\Leftrightarrow\frac{1}{x}=-1\Leftrightarrow x=-1\)

\(\Leftrightarrow x^4-a^4+a^2x^2-a^4=0\)
\(\Leftrightarrow\left(x^2-a^2\right)\left(x^2+a^2\right)+a^2\left(x^2-a^2\right)=0\)
\(\Leftrightarrow\left(x^2-a^2\right)\left(x^2+2a^2\right)=0\)
\(\Leftrightarrow x=\pm a\)
Chia 2 vế cho \(a^4\) thì pt trở thành:
\(\frac{x^4}{a^4}+\frac{x^2}{a^2}-2=0\)
Đặt \(\frac{x^2}{a^2}=t\ge0\Rightarrow t^2+t-2=0\Rightarrow\left[{}\begin{matrix}t=1\\t=-2\left(l\right)\end{matrix}\right.\)
\(\Rightarrow\frac{x^2}{a^2}=1\)
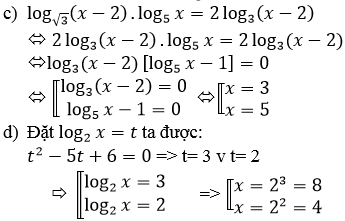
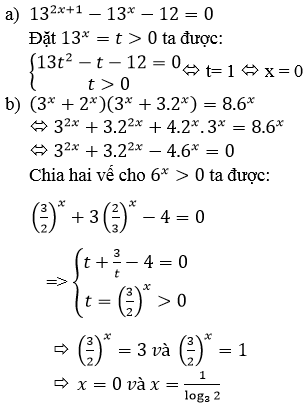

\(\Leftrightarrow2^{-3}.2^{2x}-3.2^{-2}.2^x+1=0\)
\(\Leftrightarrow\dfrac{1}{8}2^{2x}-\dfrac{3}{4}2^x+1=0\)
Đặt \(2^x=t>0\)
\(\Rightarrow\dfrac{1}{8}t^2-\dfrac{3}{4}t+1=0\Rightarrow\left[{}\begin{matrix}t=4\\t=2\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}2^x=4\\2^x=2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=2\\x=1\end{matrix}\right.\)