
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


tan3x.tanx = 1 (Điều kiện: 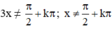 )
)
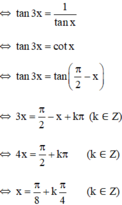
Các nghiệm thuộc họ nghiệm trên đều thỏa mãn điều kiện.
Vậy phương trình có họ nghiệm 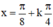 (k ∈ Z).
(k ∈ Z).

a.
\(\Leftrightarrow4cos^32x-3cos2x+3cos2x=\sqrt{2}\)
\(\Leftrightarrow cos^32x=\dfrac{\sqrt{2}}{4}\)
\(\Leftrightarrow cos2x=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow2x=\pm\dfrac{\pi}{4}+k2\pi\)
\(\Leftrightarrow x=\pm\dfrac{\pi}{8}+k\pi\) (\(k\in Z\))
c.
ĐKXĐ: \(\left\{{}\begin{matrix}cos3x\ne0\\cosx\ne0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{3}\\x\ne\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(tan3x.tanx=1\)
\(\Rightarrow tan3x=\dfrac{1}{tanx}\)
\(\Rightarrow tan3x=cotx\)
\(\Rightarrow tan3x=tan\left(\dfrac{\pi}{2}-x\right)\)
\(\Rightarrow3x=\dfrac{\pi}{2}-x+k\pi\)
\(\Rightarrow x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\)

a: ĐKXĐ: sin 2x<>1
=>2x<>pi/2+k2pi
=>x<>pi/4+kpi
\(\dfrac{cos2x}{sin2x-1}=0\)
=>cos2x=0
=>2x=pi/2+kpi
=>x=pi/4+kpi/2
Kết hợp ĐKXĐ, ta được:
x=3/4pi+k2pi hoặc x=7/4pi+k2pi
b: cos(sinx)=1
=>sin x=kpi
=>sin x=0
=>x=kpi
c: \(2\cdot sin^2x-1+cos3x=0\)
=>cos3x+cos2x=0
=>cos3x=-cos2x=-sin(pi/2-2x)=sin(2x-pi/2)
=>cos3x=cos(pi/2-2x+pi/2)=cos(pi-2x)
=>3x=pi-2x+k2pi hoặc 3x=-pi+2x+k2pi
=>x=-pi+k2pi hoặc x=pi/5+k2pi/5
e: cos3x=-cos7x
=>cos3x=cos(pi-7x)
=>3x=pi-7x+k2pi hoặc 3x=-pi+7x+k2pi
=>x=pi/10+kpi/5 hoặc x=pi/4-kpi/2

Nhận thấy tanx=0 không phải nghiệm
\(\Leftrightarrow tan3x=\frac{1}{tanx}\)
\(\Leftrightarrow tan3x=cotx=tan\left(\frac{\pi}{2}-x\right)\)
\(\Rightarrow3x=\frac{\pi}{2}-x+k\pi\)
\(\Rightarrow x=\frac{\pi}{8}+\frac{k\pi}{4}\)
\(\Rightarrow x=\frac{\pi}{8}+\frac{k\pi}{4}\) với \(k=\left\{0;1;2...;7\right\}\)

\(a,f'\left(x\right)=3x^2-6x\\ f'\left(x\right)\le0\Leftrightarrow3x^2-6x\le0\\ \Leftrightarrow3x\left(x-2\right)\le0\Leftrightarrow0\le x\le2\)
Lời giải:
a. $f'(x)\leq 0$
$\Leftrightarrow 3x^2-6x\leq 0$
$\Leftrightarrow x(x-2)\leq 0$
$\Leftrightarrow 0\leq x\leq 2$
b.
$f'(x)=x^2-3x+2=0$
$\Leftrightarrow 3x^2-6x=x^2-3x+2=0$
$\Leftrightarrow 3x(x-2)=(x-1)(x-2)=0$
$\Leftrightarrow x-2=0$
$\Leftrightarrow x=2$
c.
$g(x)=f(1-2x)+x^2-x+2022$
$g'(x)=(1-2x)'f(1-2x)'_{1-2x}+2x-1$
$=-2[3(1-2x)^2-6(1-2x)]+2x-1$
$=-24x^2+2x+5$
$g'(x)\geq 0$
$\Leftrightarrow -24x^2+2x+5\geq 0$
$\Leftrightarrow (5-12x)(2x-1)\geq 0$
$\Leftrightarrow \frac{-5}{12}\leq x\leq \frac{1}{2}$
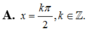
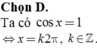
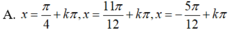
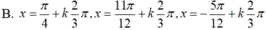
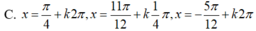
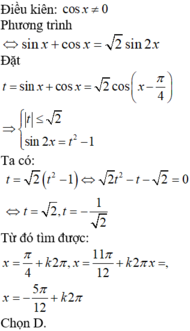
ĐKXĐ: \(\left\{{}\begin{matrix}cos3x\ne0\\cosx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\frac{sin3x.sinx}{cos3x.cosx}=1\)
\(\Leftrightarrow sin3x.sinx-cos3x.cosx=0\)
\(\Leftrightarrow sin2x=0\)
\(\Leftrightarrow2sinx.cosx=0\)
\(\Leftrightarrow sinx=0\Leftrightarrow x=k\pi\)