Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Pt hoành độ giao điểm: \(x^2-2mx+m=2x-1\)
\(\Leftrightarrow x^2-2\left(m+1\right)x+m+1=0\)
\(\Delta'=\left(m+1\right)^2-\left(m+1\right)>0\Leftrightarrow\left[{}\begin{matrix}m>0\\m< -1\end{matrix}\right.\) (1)
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=m+1\end{matrix}\right.\)
\(x_1^2+x_2^2\le12\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2\le12\)
\(\Leftrightarrow4\left(m+1\right)^2-2\left(m+1\right)-12\le0\)
\(\Leftrightarrow2m^2+3m-5\le0\Rightarrow-\frac{5}{2}\le m\le1\) (2)
Kết hợp (1); (2) \(\Rightarrow\left[{}\begin{matrix}-\frac{5}{2}\le m< -1\\0< m\le1\end{matrix}\right.\)

\(x_1=0\Rightarrow m=\frac{2019}{2}\Rightarrow x_2=\frac{2019}{2}\) thỏa mãn
Để \(0< x_1< 1< x_2\)
\(\Leftrightarrow y\left(0\right).y\left(1\right)< 0\)
\(\Leftrightarrow\left(2m-2019\right)\left(m-2018\right)< 0\)
\(\Rightarrow\frac{2019}{2}< m< 2018\)
Vậy \(\frac{2019}{2}\le m< 2018\)

\(\Delta=\left(m+2\right)^2-4\left(m^2+1\right)>0\Rightarrow-3m^2+4m>0\Rightarrow0< m< \frac{4}{3}\)
Theo Viet ta có: \(\left\{{}\begin{matrix}x_1+x_2=m+2\\x_1x_2=m^2+1\end{matrix}\right.\)
\(A=x_1^3+x_2^3+x_1^2+x_2^2\)
\(=\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)+\left(x_1+x_2\right)^2-2x_1x_2\)
\(=\left(m+2\right)^3-3\left(m^2+1\right)\left(m+2\right)+\left(m+2\right)^2-2\left(m^2+1\right)\)
\(=-2m^3-m^2+13m+4\)
Bạn coi lại đề, biểu thức trên ko có GTLN hay GTNN trên khoảng \(\left(0;\frac{4}{3}\right)\)
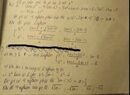

Phương trình hoành độ giao điểm:
\(x^2+6x=2x-m+2\Leftrightarrow x^2+4x+m-2=0\) (1)
\(\Delta'=4-\left(m-2\right)=6-m>0\Rightarrow m< 6\)
Theo định lý Viet: \(\left\{{}\begin{matrix}x_1+x_2=-4\\x_1x_2=m-2\end{matrix}\right.\)
\(x_1^3+x_2^3\ge4\)
\(\Leftrightarrow\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)\ge4\)
\(\Leftrightarrow\left(-4\right)^3+12\left(m-2\right)\ge4\)
\(\Leftrightarrow12m\ge92\Rightarrow m\ge\frac{23}{3}\)
Vậy ko tồn tại m thỏa mãn?