Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
1) Áp dụng bất đẳng thức AM - GM và bất đẳng thức Schwarz:
\(P=\dfrac{1}{a}+\dfrac{1}{\sqrt{ab}}\ge\dfrac{1}{a}+\dfrac{1}{\dfrac{a+b}{2}}\ge\dfrac{4}{a+\dfrac{a+b}{2}}=\dfrac{8}{3a+b}\ge8\).
Đẳng thức xảy ra khi a = b = \(\dfrac{1}{4}\).
2.
\(4=a^2+b^2\ge\dfrac{1}{2}\left(a+b\right)^2\Rightarrow a+b\le2\sqrt{2}\)
Đồng thời \(\left(a+b\right)^2\ge a^2+b^2\Rightarrow a+b\ge2\)
\(M\le\dfrac{\left(a+b\right)^2}{4\left(a+b+2\right)}=\dfrac{x^2}{4\left(x+2\right)}\) (với \(x=a+b\Rightarrow2\le x\le2\sqrt{2}\) )
\(M\le\dfrac{x^2}{4\left(x+2\right)}-\sqrt{2}+1+\sqrt{2}-1\)
\(M\le\dfrac{\left(2\sqrt{2}-x\right)\left(x+4-2\sqrt{2}\right)}{4\left(x+2\right)}+\sqrt{2}-1\le\sqrt{2}-1\)
Dấu "=" xảy ra khi \(x=2\sqrt{2}\) hay \(a=b=\sqrt{2}\)
3. Chia 2 vế giả thiết cho \(x^2y^2\)
\(\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{x^2}+\dfrac{1}{y^2}-\dfrac{1}{xy}\ge\dfrac{1}{4}\left(\dfrac{1}{x}+\dfrac{1}{y}\right)^2\)
\(\Rightarrow0\le\dfrac{1}{x}+\dfrac{1}{y}\le4\)
\(A=\left(\dfrac{1}{x}+\dfrac{1}{y}\right)\left(\dfrac{1}{x^2}+\dfrac{1}{y^2}-\dfrac{1}{xy}\right)=\left(\dfrac{1}{x}+\dfrac{1}{y}\right)^2\le16\)
Dấu "=" xảy ra khi \(x=y=\dfrac{1}{2}\)
\(x^2+2x-3=0\) có nghiệm \(x=1\) nên giới hạn đã cho hữu hạn khi \(2x^2+ax+b=0\) cũng có nghiệm \(x=1\)
\(\Rightarrow2.1^2+a.1+b=0\Rightarrow a+b+2=0\Rightarrow b=-a-2\)
Thay vào:
\(\lim\limits_{x\rightarrow1}\dfrac{2x^2+ax-a-2}{x^2+2x-3}=\lim\limits_{x\rightarrow1}\dfrac{\left(x-1\right)\left(2x+2\right)+a\left(x-1\right)}{\left(x-1\right)\left(x+3\right)}\)
\(=\lim\limits_{x\rightarrow1}\dfrac{\left(x-1\right)\left(2x+2+a\right)}{\left(x-1\right)\left(x+3\right)}=\lim\limits_{x\rightarrow1}\dfrac{2x+2+a}{x+3}=\dfrac{4+a}{4}=\dfrac{3}{4}\)
\(\Rightarrow4+a=3\Rightarrow a=-1\Rightarrow b=-a-2=-1\)
1) ta có: A= x^3 -8y^3=> A=(x-2y)(x^2 +2xy+4y^2)=>A=5.(29+2xy) (vì x-2y=5 và x^2+4y^2=29) (1)
Mặt khác : x-2y=5(gt)=> (x-2y)^2=25=> x^2-4xy+4y^2=25=>29-4xy=25(vì x^2+4y^2=29)
=> xy=1 (2)
Thay (2) vào (1) ta đc: A= 5.(29+2.1)=155
Vậy gt của bt A là 155
2) theo bài ra ta có: a+b+c=0 => a+b=-c=>(a+b)^2=c^2=> a^2 +b^2+2ab=c^2=>c^2-a^2-b^2=2ab
=> \(\left(c^2-a^2-b^2\right)^2=4a^2b^2\)
=>\(c^4+a^4+b^4-2c^2a^2+2a^2b^2-2b^2c^2=4a^2b^2\)
=>\(a^4+b^4+c^4=2a^2b^2+2b^2c^2+2c^2a^2\)
=>\(2\left(a^4+b^4+c^4\right)=\left(a^2+b^2+c^2\right)^2\)
=> \(a^4+b^4+c^4=\frac{1}{2}\left(a^2+b^2+c^2\right)^2\) (đpcm)
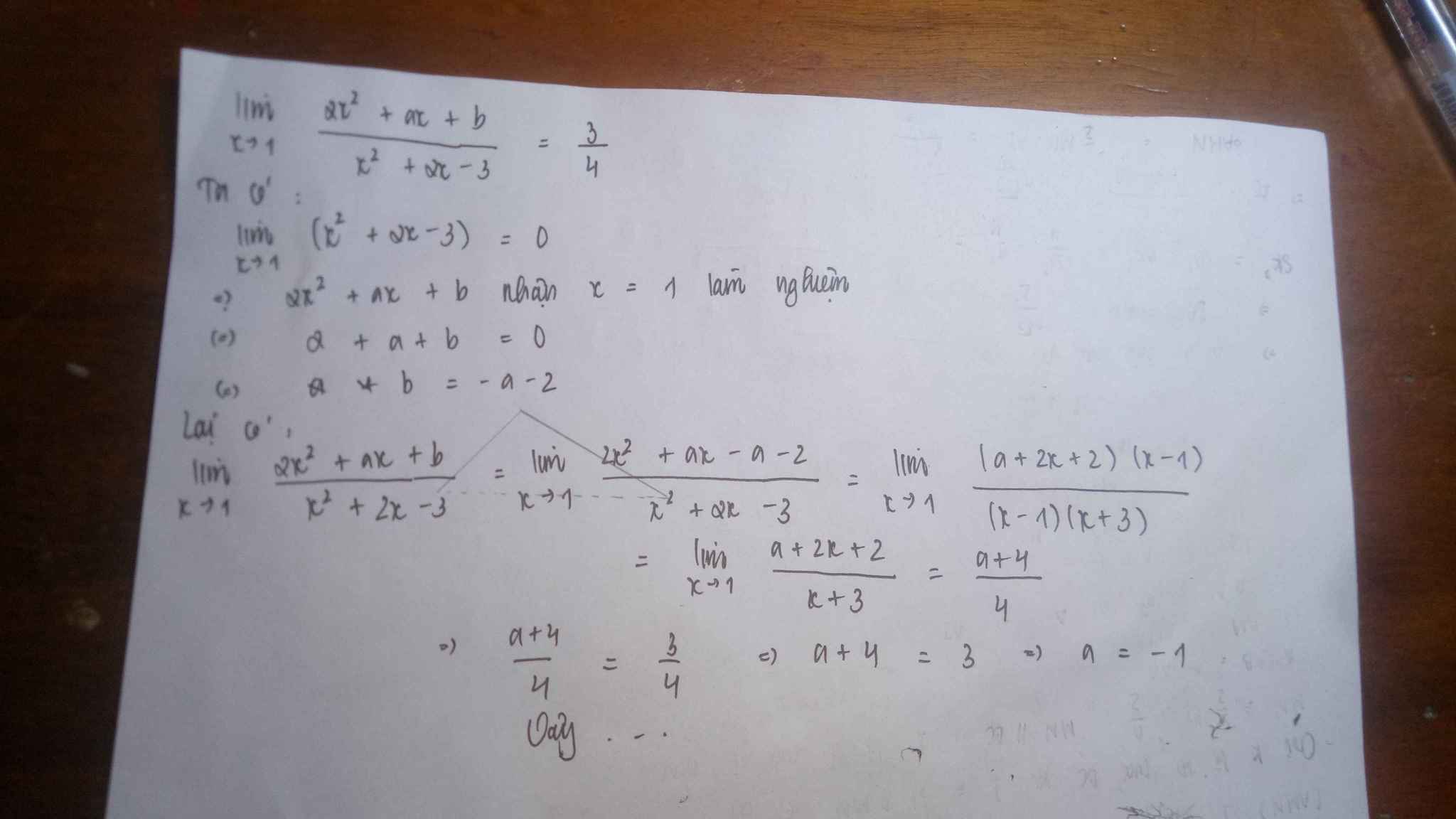
Đáp án D