Tìm x biết : x^2019 = x ^3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DH
1
30 tháng 3 2022
\(\Leftrightarrow\dfrac{x-2}{2020}-1+\dfrac{x-3}{2019}-1=\dfrac{x-2019}{3}-1+\dfrac{x-2020}{2}-1\)
=>x-2022=0
hay x=2022
NN
6
KN
8 tháng 1 2019
\(x+\left(x+1\right)+\left(x+2\right)+\left(x+3\right)+...+\left(x+2019\right)=2019\)
\(\Leftrightarrow x+x+1+x+2+x+3+...+x+2019=2019\)
\(\Leftrightarrow2020x+\left(1+2+3+...+2018\right)+2019=2019\)
\(\Leftrightarrow2020x+\frac{\left(1+2018\right)\times2018}{2}=0\)
\(\Leftrightarrow2020x+2037171=0\)
\(\Leftrightarrow2020x=0-2037171\)
\(\Leftrightarrow2020x=-2037171\)
\(\Leftrightarrow x=\frac{-2037171}{2020}\)
\(\Leftrightarrow x=-1008,5004\)
\(\text{Vậy }x=-1008,5004\)
8 tháng 1 2019
\(x+\left(x+1\right)+\left(x+2\right)+\left(x+3\right)+.....+\left(x+2019\right)=2019\)
\(\left(x+x+x+x+..........+x\right)+\left(1+2+3+......+2019\right)=2019\)
\(2020x+2039190=2019\)
\(2020x=-2037171\)
\(\Leftrightarrow x=\frac{-2037171}{2020}\)
S
29 tháng 12 2020
\(\dfrac{x-1}{2019}+\dfrac{x-2}{2018}+\dfrac{x-3}{2017}=3\)
\(\Leftrightarrow\left(\dfrac{x-1}{2019}-1\right)+\left(\dfrac{x-2}{2018}-1\right)+\left(\dfrac{x-3}{2017}-1\right)=0\)
\(\Leftrightarrow\dfrac{x-1-2019}{2019}+\dfrac{x-2-2018}{2018}+\dfrac{x-3-2017}{2017}=0\)
\(\Leftrightarrow\dfrac{x-2020}{2019}+\dfrac{x-2020}{2018}+\dfrac{x-2020}{2017}=0\)
\(\Leftrightarrow\left(x-2020\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}+\dfrac{1}{2017}\right)=0\)
Vi \(\dfrac{1}{2019}+\dfrac{1}{2018}+\dfrac{1}{2017}\ne0\)
nên \(x-2020=0\)
\(\Leftrightarrow x=2020\)
Vậy ...
3 tháng 5 2019
x−1/2019+x−2/2018=x−3/2017+x−4/2016(đề có thiếu không bạn??)
⇔(x−1/2019−1)+(x−2/2018−1)=(x−3/2017−1)+(x−4/2016−1)
⇔x−2020/2019+x−2020/2018=x−2020/2017+x−2020/2016
⇔x−2020/2019+x−2020/2018−x−2020/2017−x−2020/2016
⇔(x−2020)(1/2019+1/2018−1/2017−1/2016)=0
Mà 1/2019+1/2018−1/2017−1/2016≠0
⇔x−2020=0
⇔x=2020
B
26 tháng 10 2020
\(x^{2019}=x^3\)
\(\Leftrightarrow x^{2019}-x^3=0\)
\(\Leftrightarrow x^3\left(x^{2016}-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=0\\x=1\end{cases}}\)
BL
0
BL
1
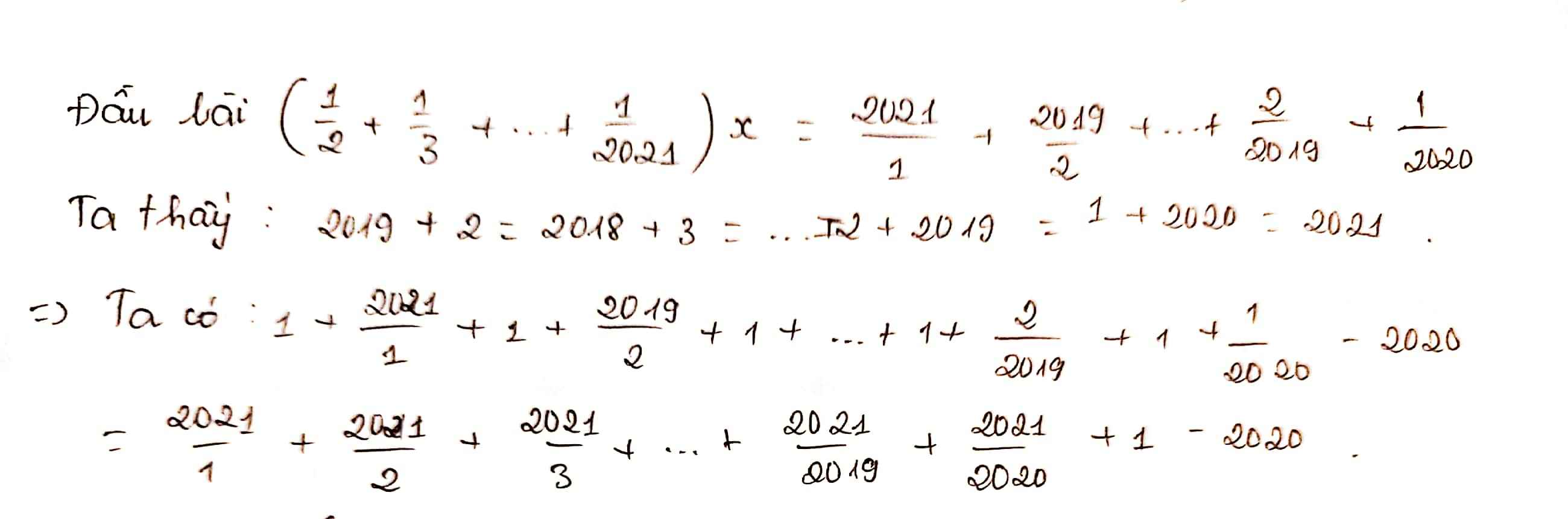
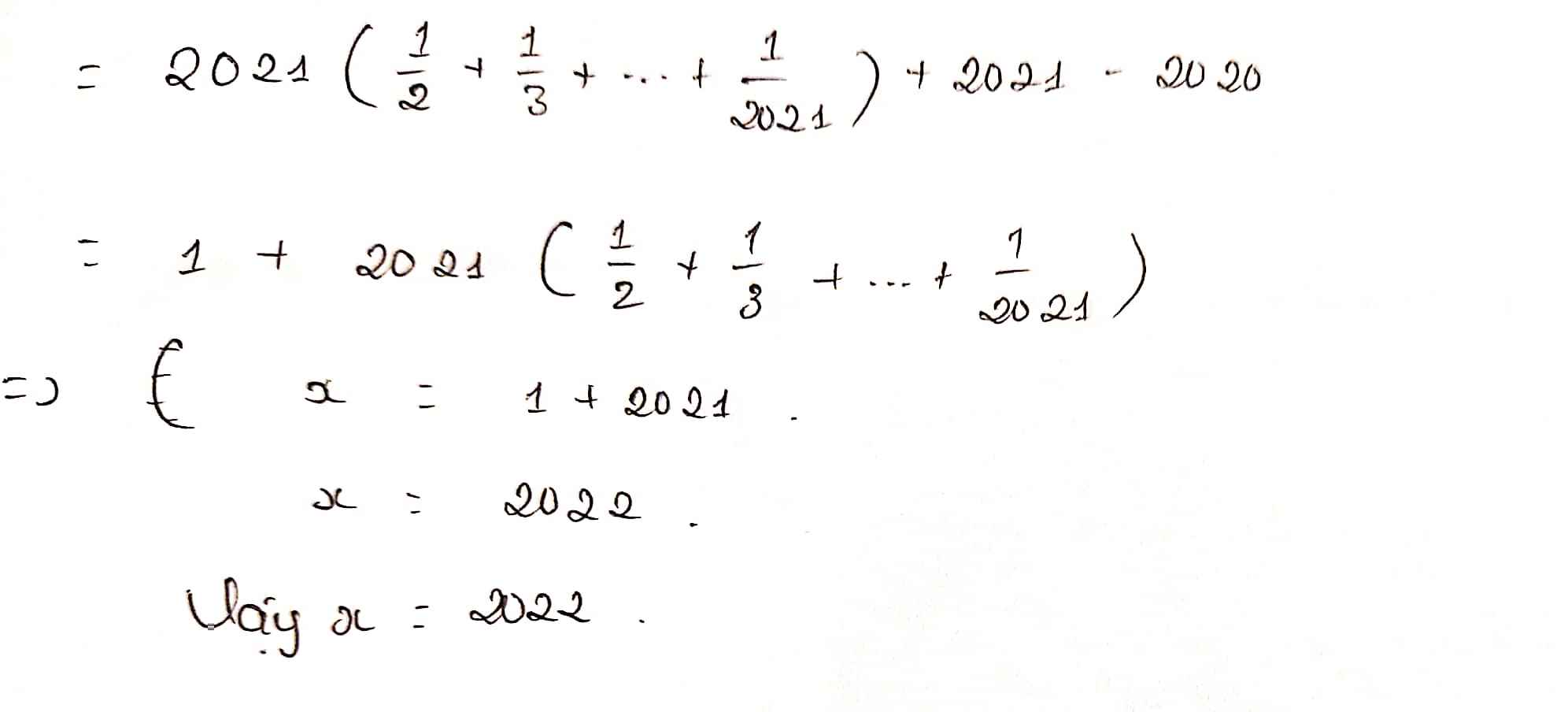
x=1 vì 1^2019=1,x^3=1
hoặc x=0 vì 0^2019=0,0^3=0