(2cos2x+1)(2cos6x+1)(2cos18x+1)=1
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(sinx\left(1+2cos2x+2cos4x+2cos6x\right)\)
\(=sinx+2sinx.cos2x+2sinx.cos4x+2sinx.cos6x\)
\(=sinx+sin3x+sin\left(-x\right)+sin5x+sin\left(-3x\right)+sin7x+sin\left(-5x\right)\)
\(=sinx+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)
\(=sin7x\)
Rút gọn
A= \(\frac{cosx-cos2x-cos3x+cos4x}{sinx-sin2x-sin3x+sin4x}\)
B= sinx(1+2cos2x+2cos4x+2cos6x)

\(A=\frac{cosx-cos3x+cos4x-cos2x}{sinx-sin3x+sin4x-sin2x}=\frac{2sin2x.sinx-2sin3x.sinx}{-2cos2x.sinx+2cos3x.sinx}\)
\(=\frac{sin2x-sin3x}{cos3x-cos2x}=\frac{-2cos\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}{-2sin\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}=cot\left(\frac{5x}{2}\right)\)
\(B=sinx+2cos2x.sinx+2cos4x.sinx+2cos6x.sinx\)
\(=sinx+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)
\(=sin7x\)


P/t \(\Leftrightarrow2cos2x.sin2x-sin2x+2cos^22x-cos2x-1=0\)
\(\Leftrightarrow sin4x-sin2x+cos4x-cos2x=0\)
\(\Leftrightarrow2sinx.cos3x-2sin3x.sinx=0\)
\(\Leftrightarrow sinx\left(cos3x-sin3x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\left(1\right)\\cos3x=sin3x\left(2\right)\end{matrix}\right.\)
(1) \(\Leftrightarrow x=k\pi\left(k\in Z\right)\)
(2) \(\Leftrightarrow sin3x-cos3x=0\) \(\Leftrightarrow\sqrt{2}sin\left(3x-\dfrac{\pi}{4}\right)=0\)
\(\Leftrightarrow3x-\dfrac{\pi}{4}=k\pi\Leftrightarrow x=\dfrac{\pi}{12}+\dfrac{k\pi}{3}\left(k\in Z\right)\)
Vậy ...

Với \(cosx=0\) ko phải nghiệm
Với \(cosx\ne0\)
\(\Rightarrow\left(2sin5x-1\right)\left(2cos2x.cosx-cosx\right)=2sinx.cosx\)
\(\Leftrightarrow\left(2sin5x-1\right)\left(cos3x+cosx-cosx\right)=sin2x\)
\(\Leftrightarrow cos3x\left(2sin5x-1\right)=sin2x\)
\(\Leftrightarrow2sin5x.cos3x-cos3x=sin2x\)
\(\Leftrightarrow sin8x+sin2x-cos3x=sin2x\)
\(\Leftrightarrow sin8x=cos3x=sin\left(\dfrac{\pi}{2}-3x\right)\)
\(\Leftrightarrow...\)

=>\(2\cdot cos2x\cdot sin2x+2cos^22x-sin2x-cos2x-1=0\)
=>\(2cos2x\cdot sin2x+2\cdot cos^22x-1=sin2x+cos2x\)
=>\(sin4x+cos4x=sin2x+cos2x\)
=>\(sin\left(4x+\dfrac{pi}{4}\right)=sin\left(2x+\dfrac{pi}{4}\right)\)
=>4x+pi/4=2x+pi/4+k2pi hoặc 4x+pi/4=pi-2x-pi/4+k2pi
=>2x=k2pi hoặc 6x=1/2pi+k2pi
=>x=kpi hoặc x=1/12pi+kpi/3
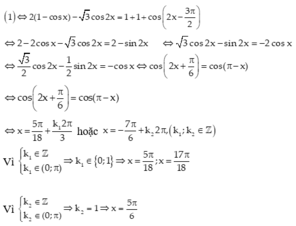
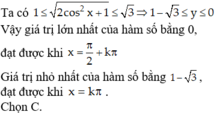
Nhận thấy \(sinx=0\) ko phải nghiệm
\(\Leftrightarrow sinx\left(2cos2x+1\right)\left(2cos6x+1\right)\left(2cos18x+1\right)=sinx\)
\(\Leftrightarrow\left(2cos2x.sinx+sinx\right)\left(2cos6x+1\right)\left(2cos18x+1\right)=sinx\)
\(\Leftrightarrow\left(sin3x-sinx+sinx\right)\left(2cos6x+1\right)\left(2cos18x+1\right)=sinx\)
\(\Leftrightarrow\left(2cos6x.sin3x+sin3x\right)\left(2cos18x+1\right)=sinx\)
\(\Leftrightarrow\left(2cos18x.sin9x+sin9x\right)=sinx\)
\(\Leftrightarrow sin27x=sinx\)