CMR: Pt \(\dfrac{1}{cosx}-\dfrac{1}{sinx}=m\) có nghiệm với mọi m thuộc R, sử dụng hàm số liên tục
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 1
Hàm số xác định trên R khi và chỉ khi:
\(sin^2x+\left(2m-3\right)cosx+3m-2>0;\forall x\in R\)
\(\Leftrightarrow-cos^2x+\left(2m-3\right)cosx+3m-1>0\)
\(\Leftrightarrow t^2-\left(2m-3\right)t-3m+1< 0;\forall t\in\left[-1;1\right]\)
\(\Leftrightarrow t^2+3t+1< m\left(2t+3\right)\)
\(\Leftrightarrow\dfrac{t^2+3t+1}{2t+3}< m\) (do \(2t+3>0;\forall t\in\left[-1;1\right]\))
\(\Leftrightarrow m>\max\limits_{\left[-1;1\right]}\dfrac{t^2+3t+1}{2t+3}\)
Ta có: \(\dfrac{t^2+3t+1}{2t+3}=\dfrac{t^2+t-2+2t+3}{2t+3}=\dfrac{\left(t-1\right)\left(t+2\right)}{2t+3}+1\)
Do \(-1\le t\le1\Rightarrow\dfrac{\left(t-1\right)\left(t+2\right)}{2t+3}\le0\)
\(\Rightarrow\max\limits_{\left[-1;1\right]}\dfrac{t^2+3t+1}{2t+3}=1\)
\(\Rightarrow m>1\)
CM
19 tháng 3 2019
Chọn D.
Phương pháp:
+ Đặt 3 sin x - cos x - 1 2 cos x - sin x + 4 = t biến đổi đưa về a sin x + b cos x = c , phương trình này có nghiệm khi a 2 + b 2 ≥ c 2 từ đó ta tìm ta được điều kiện của t.
+ Dựa vào đồ thị hàm số để xác định điều kiện nghiệm của phương trình f x = f t
Từ đó suy ra điều kiện có nghiệm của phương trình đã cho.
Chú ý rằng nếu hàm f t đồng biến (hoặc nghịch biến) trên (a;b) thì phương trình f u = f v nếu có nghiệm thì đó là nghiệm duy nhất trên a ; b ⇔ u = v
1 tháng 9 2021
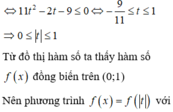
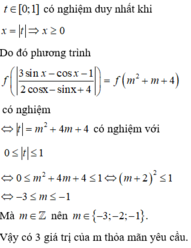
y = \(\dfrac{sin^2x}{cosx\left(sinx-cosx\right)}+\dfrac{1}{4}\)
y = \(\dfrac{sin^2x}{sinx.cosx-cos^2x}+\dfrac{1}{4}=\dfrac{\dfrac{sin^2x}{cos^2x}}{\dfrac{sinx.cosx}{cos^2x}-1}+\dfrac{1}{4}\)
y = \(\dfrac{tan^2x}{tanx-1}+\dfrac{1}{4}\)
y = \(\dfrac{4tan^2x+tanx-1}{4tanx-4}\). Đặt t = tanx. Do x ∈ \(\left(\dfrac{\pi}{4};\dfrac{\pi}{2}\right)\) nên t ∈ (1 ; +\(\infty\))\
Ta đươc hàm số f(t) = \(\dfrac{4t^2+t-1}{4t-4}\)
⇒ ymin = \(\dfrac{17}{4}\) khi t = 2. hay x = arctan(2) + kπ
PA
0
30 tháng 5 2021
C1: \(a.sinx+b.cosx=c\)
Pt vô nghiệm \(\Leftrightarrow a^2+b^2< c^2\)
Bạn áp dụng công thức trên sẽ tìm ra m
C2: (Bạn vẽ đường tròn lượng giác sẽ tìm được)
Hàm số \(y=sinx\) đồng biến trên khoảng \(\left(-\dfrac{\pi}{2}+k2\pi;\dfrac{\pi}{2}+k2\pi\right)\) ( góc phần tư thứ IV và I)
Hàm nghịch biến trên khoảng \(\left(\dfrac{\pi}{2}+k2\pi;\dfrac{3\pi}{2}+k2\pi\right)\)( góc phần tư thứ II và III)
Ý A, khoảng nằm trong góc phần tư thứ III và thứ IV => Hàm nghịch biến sau đó đồng biến
Ý B, khoảng nằm trong góc phần tư thứ I và thứ II => hàm đồng biến sau đó nghịch biến
Ý C, khoảng nằm trong góc phần tư thứ IV; I ; II => hàm đồng biền sau đó nghịch biến
Ý D, khoảng nằm trong phần tư thứ IV ; I=> hàm đồng biến
Đ/A: Ý D
(Toi nghĩ thế)
HP
31 tháng 5 2021
1.
ĐK: \(x\ne\dfrac{k\pi}{2}\)
\(cotx-tanx=sinx+cosx\)
\(\Leftrightarrow\dfrac{cosx}{sinx}-\dfrac{sinx}{cosx}=sinx+cosx\)
\(\Leftrightarrow\dfrac{cos^2x-sin^2x}{sinx.cosx}=sinx+cosx\)
\(\Leftrightarrow\left(\dfrac{cosx-sinx}{sinx.cosx}-1\right)\left(sinx+cosx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx+cosx=0\left(1\right)\\cosx-sinx=sinx.cosx\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow\sqrt{2}sin\left(x+\dfrac{\pi}{4}\right)=0\Leftrightarrow x=-\dfrac{\pi}{4}+k\pi\)
\(\left(2\right)\Leftrightarrow t=\dfrac{1-t^2}{2}\left(t=cosx-sinx,\left|t\right|\le2\right)\)
\(\Leftrightarrow t^2+2t-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=-1+\sqrt{2}\\t=-1-\sqrt{2}\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow cosx-sinx=-1+\sqrt{2}\)
\(\Leftrightarrow-\sqrt{2}sin\left(x-\dfrac{\pi}{4}\right)=-1+\sqrt{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}-1}{\sqrt{2}}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+arcsin\left(\dfrac{\sqrt{2}-1}{\sqrt{2}}\right)+k2\pi\\x=\dfrac{5\pi}{4}-arcsin\left(\dfrac{\sqrt{2}-1}{\sqrt{2}}\right)+k2\pi\end{matrix}\right.\)
Vậy phương trình đã cho có nghiệm:
\(x=-\dfrac{\pi}{4}+k\pi;x=\dfrac{\pi}{4}+arcsin\left(\dfrac{\sqrt{2}-1}{\sqrt{2}}\right)+k2\pi;x=\dfrac{5\pi}{4}-arcsin\left(\dfrac{\sqrt{2}-1}{\sqrt{2}}\right)+k2\pi\)
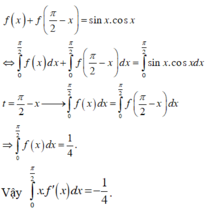
Lời giải:
Ta có: \(\frac{1}{\cos x}-\frac{1}{\sin x}=m\)
\(\Leftrightarrow f(x)=\frac{1}{\cos x}-\frac{1}{\sin x}-m=0\)
Ta thấy: Hàm \(f(x)\) liên tục trên đoạn \(\left[\frac{\pi}{6}; \frac{\pi}{3}\right]\), mà:
\(f\left(\frac{\pi}{3}\right)=\frac{6-2\sqrt{3}}{3}-m\)
\(f\left(\frac{\pi}{6}\right)=\frac{-6+2\sqrt{3}}{3}-m\)
\(\Rightarrow f\left(\frac{\pi}{3}\right)f\left(\frac{\pi}{6}\right)=\left(\frac{6-2\sqrt{3}}{3}-m\right)\left(\frac{-6+2\sqrt{3}}{3}-m\right)=-\left(\frac{6-2\sqrt{3}}{3}-m\right)^2\)
\(\Rightarrow f\left(\frac{\pi}{3}\right)f\left(\frac{\pi}{6}\right)\leq 0\)
Do đó tồn tại ít nhất một nghiệm \(c\in \left[\frac{\pi}{6}; \frac{\pi}{3}\right]\)
Ta có đpcm.