 Help me bài 2 , 3 . Thanks nhiều nhiều ạ
Help me bài 2 , 3 . Thanks nhiều nhiều ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Sai rồi, quan hệ cạnh và góc đối diện không đc áp dụng ở 2 tam giác như bạn làm đâu nhe!

a)h(x)=f(x)-g(x)
=(2x3 +3x2 -2x +3)-(2x3 +3x2 -7x +2)
=2x3 + 3x2 - 2x +3 - 2x3 -3x2 + 7x -2
=5x+1
b)h(x)=5x+1=0
=>5x=-1
x=\(\frac{-1}{5}\)

Ta có :\(A=3+3^2+3^3+...+3^{2008}\)(1)
\(\Rightarrow3A=3^2+3^3+3^4+...+3^{2009}\)(2)
Lấy (2) trừ đi 1 ta có :
\(\Rightarrow2A=3^{2009}-3\)
Ta lại có :
\(2A+3=3^x\)
\(\Rightarrow3^{2009}=3^x\)
\(\Rightarrow x=2009\)

\(\left|2x-3\right|=3-2x\)
\(ĐK:x\le\dfrac{3}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-3=3-2x\\3-2x=3-2x\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\0=0\left(đúng\right)\end{matrix}\right.\)
Vậy \(S=\left\{x\in R;x=\dfrac{3}{2}\right\}\)

a) Tìm h(x) = f(x) - g(x)
f(x) - g(x) = (-2x2 - 3x3 - 5x + 5x3 - x + x2 + 4x + 3 + 4x2) - (2x2 - x3 + 3x + 3x3 + x2 - x - 9x + 2)
= -2x2 - 3x3 - 5x + 5x3 - x + x2 + 4x + 3 + 4x2 - 2x2 + x3 - 3x - 3x3 - x2 + x + 9x - 2
= (-2x2 + x2 + 4x2 - 2x2 - x2) + (-3x3 + 5x3 + x3 - 3x3) + (-5x - x + 4x - 3x + x + 9x) + (3 - 2)
= 5x + 1
Vậy h(x) = 5x + 1
b) Tìm nghiệm của đa thức h(x)
Cho h(x) = 0
\(\Leftrightarrow\) 5x + 1 = 0
5x = 0 + 1
5x = 1
x = \(\dfrac{1}{5}\)
Vậy x = \(\dfrac{1}{5}\) là nghiệm của đa thức h(x).

e: \(\left(-4156+2021\right)-\left(119+2021-4156\right)\)
\(=-4156+2021-119-2021+4156\)
\(=\left(-4156+4156\right)+\left(2021-2021\right)-119\)
=0+0-119
=-119
g: \(315\cdot75-\left(15\cdot100-315\cdot25\right)\)
\(=315\cdot75-15\cdot100+315\cdot25\)
\(=315\left(75+25\right)-15\cdot100\)
\(=315\cdot100-15\cdot100=300\cdot100=30000\)
h: \(\left(-489\right)\cdot125-\left(125\cdot11-500\cdot25\right)\)
\(=-489\cdot125-125\cdot11+500\cdot25\)
\(=125\left(-489-11\right)+500\cdot25\)
\(=125\cdot\left(-500\right)+500\cdot25\)
\(=500\left(-125+25\right)\)
\(=500\cdot\left(-100\right)=-50000\)
Bài 2:
a: \(-415-3\left(2x-1\right)^2=-490\)
=>\(3\left(2x-1\right)^2+415=490\)
=>\(3\left(2x-1\right)^2=75\)
=>\(\left(2x-1\right)^2=25\)
=>\(\left[{}\begin{matrix}2x-1=5\\2x-1=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=6\\2x=-4\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=3\\x=-2\end{matrix}\right.\)

Tôi đây
Ko biết làm bt đó
# USAS - 12 #
\(=\frac{5}{3}.\frac{7}{5}.\frac{9}{7}......\frac{91}{89}\)
\(=\frac{91}{3}\)
@@@@@@@@@@@2

Ta có: \(A=3+3^2+3^3+...+3^{2008}\)
\(\Rightarrow3A=3^2+3^3+3^4+...+3^{2009}\)
Trừ \(3A-A=3^2+3^3+3^4+...+3^{2009}-3-3^2-3^3-...-3^{2008}\)
\(\Rightarrow2A=3^{2009}-3\)
Mà \(2A=3^x-3\)
\(\Rightarrow3^x=3^{2009}\)
\(\Rightarrow x=2009.\)
Vậy x = 2009.
\(a=3+3^2+3^3+...+3^{2008}\)
\(3a=3^2+3^3+3^4+...+3^{2009}\)
\(3a-a=\left(3^2+3^3+3^4+...+3^{2009}\right)-\left(3+3^2+3^3+...+3^{2008}\right)\)
\(2a=3^{2009}-3\)
\(2a+3=3^{2009}=3^x\)
\(x=2009\)


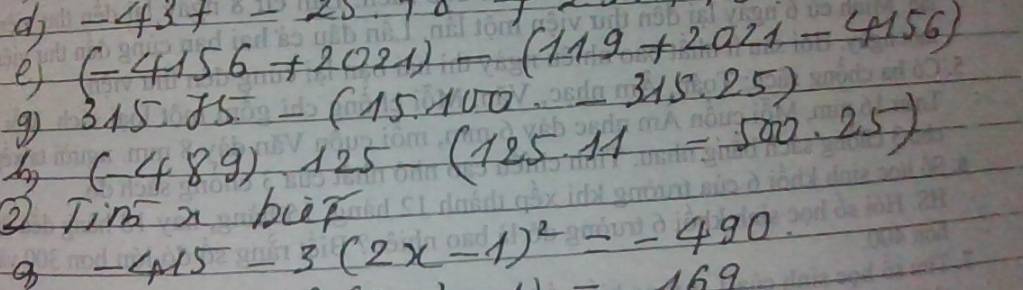
Bài 3:
Vì (d') và (d'') cắt nhau tại một điểm trên trục tung nên ta có:
\(\left\{{}\begin{matrix}m+2< >2\\-m^2+4m-2=m-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< >0\\-m^2+3m=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< >0\\m\left(-m+3\right)=0\end{matrix}\right.\Leftrightarrow m=3\)