Cho biểu thức: A = \(\dfrac{\sqrt{4x^2-4x+1}}{4x-2}\). Chứng tỏ | A | = 0,5 với x \(\ne\) 0,5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TN
1
Y
14 tháng 5 2019
a) A \(=\frac{x^2-4}{2}\cdot\sqrt{\frac{2^2}{\left(x-2\right)^2}}\) \(=\frac{x^2-4}{2}\cdot\left|\frac{2}{x-2}\right|\)
+ Với x < 2 ta có \(A=\frac{x^2-4}{2}\cdot\frac{2}{2-x}\)
\(A=\frac{\left(x+2\right)\left(x-2\right)}{2-x}=-\left(x+2\right)\)
+ Với x > 2 ta có : \(A=\frac{x^2-4}{2}\cdot\frac{2}{x-2}\)
\(A=\frac{\left(x-2\right)\left(x+2\right)}{x-2}=x+2\)
câu b và c tương tự
D
31 tháng 7 2017
A = \(\dfrac{\sqrt{4x^2-4x+1}}{4x-2}\)
A = \(\dfrac{\sqrt{\left(2x-1\right)^2}}{2\left(2x-1\right)}\)
A = \(\dfrac{\left|2x-1\right|}{2\left(2x-1\right)}\)
\(\left|A\right|=\dfrac{2x-1}{2\left(2x-1\right)}\) \(\Rightarrow\left|A\right|=\dfrac{1}{2}=0,5\left(x\ne0,5\right)\)
TN
0
20 tháng 2 2021
a) Ta có: \(M=\left(\dfrac{\sqrt{x}-3}{\sqrt{x}-2}-\dfrac{\sqrt{x}+1}{\sqrt{x}+3}\right)\cdot\dfrac{x+3\sqrt{x}}{7-\sqrt{x}}\)
\(=\left(\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}-\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}\right)\cdot\dfrac{x+3\sqrt{x}}{7-\sqrt{x}}\)
\(=\dfrac{x-9-\left(x-2\sqrt{x}+\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{-\left(\sqrt{x}-7\right)}\)
\(=\dfrac{x-9-x+\sqrt{x}+2}{\sqrt{x}-2}\cdot\dfrac{-\sqrt{x}}{\sqrt{x}-7}\)
\(=\dfrac{\sqrt{x}-7}{\sqrt{x}-2}\cdot\dfrac{-\sqrt{x}}{\sqrt{x}-7}\)
\(=\dfrac{-\sqrt{x}}{\sqrt{x}-2}\)
b) Ta có: \(x^2-4x=0\)
\(\Leftrightarrow x\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(nhận\right)\\x=4\left(loại\right)\end{matrix}\right.\)
Thay x=0 vào biểu thức \(M=\dfrac{-\sqrt{x}}{\sqrt{x}-2}\), ta được:
\(M=\dfrac{-\sqrt{0}}{\sqrt{0}-2}=-\dfrac{0}{-2}=0\)
Vậy: Khi \(x^2-4x=0\) thì M=0
5 tháng 7 2021
a) Pt \(\Leftrightarrow\sqrt{\left(x-2\right)^2}=5\Leftrightarrow\left|x-2\right|=5\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=5\\x-2=-5\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=7\\x=-3\end{matrix}\right.\)
Vậy...
b)Đk: \(x\ge-1\)
Pt \(\Leftrightarrow4\sqrt{x+1}-3\sqrt{x+1}+2\sqrt{x+1}=16-\sqrt{x+1}\)
\(\Leftrightarrow4\sqrt{x+1}=16\)\(\Leftrightarrow x+1=16\)\(\Leftrightarrow x=15\) (tm)
Vậy...
\(A=\dfrac{a^2+\sqrt{a}}{a-\sqrt{a}+1}-\dfrac{2a+\sqrt{a}}{\sqrt{a}}+1\) (a>0)
\(=\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-\dfrac{\sqrt{a}\left(2\sqrt{a}+1\right)}{\sqrt{a}}+1\)
\(=a+\sqrt{a}-\left(2\sqrt{a}+1\right)+1=a-\sqrt{a}\)
b) \(A=a-\sqrt{a}=a-2.\dfrac{1}{2}\sqrt{a}+\dfrac{1}{4}-\dfrac{1}{4}=\left(\sqrt{a}-\dfrac{1}{2}\right)^2-\dfrac{1}{4}\ge-\dfrac{1}{4}\)
Dấu "=" xảy ra khi \(\sqrt{a}=\dfrac{1}{2}\Leftrightarrow a=\dfrac{1}{4}\left(tmđk\right)\)
Vậy \(A_{min}=-\dfrac{1}{4}\)
AT
5 tháng 7 2021
a) \(\sqrt{x^2-4x+4}=5\Rightarrow\sqrt{\left(x-2\right)^2}=5\Rightarrow\left|x-2\right|=5\)
\(\Rightarrow\left[{}\begin{matrix}x-2=5\\x-2=-5\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=7\\x=-3\end{matrix}\right.\)
b) \(\sqrt{16x+16}-3\sqrt{x+1}+\sqrt{4x+4}=16-\sqrt{x+1}\)
\(\Rightarrow\sqrt{16\left(x+1\right)}-3\sqrt{x+1}+\sqrt{4\left(x+1\right)}+\sqrt{x+1}=16\)
\(\Rightarrow4\sqrt{x+1}-3\sqrt{x+1}+2\sqrt{x+1}+\sqrt{x+1}=16\)
\(\Rightarrow4\sqrt{x+1}=16\Rightarrow\sqrt{x+1}=4\Rightarrow x=15\)
a) \(A=\dfrac{a^2+\sqrt{a}}{a-\sqrt{a}+1}-\dfrac{2a+\sqrt{a}}{\sqrt{a}}+1\)
\(=\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)\left(a-\sqrt{a}+1\right)}{a-\sqrt{a}+1}-\dfrac{\sqrt{a}\left(2\sqrt{a}+1\right)}{\sqrt{a}}+1\)
\(=a+\sqrt{a}-2\sqrt{a}-1+1=a-\sqrt{a}\)
b) Ta có: \(a-\sqrt{a}=\left(\sqrt{a}\right)^2-2.\sqrt{a}.\dfrac{1}{2}+\left(\dfrac{1}{2}\right)^2-\dfrac{1}{4}\)
\(=\left(\sqrt{a}-\dfrac{1}{2}\right)^2-\dfrac{1}{4}\ge-\dfrac{1}{4}\)
\(\Rightarrow A_{min}=-\dfrac{1}{4}\) khi \(a=\dfrac{1}{4}\)
PT
1
22 tháng 5 2022
a: \(=4a-4\sqrt{10a}-9\sqrt{10a}=4a-13\sqrt{10a}\)
b: \(=\sqrt{x}\left(4-\sqrt{2}\right)\cdot\sqrt{x}\left(1-\sqrt{2}\right)\)
\(=x\cdot\left(4-4\sqrt{2}-\sqrt{2}+2\right)\)
\(=\left(6-5\sqrt{2}\right)x\)
c: \(=\dfrac{2}{2x-1}\cdot x\sqrt{5}\cdot\left(2x-1\right)=2x\sqrt{5}\)
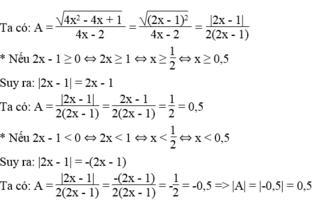
\(A=\dfrac{\sqrt{4x^2-4x+1}}{4x-2}=\dfrac{\sqrt{\left(2x-1\right)^2}}{2\left(2x-1\right)}=\dfrac{\left|2x-1\right|}{2\left(2x-1\right)}\)
\(\Rightarrow\left|A\right|=\left|\dfrac{\left|2x-1\right|}{2\left(2x-1\right)}\right|=\dfrac{\left|2x-1\right|}{2\left|2x-1\right|}=\dfrac{1}{2}\)
Ta có: \(A=\dfrac{\sqrt{4x^2-4x+1}}{4x-2}\)
\(=\dfrac{\left|2x-1\right|}{2\left(2x-1\right)}\)
\(=\left[{}\begin{matrix}-\dfrac{\left(2x-1\right)}{2\left(2x-1\right)}=-\dfrac{1}{2}\left(x< \dfrac{1}{2}\right)\\\dfrac{2x-1}{2\left(2x-1\right)}=\dfrac{1}{2}\left(x\ge\dfrac{1}{2}\right)\end{matrix}\right.\)
\(\Leftrightarrow\left|A\right|=0.5\)