Sửa đề : \(\dfrac{a^2}{a^2+b}+\dfrac{b^2}{b^2+a}\le1\\ \) (*)

\(< =>\dfrac{a^2\left(b^2+a\right)+b^2\left(a^2+b\right)}{\left(a^2+b\right)\left(b^2+a\right)}\le1\\ < =>a^2b^2+a^3+b^2a^2+b^3\le\left(a^2+b\right)\left(b^2+a\right)\) ( Nhân cả 2 vế cho `(a^{2}+b)(b^{2}+a)>0` )

\(< =>a^3+b^3+2a^2b^2\le a^2b^2+b^3+a^3+ab\\ < =>a^2b^2\le ab\\ < =>ab\le1\) ( Chia 2 vế cho `ab>0` )

Do a,b >0 

Nên áp dụng BDT Cô Si :

\(2\ge a+b\ge2\sqrt{ab}< =>\sqrt{ab}\le1\\ < =>ab\le1\)

Do đó (*) luôn đúng

Vậy ta chứng minh đc bài toán

Dấu "=" xảy ra khi : \(a=b>0,a+b=2< =>a=b=1\)

a Sửa đề : Chứng minh \(\dfrac{a^2}{a^2+b}\)+\(\dfrac{b^2}{b^2+a}\)\(\le\) 1 ( Đề thi vào 10 Hà Nội).

Bất đẳng thức trên tương đương : 

\(\dfrac{a^2+b-b}{a^2+b}\)+\(\dfrac{b^2+a-a}{b^2+a}\)\(\le\)1

\(\Leftrightarrow\) 1 - \(\dfrac{b}{a^2+b}\)+ 1 - \(\dfrac{a}{b^2+a}\)\(\le\)1

\(\Leftrightarrow\)1 - \(\dfrac{b}{a^2+b}\) - \(\dfrac{a}{b^2+a}\)\(\le\)0

\(\Leftrightarrow\)- \(\dfrac{b}{a^2+b}\)- \(\dfrac{a}{b^2+a}\)\(\le\)-1

\(\Leftrightarrow\)\(\dfrac{a}{b^2+a}\)+ \(\dfrac{b}{a^2+b}\)\(\ge\)1

Xét VT = \(\dfrac{a^2}{ab^2+a^2}\)+ \(\dfrac{b^2}{a^2b+b^2}\)\(\ge\)\(\dfrac{\left(a+b\right)^2}{ab^2+a^2+a^2b+b^2}\) (Cauchy - Schwarz)

= \(\dfrac{\left(a+b\right)^2}{ab\left(b+a\right)+a^2+b^2}\)

\(\ge\)\(\dfrac{\left(a+b\right)^2}{2ab+a^2+b^2}\)

= \(\dfrac{\left(a+b\right)^2}{\left(a+b\right)^2}\)= 1

Vậy BĐT được chứng minh

Dấu '=' xảy ra \(\Leftrightarrow\)a = b = 1

đkxđ: \(\left\{{}\begin{matrix}x\ne0\\y\ne0\end{matrix}\right.\)

pt đầu \(\Leftrightarrow x+\dfrac{2}{x}+y+\dfrac{1}{y}=6\)            (3)

pt thứ 2 \(\Leftrightarrow x^2+\dfrac{4}{x^2}+y^2+\dfrac{1}{y^2}=14\) \(\Leftrightarrow\left(x^2+2.x.\dfrac{2}{x}+\dfrac{4}{x^2}\right)+\left(y^2+2y.\dfrac{1}{y}+\dfrac{1}{y^2}\right)=20\)

\(\Leftrightarrow\left(x+\dfrac{2}{x}\right)^2+\left(y+\dfrac{1}{y}\right)^2=20\)                   (4)

Đặt \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=u\left(\left|u\right|\ge2\sqrt{2}\right)\\y+\dfrac{1}{y}=v\left(\left|v\right|\ge2\right)\end{matrix}\right.\) thì từ (3) và (4) suy ra \(\left\{{}\begin{matrix}u+v=6\\u^2+v^2=20\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}v=6-u\\u^2+\left(6-u\right)^2=20\end{matrix}\right.\) 

\(u^2+\left(6-u\right)^2=20\) \(\Leftrightarrow u^2+36-12u+u^2=20\) \(\Leftrightarrow2u^2-12u+16=0\) \(\Leftrightarrow u^2-6u+8=0\) \(\Leftrightarrow\left(u-2\right)\left(u-4\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}u=2\left(loại\right)\\u=4\left(nhận\right)\end{matrix}\right.\). 

\(\Rightarrow v=6-u=2\), suy ra \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=4\\y+\dfrac{1}{y}=2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x=2\pm\sqrt{2}\\y=1\end{matrix}\right.\) (nhận).

 Vậy hpt đã cho có các nghiệm \(\left(x;y\right)\in\left\{\left(2-\sqrt{2};1\right);\left(2+\sqrt{2};1\right)\right\}\)

\(A=\dfrac{\cos^217^o+2\cos^273^o}{\cot65^o\cot25^o}-\sin^217^o\)

\(A=\dfrac{\left(\cos^217^o+\cos^273^o\right)+\cos^273^o}{\tan25^o\cot25^o}-\sin^217^o\) 

(áp dụng công thức \(\cot\alpha=\tan\left(90^o-\alpha\right)\))

\(A=\left(\cos^217^o+\sin^217^o\right)+\sin^217^o-\sin^217^o\) 

(áp dụng công thức \(\tan\alpha.\cot\alpha=1\) và \(\cos\alpha=\sin\left(90^o-\alpha\right)\))

\(A=1\) 

 Điều cần chứng minh của bạn mới có 1 vế thôi nhé. Mình chưa thấy vế kia đâu thì không thể giúp bạn được.

Lượng phần trăm mất đi hay còn lại em ơi?

Luong phan tram mất di á cô

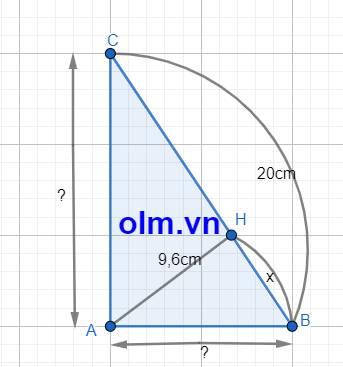
Gọi độ dài đoạn BH là: \(x\) ( cm) ; \(x\) > 0; AC > AB nên  \(x\) < CH

Xét tam giác vuông HAB vuông tại H theo pytago ta có:

AB² = HA² + HB² = 9,6² + \(x^2\) = 92,16 + \(x^2\)

Xét tam giác vuông AHC vuông tại H theo pytago ta có:

AC² = HA² + HC² = 9,6² + (\(20-x\))² = 92,16 + 400 - 40\(x\) + \(x^2\) 

AC² = 492,16 - 40\(x\) + \(x^2\)

Xét tam giác vuông ABC vuông tại A theo pytago ta có:

AC² + AB² = BC²

492,16  - 40\(x\) + \(x^2\) + 92,16 + \(x^2\) = 20²

(\(x^2\) + \(x^2\)) - 40\(x\) + (492,16 + 92,16) - 400 = 0

2\(x^2\) - 40\(x\) + 584,32 - 400 = 0

2\(x^2\)- 40\(x\) + 184,32 =0

\(x^2\) - 20\(x\) + 92,16 = 0

△' = 10² - 92,16 = 7,84 > 0

\(x\)₁ =  -(-10) + \(\sqrt{7,84}\) =  12,8 ⇒ CH = 20 - 12,8 = 7,2 < BH  (loại )

\(x_2\) = -(-10) - \(\sqrt{7,84}\) = 7,2 ⇒ CH = 20 - 7,2 = 12,8 (thỏa mãn)

Thay \(x_2\) = 7,2 vào biểu thức: AB² = 92,16 + \(x^2\) = 92,16 + 7,2² = 144 

⇒AB = \(\sqrt{144}\) = 12 

Thay \(x_2\) = 7,2 vào biểu thức: AC² = 492,16 - 40\(x\) + \(x^2\) 

AC² = 492,16 - 40\(\times\) 7,2 + 7,2² = 256

AC = \(\sqrt{256}\) = 16

Kết luận AB = 12 cm; AC = 16 cm 

\(\dfrac{5}{\sqrt{7}}+\sqrt{2}+\dfrac{1}{\sqrt{2}}-1-7+\dfrac{\sqrt{3}}{\sqrt{7}}+1\)

\(=\dfrac{5\sqrt{7}}{7}+\dfrac{3\sqrt{2}}{2}-7+\dfrac{\sqrt{21}}{7}\)

\(=\dfrac{5\sqrt{7}+\sqrt{21}-49}{7}+\dfrac{3\sqrt{2}}{2}\)

\(\dfrac{1}{2\sqrt{3}-3\sqrt{2}}+\dfrac{1}{2\sqrt{3}+3\sqrt{2}}\)

\(=\dfrac{2\sqrt{3}+3\sqrt{2}+2\sqrt{3}-3\sqrt{2}}{\left(2\sqrt{3}-3\sqrt{2}\right)\left(2\sqrt{3}+3\sqrt{2}\right)}\)

\(=\dfrac{\left(2\sqrt{3}+2\sqrt{3}\right)+\left(3\sqrt{2}-3\sqrt{2}\right)}{\left(2\sqrt{3}\right)^2-\left(3\sqrt{2}\right)^2}\)

\(=\dfrac{4\sqrt{3}+0}{4.3-9.2}\)

\(=\dfrac{4\sqrt{3}}{12-18}\)

\(=\dfrac{4\sqrt{3}}{-6}\)

\(=-\dfrac{2\sqrt{3}}{3}\)