
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) x = -1. b) x = 4 hoặc x = 5.
c) x = ± 2 . d) x = 1 hoặc x = 2.

Bài 1
a/ \(x\left(x^2+1\right)+2\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x^2+1\right)=0\Rightarrow x=-2\)
b/
\(\Leftrightarrow x^3-6x^2+9x+5x^2-30x+45=0\)
\(\Leftrightarrow x\left(x-3\right)^2+5\left(x-3\right)^2=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-3\right)^2=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-5\\x=3\end{matrix}\right.\)
1.
c/ \(\Leftrightarrow x^3+2x^2+2x+x^2+2x+2=0\)
\(\Leftrightarrow x\left(x^2+2x+2\right)+x^2+2x+2=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^2+2x+2=0\left(vn\right)\end{matrix}\right.\)
d/
\(\Leftrightarrow x^4+x^3-2x^2-x^3-x^2+2x+4x^2+4x-8=0\)
\(\Leftrightarrow x^2\left(x^2+x-2\right)-x\left(x^2+x-2\right)+4\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left(x^2-x+4\right)\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-x+4=0\left(vn\right)\\x^2+x-2=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)

Đáp án D.
- Cách 1:
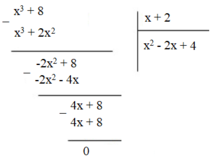
- Cách 2: sử dụng hằng đẳng thức
Ta có:
x 3 + 8 = x 3 + 2 3 x + 2 x 2 - 2 x + 4 ⇒ x 3 + 8 : x + 2 = x 2 - 2 x + 4
⇒ Chọn D

\(a,\Leftrightarrow x^3-8-x^3-2x=12\Leftrightarrow-2x=20\Leftrightarrow x=-10\\ b,\Leftrightarrow x^2-6x+9-x^2+4=16\Leftrightarrow=-6x=3\Leftrightarrow x=-\dfrac{1}{2}\\ c,\Leftrightarrow x\left(x^2-9\right)=0\\ \Leftrightarrow x\left(x-3\right)\left(x+3\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\\ d,\Leftrightarrow x^2\left(x-6\right)+9\left(x-6\right)=0\\ \Leftrightarrow\left(x^2+9\right)\left(x-6\right)=0\\ \Leftrightarrow x=6\left(x^2+9>0\right)\)

a: \(\Leftrightarrow\left(x-3\right)\left(5x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=\dfrac{1}{5}\end{matrix}\right.\)
b: \(\Leftrightarrow x\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=-1\end{matrix}\right.\)
 Bài 1.
Bài 1.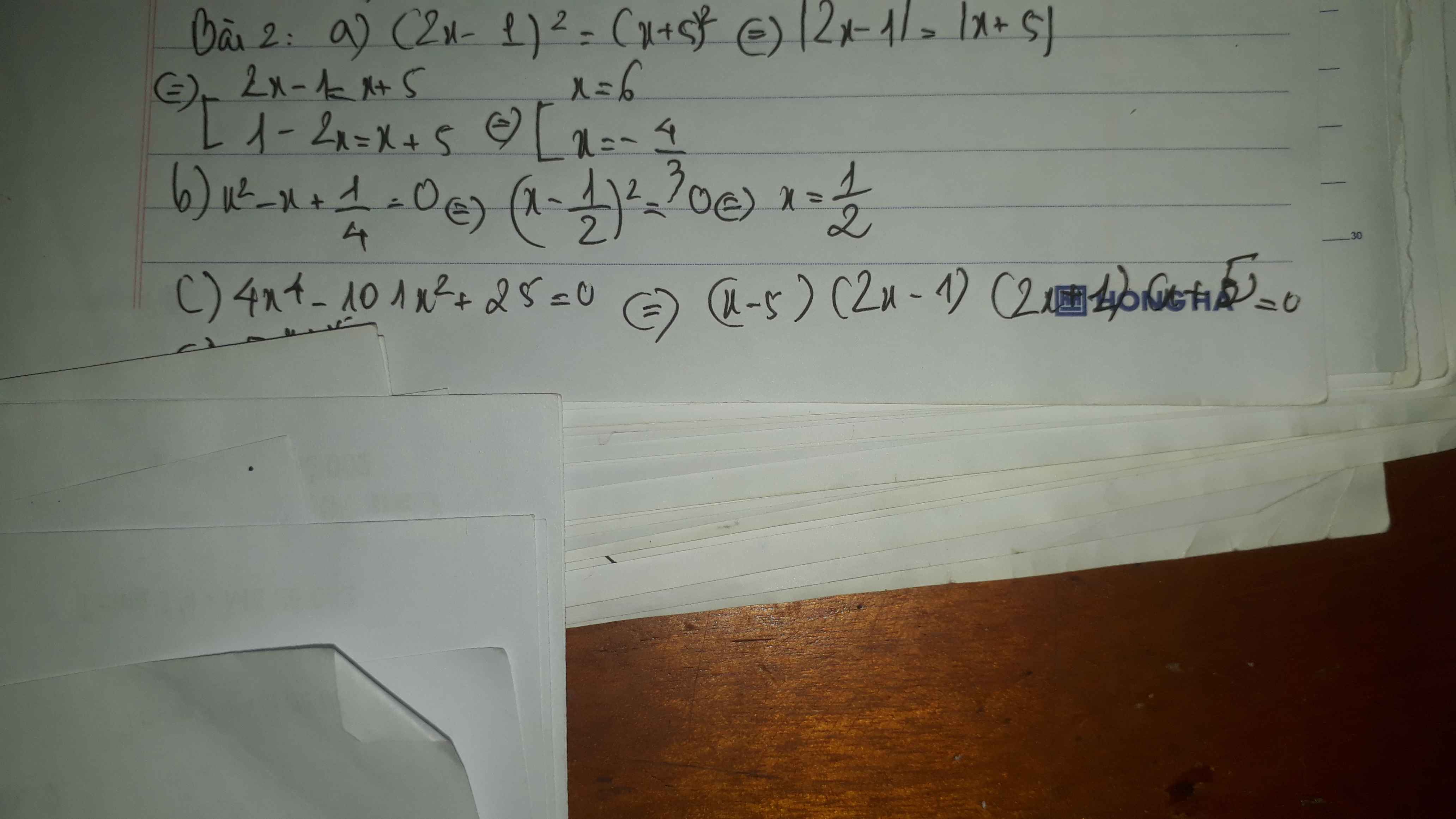
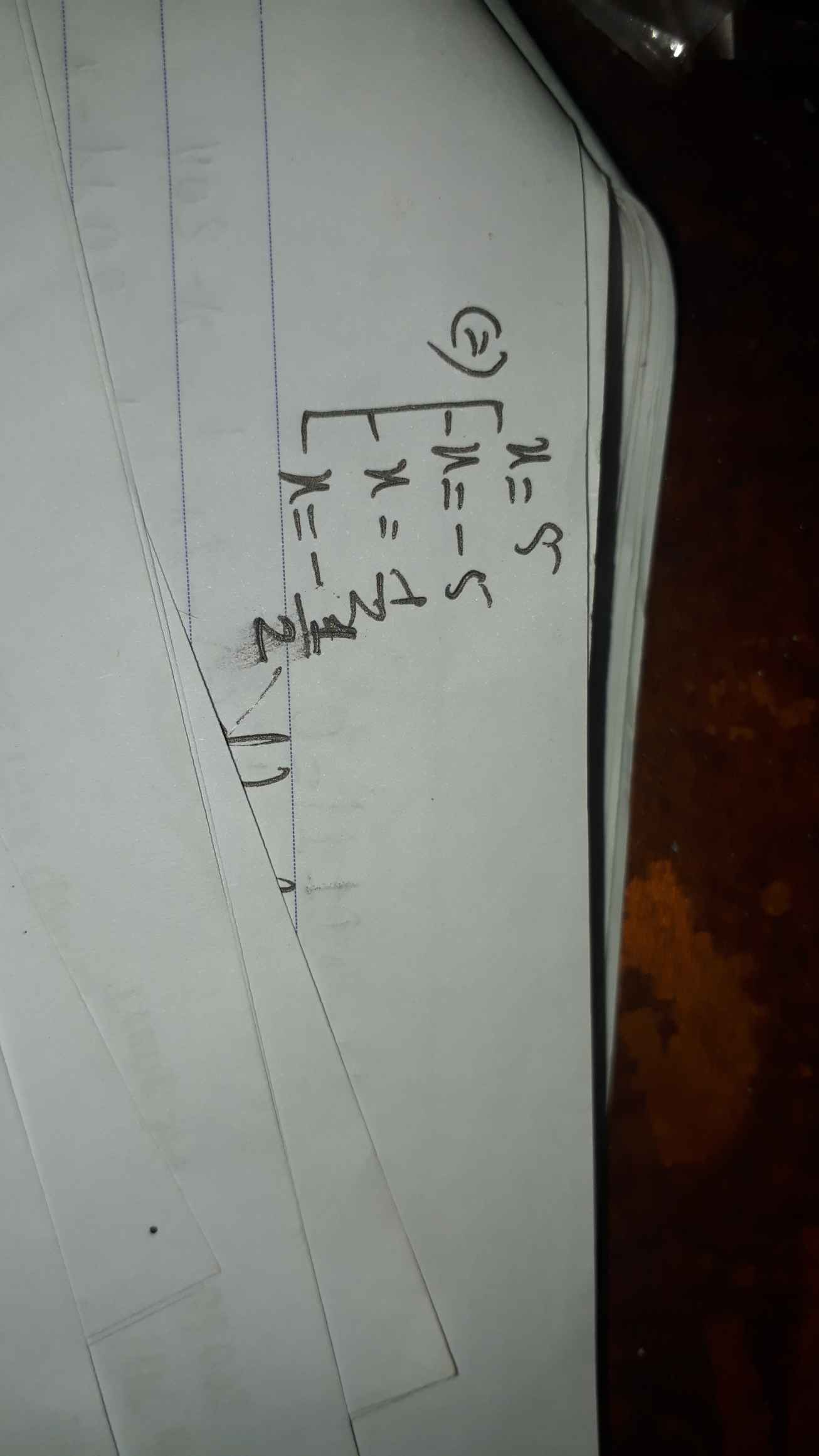

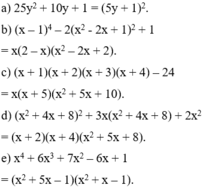
a) x3 - 8 = ( x - 2 )( x - 12 )
<=> ( x - 2 )( x2 + 2x + 4 ) - ( x - 2 )( x - 12 ) = 0
<=> ( x - 2 )( x2 + 2x + 4 - x + 12 ) = 0
<=> ( x - 2 )( x2 + x + 16 ) = 0
<=> \(\orbr{\begin{cases}x-2=0\\x^2+x+16=0\end{cases}}\Leftrightarrow x=2\)( vì x2 + x + 16 = ( x2 + x + 1/4 ) + 63/4 = ( x + 1/2 )2 + 63/4 ≥ 63/4 > 0 ∀ x )
b) x2( x2 + 4 ) - x2 = 4
<=> x2( x2 + 4 ) - x2 - 4 = 0
<=> x2( x2 + 4 ) - ( x2 + 4 ) = 0
<=> ( x2 + 4 )( x2 - 1 ) = 0
<=> \(\orbr{\begin{cases}x^2+4=0\\x^2-1=0\end{cases}}\Leftrightarrow x=\pm1\)( vì x2 + 4 ≥ 4 > 0 ∀ x )
Ko khó nè :3, đừng tách ra nhé !
a, \(x^3-8=\left(x-2\right)\left(x-12\right)\)
\(\Leftrightarrow\left(x-2\right)\left(x^2+2x+4\right)=\left(x-2\right)\left(x-12\right)\)
\(\Leftrightarrow\left(x-2\right)\left(x^2+2x+4-x+12\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x^2+x+16\right)=0\Leftrightarrow x=2\)
b, \(x^2\left(x^2+4\right)-x^2=4\Leftrightarrow-x^2\left(-x^2-4\right)-x^2=4\)
\(\Leftrightarrow-x^2\left(4-x^2\right)-x^2=4\Leftrightarrow-x^2\left(2-x\right)\left(2+x\right)-x^2-4=0\)
\(\Leftrightarrow-x^2\left(2-x\right)\left(2+x\right)+\left(-x^2-4\right)=0\)
\(\Leftrightarrow-x^2\left(2-x\right)\left(2+x\right)+\left(2-x\right)\left(2+x\right)=0\)
\(\Leftrightarrow\left(-x^2+1\right)\left(2-x\right)\left(2+x\right)=0\Leftrightarrow x=\pm1;\pm2\)
Check hộ dáp án nhá :), ko chắc lắm nếu khai triển sẽ dễ nhìn hơn đấy.