K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
5 tháng 1 2022
a: \(M=\dfrac{18+5x+15+3x-9}{\left(x+3\right)\left(x-3\right)}=\dfrac{8x+24}{\left(x+3\right)\left(x-3\right)}=\dfrac{8}{x-3}\)
b: Thay x=11 vào M, ta được:
\(M=\dfrac{8}{11-3}=1\)
5 tháng 1 2022
a) \(M=\dfrac{18}{x^2-9}+\dfrac{5}{x-3}+\dfrac{3}{x+3}.\left(x\ne\pm3\right).\)
\(M=\dfrac{18}{\left(x-3\right)\left(x+3\right)}+\dfrac{5}{x-3}+\dfrac{3}{x+3}=\dfrac{18+5\left(x+3\right)+3\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{18+5x+15+3x-9}{\left(x-3\right)\left(x+3\right)}=\dfrac{24+8x}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{8\left(3+x\right)}{\left(x-3\right)\left(x+3\right)}=\dfrac{8}{x-3}.\)
b) Thay \(x=11\left(TM\right)\) vào biểu thức M:
\(\dfrac{8}{11-3}=\dfrac{8}{8}=1.\)
TT
3
2
23 tháng 5 2022
Với `x \ne +-2` có:
`M=[x^3]/[x^2-4]-x/[x-2]-2/[x+2]`
`M=[x^3-x(x+2)-2(x-2)]/[(x-2)(x+2)]`
`M=[x^3-x^2-2x-2x+4]/[(x-2)(x+2)]`
`M=[x^3-x^2-4x+4]/[(x-2)(x+2)]`
`M=[x^2(x-1)-4(x-1)]/[x^2-4]`
`M=[(x-1)(x^2-4)]/[x^2-4]`
`M=x-1`
23 tháng 5 2022
\(M=\dfrac{x^3}{x^2-4}-\dfrac{x}{x-2}-\dfrac{2}{x+2}\)
\(=\dfrac{x^3-x\left(x+2\right)-2\left(x-2\right)}{x^2-4}\)
\(=\dfrac{x^3-x^2-2x-2x+4}{x^2+4}=\dfrac{x^3-4x-x^2+4}{x^2-4}=\dfrac{x\left(x^2-4\right)-\left(x^2-4\right)}{x^2-4}\)
\(=\dfrac{\left(x^2-4\right)\left(x-1\right)}{x^2-4}=x-1\)
CM
8 tháng 6 2017
a) M có nghĩa khi a 3 - 4 a ≠ 0 ⇔ a ≠ { 0 ; ± 2 }
b) Rút gọn thu được: M = a ( a 2 + 4 a + 4 ) a ( a 2 − 4 ) = a + 2 a − 2
c) M = − 3 ⇔ a + 2 a − 2 = − 3 ⇔ a = 1 (TMĐK)
28 tháng 9 2023
`#3107.\text {DN}`
a)
\((2x-3)^2-x(3-x)+5x-4x^2+17\)
`= 4x^2 - 12x + 9 - 3x + x^2 + 5x - 4x^2 + 17`
`= x^2 - 10x + 26`
b)
`M = x^2 - 10x + 26`
`= [(x)^2 - 2*x*5 + 5^2] + 1`
`= (x - 5)^2 + 1`
Vì `(x - 5)^2 \ge 0` `AA` `x => (x - 5)^2 + 1 \ge 1` `AA` `x`
Vậy, giá trị biểu thức M luôn có giá trị dương với mọi x.
13 tháng 6 2018
a, \(M=\sqrt{x^2-4x+4}-\sqrt{x^2+4x+4}\) (ĐK : \(\forall x\in R\))
\(=\sqrt{\left(x-2\right)^2}-\sqrt{\left(x+2\right)^2}\)
* Nếu x\(\ge2\Rightarrow M=x-2-x-2=-4\)
*Nếu x<2 => M=2-x-x-2=-2x
b,Để M=2\(\ne-4\)
=>M=-2x
=>-2x=-4
=>x=2
__________________________________________________________________________________________
P=\(\sqrt{x+2\sqrt{x-1}}+\sqrt{x-2\sqrt{x-1}}\)
\(=\sqrt{x-1+2\sqrt{x-1}+1}+\sqrt{x-1-2\sqrt{x-1}+1}\)
\(=\sqrt{\left(\sqrt{x-1}+1\right)^2}+\sqrt{\left(\sqrt{x-1}-1\right)^2}\)
* Nếu \(x\ge2\Rightarrow P=\sqrt{x-1}+1+\sqrt{x-1}-1=2\sqrt{x-1}\)
* Nếu x<2 =>P=\(\sqrt{x-1}+1+1-\sqrt{x-1}=2\)
VẬY.......
Tk nha!
27 tháng 5 2016
KHI X<=2 => X ÂM
KHI ĐÓ TRỊ TUYỆT ĐỐI CỦA X-2=2-x
m=3X+2-X=2X+2
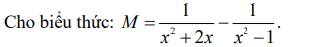
ĐKXĐ: \(x\notin\left\{0;-2;1;-1\right\}\)
\(M=\dfrac{1}{x^2+2x}-\dfrac{1}{x^2-1}\)
\(=\dfrac{x^2-1-x^2-2x}{\left(x^2-1\right)\left(x^2+2x\right)}\)
\(=\dfrac{-2x-1}{\left(x-1\right)\left(x+1\right)\cdot x\left(x+2\right)}\)