K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
11 tháng 3 2022
Bài 2
a, bạn tự vẽ
b, Hoành độ giao điểm tm pt
\(2x^2-2x+3=0\)
\(\Delta'=1-3.2=-5< 0\)
Vậy pt vô nghiệm hay (d) ko cắt (P)
A
10 tháng 10
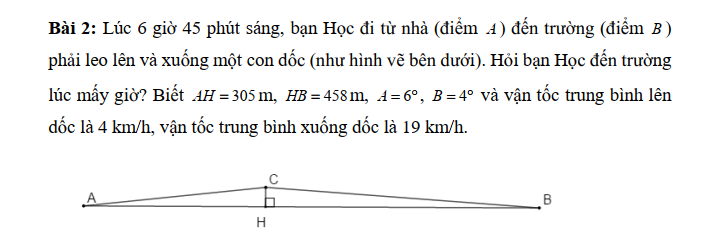
Độ dài ACACAC được tính từ góc A=6∘A = 6^\circA=6∘ và cạnh đối AH=305 mAH = 305 \, mAH=305m.
AC=AHsinA=305sin6∘AC = \frac{AH}{\sin A} = \frac{305}{\sin 6^\circ}AC=sinAAH=sin6∘305Độ dài CBCBCB được tính từ góc B=4∘B = 4^\circB=4∘ và cạnh đối HB=458 mHB = 458 \, mHB=458m.
CB=HBsinB=458sin4∘CB = \frac{HB}{\sin B} = \frac{458}{\sin 4^\circ}CB=sinBHB=sin4∘458Thời gian leo dốc từ AAA đến CCC:
tAC=AC4 km/ht_{AC} = \frac{AC}{4 \, km/h}tAC=4km/hACThời gian xuống dốc từ CCC đến BBB:
tCB=CB19 km/ht_{CB} = \frac{CB}{19 \, km/h}tCB=19km/hCBTổng thời gian di chuyển: ttotal=tAC+tCBt_{\text{total}} = t_{AC} + t_{CB}ttotal=tAC+tCBThời gian bạn Học đến trường bằng cách cộng tổng thời gian này vào thời gian khởi hành 6 giờ 45 phút.
Y
6 tháng 7 2023
\(VT=\sqrt{\dfrac{\sqrt{5}}{8\sqrt{5}+3\sqrt{35}}}.\left(3\sqrt{2}+\sqrt{14}\right)\)
\(=\sqrt{\dfrac{\sqrt{5}}{8\sqrt{5}+3\sqrt{5}.\sqrt{7}}}.\left(3\sqrt{2}+\sqrt{2}.\sqrt{7}\right)\)
\(=\sqrt{\dfrac{\sqrt{5}}{\sqrt{5}\left(8+3\sqrt{7}\right)}}.\left[\sqrt{2}\left(3+\sqrt{7}\right)\right]\)
\(=\sqrt{\dfrac{1}{8+3\sqrt{7}}}.\left[\sqrt{2}\left(3+\sqrt{7}\right)\right]\)
\(=\dfrac{\sqrt{2}\left(3+\sqrt{7}\right)}{\sqrt{8+3\sqrt{7}}}\)
\(=\dfrac{\sqrt{2}.\sqrt{2}\left(3+\sqrt{7}\right)}{\sqrt{2}.\sqrt{8+3\sqrt{7}}}\) (Nhân \(\sqrt{2}\) cả tử và mẫu)
\(=\dfrac{2\left(3+\sqrt{7}\right)}{\sqrt{16+6\sqrt{7}}}\)
\(=\dfrac{2\left(3+\sqrt{7}\right)}{\sqrt{\left(3+\sqrt{7}\right)^2}}\)
\(=\dfrac{2\left(3+\sqrt{7}\right)}{\left|3+\sqrt{7}\right|}\)
\(=\dfrac{2\left(3+\sqrt{7}\right)}{3+\sqrt{7}}\)
\(=2=VP\left(dpcm\right)\)
AH
Akai Haruma
Giáo viên
5 tháng 1
Lời giải:
ĐKXĐ: $x\geq 0; x\neq 9$
\(C=\frac{\sqrt{x}(\sqrt{x}+3)-(\sqrt{x}-3)}{(\sqrt{x}-3)(\sqrt{x}+3)}.\frac{\sqrt{x}-3}{x+3}\\ =\frac{x+2\sqrt{x}+3}{(\sqrt{x}-3)(\sqrt{x}+3)}.\frac{\sqrt{x}-3}{x+3}=\frac{x+2\sqrt{x}+3}{(\sqrt{x}+3)(x+3)}\)
27 tháng 2 2023
1: Khi x=3-2 căn 2 thì \(A=\dfrac{\sqrt{2}-1+2}{\sqrt{2}-1}=\dfrac{\sqrt{2}+1}{\sqrt{2}-1}=3+2\sqrt{2}\)
2: \(B=\dfrac{x+\sqrt{x}+2+\sqrt{x}-2}{x-4}=\dfrac{x+2\sqrt{x}}{x-4}=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
3: \(P=A:B=\dfrac{\sqrt{x}+2}{\sqrt{x}}:\dfrac{\sqrt{x}}{\sqrt{x}-2}=\dfrac{x-4}{x}\)
\(x\cdot P< =10\sqrt{x}-29-\sqrt{x-25}\)
=>\(x-4< =10\sqrt{x}-29-\sqrt{x-25}\)
\(\Leftrightarrow x-4-10\sqrt{x}+29< =-\sqrt{x-25}\)
=>\(x-10\sqrt{x}+25< =-\sqrt{x-25}\)
=>(căn x-5)^2<=-căn x-25
=>x-25=0
=>x=25
29 tháng 10 2021
2: Để (d)//y=(m2+1)x-4 thì \(\left\{{}\begin{matrix}m^2=1\\m-5\ne-4\end{matrix}\right.\Leftrightarrow m=1\)
6 tháng 7 2023
a: ĐKXĐ: a>0; a<>1
\(A=\left(\sqrt{a}-\dfrac{1}{\sqrt{a}}\right)\cdot\dfrac{a}{\sqrt{a}+1}=\dfrac{a-1}{\sqrt{a}}\cdot\dfrac{a}{\sqrt{a}+1}\)
\(=\sqrt{a}\left(\sqrt{a}-1\right)=a-\sqrt{a}\)
b: A=a-căn a+1/4-1/4
=(căn a-1/2)^2-1/4>=-1/4
Dấu = xảy ra khi a=1/4
20 tháng 4 2023
Câu I:
1. \(P=\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{2\sqrt{x}}{\sqrt{x}+1}+\dfrac{x-3}{x-1}\left(x\ge0;x\ne1\right)\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{x-1}-\dfrac{2\sqrt{x}\left(\sqrt{x}-1\right)}{x-1}+\dfrac{x-3}{x-1}\)
\(=\dfrac{x+\sqrt{x}-2x+2\sqrt{x}+x-3}{x-1}\)
\(=\dfrac{3\sqrt{x}-3}{x-1}=\dfrac{3\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{3}{\sqrt{x}+1}\)
2. \(\dfrac{1}{P}=\dfrac{4}{3}\)
\(\Leftrightarrow\dfrac{1}{\dfrac{3}{\sqrt{x}+1}}=\dfrac{4}{3}\)
\(\Leftrightarrow\dfrac{12}{\sqrt{x}+1}=3\)
\(\Leftrightarrow3\sqrt{x}+3=12\)
\(\Leftrightarrow\sqrt{x}=3\)
\(\Leftrightarrow x=9\left(Vì.x\ge0;x\ne1\right)\)
20 tháng 4 2023
Câu II:
1. Vì đường thẳng (d) cắt trục hoành tại điểm có hoành độ bằng 2, nên đường thẳng (d) cắt trục hoành tại điểm có tọa độ (2;0)
Thay x = 2; y = 0 vào phương trình đường thẳng (d), ta được:
\(0=\left(2-m\right).2+m+1\)
\(\Leftrightarrow4-2m+m+1=0\)
\(\Leftrightarrow-m=-5\)
\(\Leftrightarrow m=5\)
Vậy nếu m = 5 thì đưởng thẳng (d) cắt trục hoành tại điểm có hoành độ bằng 2.
2. \(\left\{{}\begin{matrix}3x+2y=11\\x-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x=12\\x-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\3-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=1\end{matrix}\right.\)
Vậy hệ phương trình có nghiệm duy nhất là (x; y) = (3; 1)
MH
25 tháng 9 2021
1) \(\sqrt{2x-5}=7\)
\(\left(\sqrt{2x-5}\right)^2=7^2\)
\(2x-5=49\)
\(2x=54\)
\(x=27\)
2) \(3+\sqrt{x-2}=4\)
\(\sqrt{x-2}=1\)
\(\left(\sqrt{x-2}\right)^2=1^2\)
\(x-2=1\)
\(x=3\)
25 tháng 9 2021
1) \(\sqrt{2x-5}=7\left(đk:x\ge\dfrac{5}{2}\right)\)
\(\Leftrightarrow2x-5=49\Leftrightarrow2x=54\Leftrightarrow x=27\left(tm\right)\)
2) \(3+\sqrt{x-2}=4\left(đk:x\ge2\right)\)
\(\Leftrightarrow\sqrt{x-2}=1\Leftrightarrow x-2=1\Leftrightarrow x=3\)
3) \(\Leftrightarrow\sqrt{\left(x-1\right)^2}=1\Leftrightarrow\left|x-1\right|=1\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=1\\x-1=-1\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=0\end{matrix}\right.\)
4) \(\Leftrightarrow\sqrt{\left(x-2\right)^2}=1\Leftrightarrow\left|x-2\right|=1\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=1\\x-2=-1\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=1\end{matrix}\right.\)
5) \(\Leftrightarrow\sqrt{\left(2x-1\right)^2}=\sqrt{\left(x+4\right)^2}\)
\(\Leftrightarrow\left|2x-1\right|=\left|x+4\right|\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-1=x+4\\2x-1=-x-4\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-1\end{matrix}\right.\)
6) \(ĐK:x\ge-2\)
\(\Leftrightarrow5\sqrt{x+2}-3\sqrt{x+2}-\sqrt{x+2}=\sqrt{x+7}\)
\(\Leftrightarrow\sqrt{x+2}=\sqrt{x+7}\)
\(\Leftrightarrow x+2=x+7\Leftrightarrow2=7\left(VLý\right)\)
Vậy \(S=\varnothing\)
7) \(ĐK:x\ge-1\)
\(\Leftrightarrow5\sqrt{2x+1}+3\sqrt{x+1}=4\sqrt{x+1}+4\sqrt{2x+1}\)
\(\Leftrightarrow\sqrt{2x+1}=\sqrt{x+1}\)
\(\Leftrightarrow2x+1=x+1\Leftrightarrow x=0\left(tm\right)\)
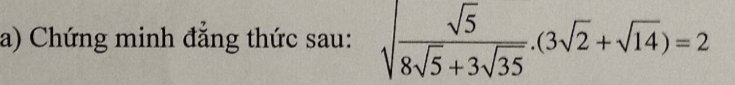
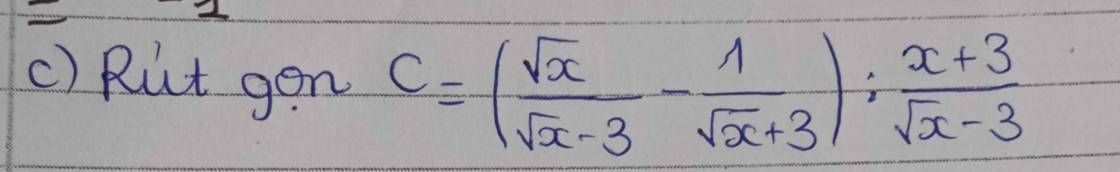
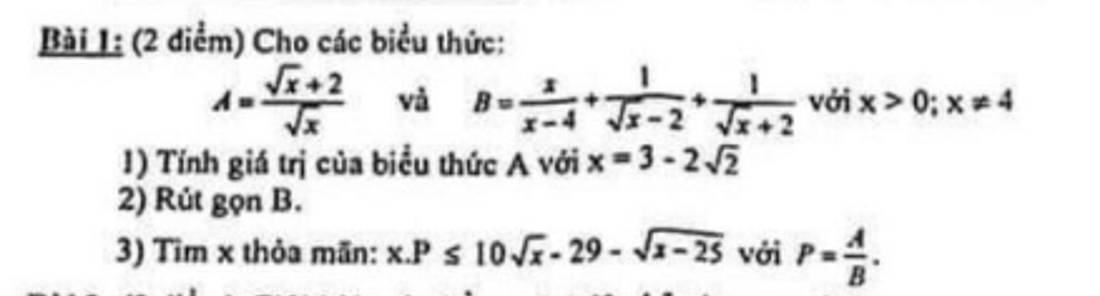
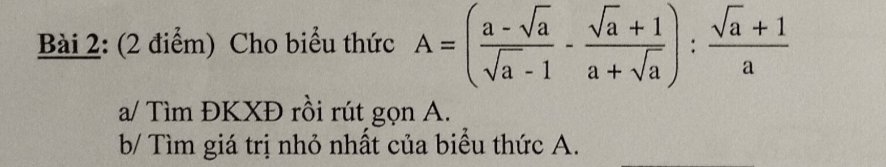
Bài nào e?
B2 á a:)