K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
T
0
Y
28 tháng 9 2023
\(a,x=36\Leftrightarrow A=\dfrac{2+\sqrt{36}}{\sqrt{36}}=\dfrac{2+6}{6}=\dfrac{4}{3}\)
\(b,B=\dfrac{x}{x-4}-\dfrac{1}{2-\sqrt{x}}+\dfrac{1}{\sqrt{x}+2}\left(dkxd:x>0,x\ne4\right)\\ =\dfrac{x}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}+\dfrac{1}{\sqrt{x}-2}+\dfrac{1}{\sqrt{x}+2}\\ =\dfrac{x+\sqrt{x}+2+\sqrt{x}-2}{x-4}\\ =\dfrac{x+2\sqrt{x}}{x-4}\\ =\dfrac{\sqrt{x}\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\\ =\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
\(c,P=\dfrac{A}{B}=\dfrac{2+\sqrt{x}}{\sqrt{x}}.\dfrac{\sqrt{x}-2}{\sqrt{x}}=\dfrac{x-4}{x}\)
Để \(Px\le\dfrac{3}{2}\left(\sqrt{x}-1\right)\) thì \(\dfrac{x-4}{x}.x\le\dfrac{3}{2}\left(\sqrt{x}-1\right)\)
\(\Leftrightarrow x-4-\dfrac{3}{2}\sqrt{x}+\dfrac{3}{2}\le0\\ \Leftrightarrow x-\dfrac{3}{2}\sqrt{x}-\dfrac{5}{2}\le0\)
\(\Leftrightarrow x\le\dfrac{25}{4}\)
Kết hợp với điều kiện \(x>0,x\ne4,x\in Z\), ta kết luận \(S=\left\{1;2;3;5;6\right\}\)
QN
0
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 1
d.
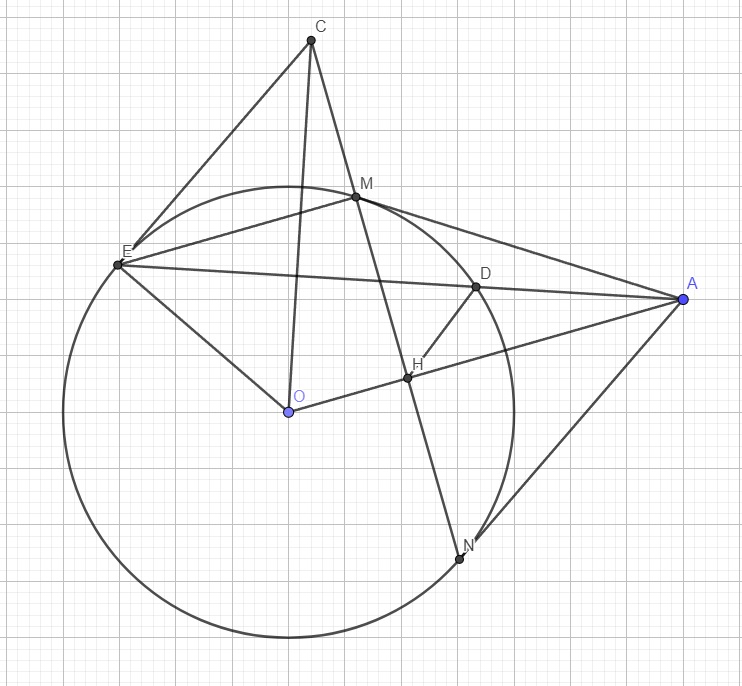
Từ câu b ta có: \(\dfrac{AD}{AO}=\dfrac{AH}{AE}\)
Xét 2 tam giác ADH và AOE có: \(\left\{{}\begin{matrix}\widehat{HAD}\text{ chung}\\\dfrac{AD}{AO}=\dfrac{AH}{AE}\end{matrix}\right.\)
\(\Rightarrow\Delta ADH\sim\Delta AOE\left(c.g.c\right)\Rightarrow\widehat{AHD}=\widehat{AEO}\)
Mà \(\widehat{AHD}+\widehat{DHO}=180^0\Rightarrow\widehat{AEO}+\widehat{DHO}=180^0\)
\(\Rightarrow\) Tứ giác DHOE nội tiếp
Lại có E và H cùng nhìn OC dưới 1 góc vuông \(\Rightarrow CHOE\) nội tiếp đường tròn đường kính OC
\(\Rightarrow C,D,H,O,E\) cùng thuộc đường tròn đường kính OC
\(\Rightarrow\widehat{CDO}\) là góc nt chắn nửa đường tròn (do OC là đường kính)
\(\Rightarrow CD\perp OD\)
\(\Rightarrow CD\) là tiếp tuyến của (O) tại D
\(\Rightarrow C\) là giao điểm 2 tiếp tuyến của (O) tại D và E
\(\Rightarrow CE=CD\) (t/c 2 tiếp tuyến cắt nhau)
Mà \(OD=OE=R\)
\(\Rightarrow OC\) là trung trực của DE \(\Rightarrow OC\perp DE\)
Theo gt I là trung điểm DE \(\Rightarrow OI\perp DE\)
\(\Rightarrow\)Đường thẳng OC trùng đường thẳng OC\(\Rightarrow O,I,C\) thẳng hàng
29 tháng 8 2021
thay \(x=3-2\sqrt{2}\) vào P ta có:
\(\dfrac{x+8}{\sqrt{x}+1}=\dfrac{3-2\sqrt{2}+8}{\sqrt{3-2\sqrt{2}}+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}-1+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}}\)
29 tháng 8 2021
\(b,x=3-2\sqrt{2}=\left(\sqrt{2}-1\right)^2\)
Thay vào P, ta được:
\(P=\dfrac{3-2\sqrt{2}+8}{\sqrt{\left(\sqrt{2}-1\right)^2}+1}=\dfrac{11-2\sqrt{2}}{\sqrt{2}}=\dfrac{11\sqrt{2}-4}{2}\)
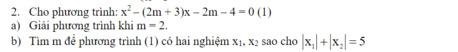
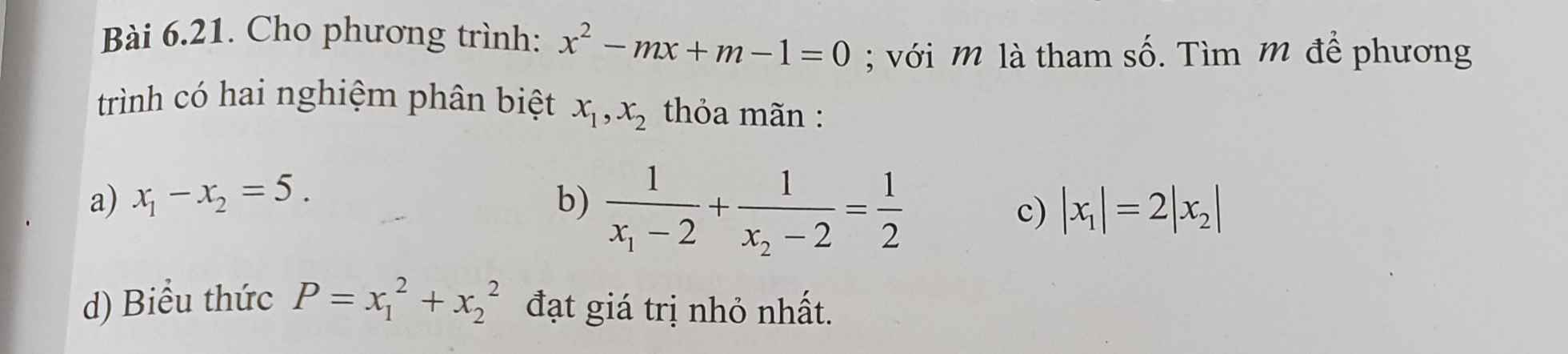
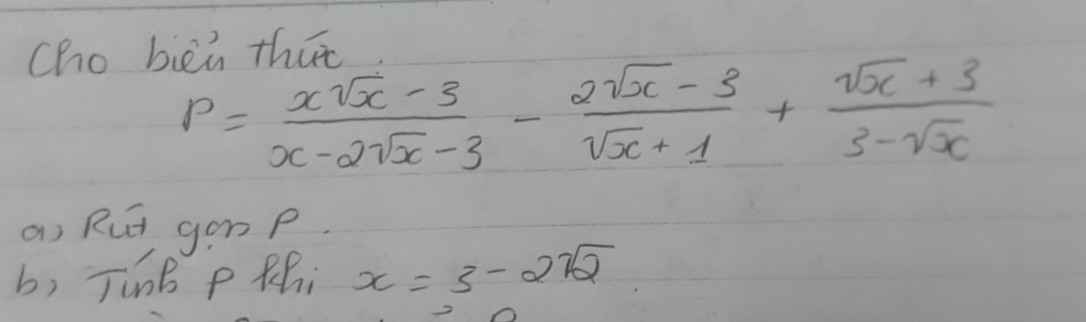
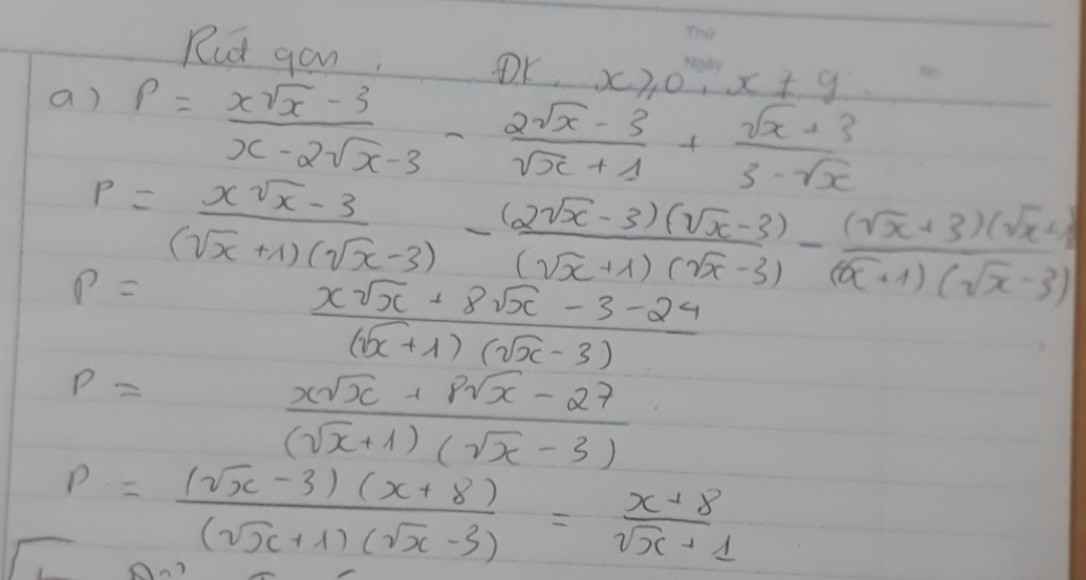
\(\Delta=\left(2m+3\right)^2+4\left(2m+4\right)=4m^2+20m+25=\left(2m+5\right)^2\ge0;\forall m\)
Pt đã cho luôn có 2 nghiệm với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m-3\\x_1x_2=-2m-4\end{matrix}\right.\)
\(\left|x_1\right|+\left|x_2\right|=5\Leftrightarrow x_1^2+x_2^2+2\left|x_1x_2\right|=25\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2+2\left|x_1x_2\right|=25\)
\(\Leftrightarrow\left(2m-3\right)^2+2\left(2m+4\right)+2\left|2m+4\right|=25\)
\(\Leftrightarrow4m^2-8m-8+4\left|m+2\right|=0\)
TH1: \(m\ge-2\)
\(\Rightarrow4m^2-8m-8+4\left(m+2\right)=0\)
\(\Leftrightarrow4m^2-4m=0\Rightarrow\left[{}\begin{matrix}m=0\\m=1\end{matrix}\right.\) (thỏa mãn)
TH2: \(m\le-2\)
\(\Rightarrow4m^2-8m-8-4\left(m+2\right)=0\)
\(\Leftrightarrow4m^2-12m-16=0\Rightarrow\left[{}\begin{matrix}m=-1\left(loại\right)\\m=4\left(loại\right)\end{matrix}\right.\)