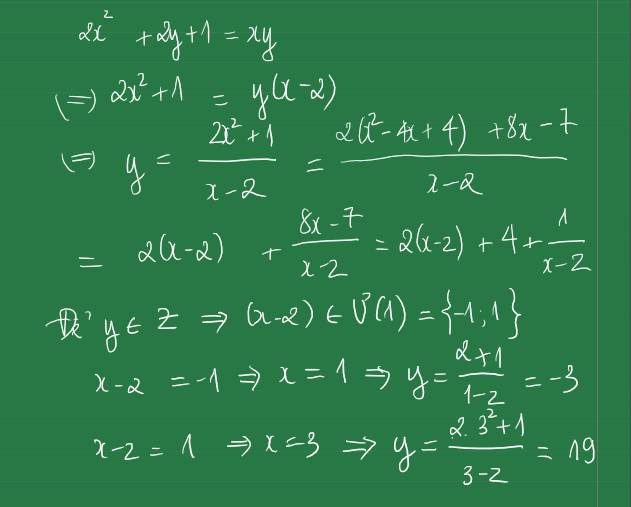K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LT
0
30 tháng 7 2021
\(x+y+xy=x^2+y^2\)
⇔ \(2xy+2x+2y=2x^2+2y^2\)
⇔ \(\left(x^2+y^2-2xy\right)+\left(x^2-2x+1\right)+\left(y^2-2y+1\right)=2\)
⇔ \(\left(x-y\right)^2+\left(x-1\right)^2+\left(y-1\right)^2=2\)
⇔ 
⇔ 
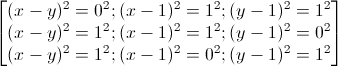
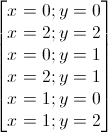
Các cặp số nguyên (x, y) thỏa mãn phương trình là : (0; 0); (2; 2); (0; 1); (2; 1); (1; 0);(1;2).
NT
1
KN
1
18 tháng 8 2023
\(x^2+y^2=3-xy\)
\(\Leftrightarrow\left(x-y\right)^2+2xy=3-xy\)
\(\Leftrightarrow\left(x-y\right)^2=3-3xy\)
\(\Leftrightarrow\left(x-y\right)^2=3\left(1-xy\right)\)
mà \(\left(x-y\right)^2\ge0,\forall x;y\inℤ\)
PT\(\Leftrightarrow\left\{{}\begin{matrix}x-y=3\\1-xy=3\end{matrix}\right.\) hay \(\left\{{}\begin{matrix}x-y=0\\1-xy=0\end{matrix}\right.\)
\(TH1:\left\{{}\begin{matrix}x-y=3\\1-xy=3\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=y+3\\xy=-2\end{matrix}\right.\)
\(\Leftrightarrow\left(x;y\right)\in\left\{\left(1;-2\right);\left(2;-1\right);\left(-1;2\right);\left(-2;1\right)\right\}\)
\(TH2:\left\{{}\begin{matrix}x-y=0\\1-xy=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=y\\xy=1\end{matrix}\right.\)
\(\Leftrightarrow\left(x;y\right)\in\left\{\left(1;1\right);\left(-1;-1\right)\right\}\)
Vậy \(\Leftrightarrow\left(x;y\right)\in\left\{\left(1;-2\right);\left(2;-1\right);\left(-1;2\right);\left(-2;1\right);\left(1;1\right);\left(-1;-1\right)\right\}\)
KN
2
NX
29 tháng 8 2023
\(x^2+y^2=3-xy\)
\(\Leftrightarrow\left(x-y\right)^2=3.\left(1-xy\right)\)
\(\Leftrightarrow x-y=3\) và \(1-xy=3\)
\(\Leftrightarrow\left(x;y\right)=\left(1;-2\right),\left(2;-1\right),\left(-1;2\right),\left(-2;1\right)\)
hoặc \(x-y=0\) và \(1-xy=0\)
\(\Leftrightarrow\left(x;y\right)=\left(1;1\right),\left(-1;-1\right)\)
NH
1
AH
Akai Haruma
Giáo viên
19 tháng 1 2021
Lời giải:PT $\Leftrightarrow x^2+x(y-2014)-(2015y+2016)=0$
Coi đây là PT bậc 2 ẩn $x$. Để pt có nghiệm nguyên thì:
$\Delta=(y-2014)^2+4(2015y+2016)=t^2$ với $t\in\mathbb{N}$
$\Leftrightarrow y^2+4032y+4064260=t^2$
$\Leftrightarrow (y+2016)^2+4=t^2$$\Leftrightarrow 4=(t-y-2016)(t+y+2016)$
Đến đây thì đơn giản rồi thì đây là dạng phương trình tích.