NQ
4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
KT
20 tháng 8 2018
c) \(x^3-9x^2+6x+16=x^3-8x^2-x^2+8x-2x+16\)
\(=x^2\left(x-8\right)-x\left(x-8\right)-2\left(x-8\right)=\left(x-8\right)\left(x^2-x-2\right)=\left(x-8\right)\left(x-2\right)\left(x+1\right)\)
d) \(2x^3+3x^2+3x+1=\left(2x+1\right)\left(x^2+x+1\right)\)
e) \(2x^3-5x^2+5x-3=\left(2x-3\right)\left(x^2-x+1\right)\)
NH
0
NQ
1
10 tháng 3 2020
(3x-2) (9x+6x+4)-(3x-1) (9x+3x+1)=x-4
(3x - 2)(15x + 4) - (3x - 1)(12x + 1) = x - 4
<=> 45x2 + 12x - 30x - 8 - (36x2 + 3x - 12x - 1) - x + 4 = 0
<=> 9x2 - 10x - 3 = 0
<=> (3x - \(\frac{5}{3}\))2 = \(\frac{52}{9}\) => \(\orbr{\begin{cases}3x-\frac{5}{3}=\frac{2\sqrt{13}}{3}\\3x-\frac{5}{3}=-\frac{2\sqrt{13}}{3}\end{cases}}\) <=> \(\orbr{\begin{cases}x=\frac{5+2\sqrt{13}}{9}\\x=\frac{5-2\sqrt{13}}{9}\end{cases}}\)
Vậy ...
8 tháng 3 2022
a: =>(3x+1)(3x-1)-(3x+1)(2x-3)=0
=>(3x+1)(3x-1-2x+3)=0
=>(3x+1)(x+2)=0
=>x=-1/3 hoặc x=-2
b: =>(3x+1)(6x+2)-(3x+1)(x-2)=0
=>(3x+1)(6x+2-x+2)=0
=>(3x+1)(5x+4)=0
=>x=-1/3 hoặc x=-4/5
MH
18 tháng 10 2023
a) \(x^2-5x+6\)
\(=x^2-2x-3x+6=x\left(x-2\right)-3\left(x-2\right)=\left(x-2\right)\left(x-3\right)\)
b) \(x^2-9x+18=x^2-3x-6x+18\)
\(=x\left(x-3\right)-6\left(x-3\right)=\left(x-3\right)\left(x-6\right)\)
c) \(x^2-6x+5=x^2-x-5x+5\)
\(=x\left(x-1\right)-5\left(x-1\right)=\left(x-1\right)\left(x-5\right)\)
d) \(3x^2+5x-30=3\left(x^2+\dfrac{5x}{3}-10\right)=3\left(x^2+2.x.\dfrac{5}{6}+\dfrac{25}{36}-\dfrac{5347}{500}\right)\)
Câu này bạn xem lại đề nha
e) \(3x^2-5x-2=3x^2-6x+x-2\)
\(3x\left(x-2\right)+x-2=\left(x-2\right)\left(3x+1\right)\)
P
28 tháng 9 2017
a) x\(^2\)+8x +15
=( x\(^2\)+3x) + ( 5x +15)
= x(x+3)+ 5 (x+3)
=(x+3) (x+5)
b)x\(^2\)-4x-12
=( x\(^2\)- 6x) +( 2x -12)
=x(x-6) + 2 (x-6)
=(x - 6) (x+2)
c)9x\(^2\)-6x-24
=(9x\(^2\)-18x)+ (12x-24)
=9x(x-2) + 12 (x -2 )
=(x-2) (9x+12)
28 tháng 9 2017
a) \(x^2+8x+15\)
\(=x^2+8x+16-1\)
\(=\left(x^2+8x+16\right)-1\)
\(=\left(x+4\right)^2-1\)
\(=\left(x+4-1\right)\left(x+4+1\right)\)
\(=\left(x+3\right)\left(x+5\right)\)
b) \(x^2-4x-12\)
\(=x^2-4x+4-16\)
\(=\left(x^2-4x+4\right)-4^2\)
\(=\left(x-2\right)^2-4^2\)
\(=\left(x-2-4\right)\left(x-2+4\right)\)
\(=\left(x-6\right)\left(x+2\right)\)
c) \(9x^2-6x-24\)
\(=9x^2-6x+1-25\)
\(=\left(9x^2-6x+1\right)-5^2\)
\(=\left(3x-1\right)^2-5^2\)
\(=\left(3x-1-5\right)\left(3x-1+5\right)\)
\(=\left(3x-6\right)\left(3x+4\right)\)
HV
27 tháng 7 2023
chuyển vế sang r phân tích thành nhân tử, có thể dùng máy tính bỏ túi nhé bạn
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
27 tháng 7 2023
câu 1: 9\(x^2\) + 12\(x\) + 5 =11
(3\(x\))2 + 2.3.\(x\) .2 + 22 + 1 = 11
(3\(x\) + 2)2 = 11 - 1
(3\(x\) + 2)2 = 10
\(\left[{}\begin{matrix}3x+2=\sqrt{10}\\3x+2=-\sqrt{10}\end{matrix}\right.\)
\(\left[{}\begin{matrix}3x=\sqrt{10}-2\\3x=-\sqrt{10}-2\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=\dfrac{\sqrt{10}-2}{3}\\x=\dfrac{-\sqrt{10}-2}{3}\end{matrix}\right.\)
Vậy S = {\(\dfrac{-\sqrt{10}-2}{3}\); \(\dfrac{\sqrt{10}-2}{3}\)}
Câu 2: 6\(x^2\) + 16\(x\) + 12 = 2\(x^2\)
6\(x^2\) + 16\(x\) + 12 - 2\(x^2\) = 0
4\(x^2\) + 16\(x\) + 12 = 0
(2\(x\))2 + 2.2.\(x\).4 + 16 - 4 = 0
(2\(x\) + 4)2 = 4
\(\left[{}\begin{matrix}2x+4=2\\2x+4=-2\end{matrix}\right.\)
\(\left[{}\begin{matrix}2x=-2\\2x=-6\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-1\\x=-3\end{matrix}\right.\)
S = { -3; -1}
3, 16\(x^2\) + 22\(x\) + 11 = 6\(x\) + 5
16\(x^2\) + 22\(x\) - 6\(x\) + 11 - 5 = 0
16\(x^2\) + 16\(x\) + 6 = 0
(4\(x\))2 + 2.4.\(x\) . 2 + 22 + 2 = 0
(4\(x\) + 2)2 + 2 = 0 (1)
Vì (4\(x\)+ 2)2 ≥ 0 ∀ ⇒ (4\(x\) + 2)2 + 2 > 0 ∀ \(x\) vậy (1) Vô nghiệm
S = \(\varnothing\)
Câu 4. 12\(x^2\) + 20\(x\) + 10 = 3\(x^2\) - 4\(x\)
12\(x^2\) + 20\(x\) + 10 - 3\(x^2\) + 4\(x\) = 0
9\(x^2\) + 24\(x\) + 10 = 0
(3\(x\))2 + 2.3.\(x\).4 + 16 - 6 = 0
(3\(x\) + 4)2 = 6
\(\left[{}\begin{matrix}3x+4=\sqrt{6}\\3x+4=-\sqrt{6}\end{matrix}\right.\)
\(\left[{}\begin{matrix}3x=-4+\sqrt{6}\\3x=-4-\sqrt{6}\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=\dfrac{\sqrt{6}-4}{3}\\x=-\dfrac{\sqrt{6}+4}{3}\end{matrix}\right.\)
S = {\(\dfrac{-\sqrt{6}-4}{3}\); \(\dfrac{\sqrt{6}-4}{3}\)}
3 tháng 8 2021
Ta có: \(E=9x^2+6x-1\)
\(=9x^2+6x+1-2\)
\(=\left(3x+1\right)^2-2\ge-2\forall x\)
Dấu '=' xảy ra khi \(x=-\dfrac{1}{3}\)
3 tháng 8 2021
\(F=\left(3x\right)^2+2.3x.1+1-2=\left(3x+1\right)^2-2\ge-2\)
Dấu = xảy ra ⇔ \(3x+1=0\Rightarrow x=\dfrac{-1}{3}\)
Vậy min của F là -2
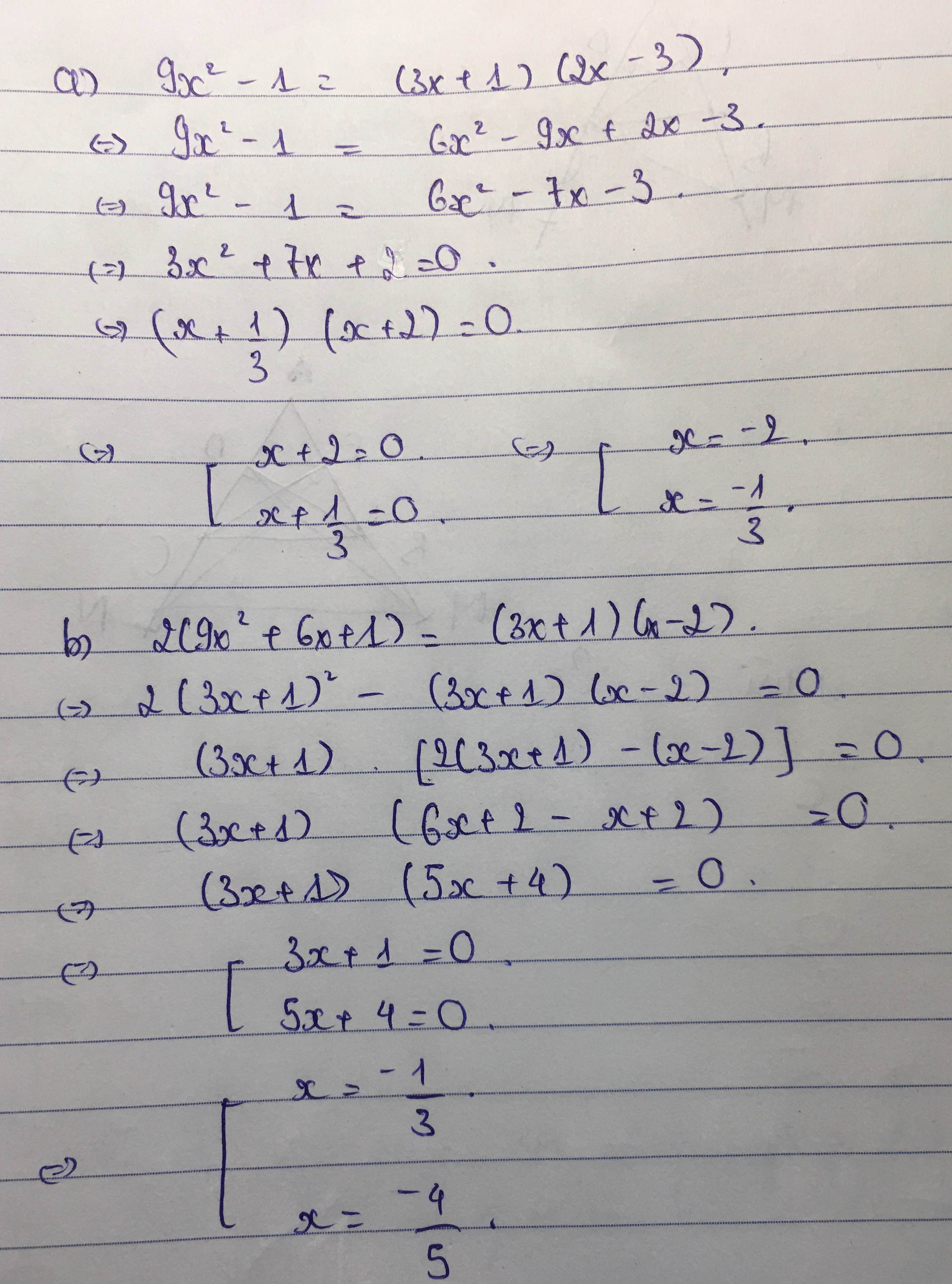
\(D=9x^2-6x+37=\left(3x\right)^2-2.3x+1+36\)
\(=\left(3x-1\right)^2+36\ge36\)
Dấu ''='' xảy ra khi \(x=\frac{1}{3}\)
Vậy GTNN D là 36 khi x = 1/3
\(D=9x^2-6x+37\)
\(=\left(3x\right)^2-2.3x.1+1^2-1^2+37\)
\(=\left[\left(3x\right)^2-2.3x.1+1^2\right]-1^2+37\)
\(=\left(3x-1\right)^2+36\ge36\forall x\)
Dấu "=" xảy ra \(\Leftrightarrow3x-1=0\Rightarrow x=\frac{1}{3}\)
Vậy \(Min_D=36\Leftrightarrow x=\frac{1}{3}\)