Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\left|x-2\right|+3x-9=0\)
\(\Leftrightarrow\left|x-2\right|=9-3x\)=> \(9-3x>0\Leftrightarrow x< 3\)
\(\Leftrightarrow\orbr{\begin{cases}x-2=9-3x\\x-2=3x-9\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}4x=11\\-2x=-7\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{11}{4}\left(tm\right)\\x=\frac{7}{2}\left(ktm\right)\end{cases}}\)

a) đặt \(\left(x^2+x\right)\)là \(y\)
ta có: \(3y^2-7y+4\)\(=0\)
<=>\(\left(3y-4\right)\left(y-1\right)=0\)
còn lại bạn tự xử nhé

a/ Với \(x\ge2\) pt trở thành:
\(x-2+3x-9=0\)
\(\Leftrightarrow4x-11=0\Rightarrow x=\frac{11}{4}\) (nhận)
Với \(x< 2\) pt trở thành:
\(2-x+3x-9=0\)
\(\Leftrightarrow2x-7=0\Rightarrow x=\frac{7}{2}>2\left(l\right)\)
b/
\(\left(x^2-5x+1\right)^2-2\left(x^2-5x+1\right)+1=0\)
\(\Leftrightarrow\left(x^2-5x+1-1\right)^2=0\)
\(\Leftrightarrow\left(x^2-5x\right)^2=0\)
\(\Leftrightarrow x^2-5x=0\)
\(\Leftrightarrow x\left(x-5\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=5\end{matrix}\right.\)

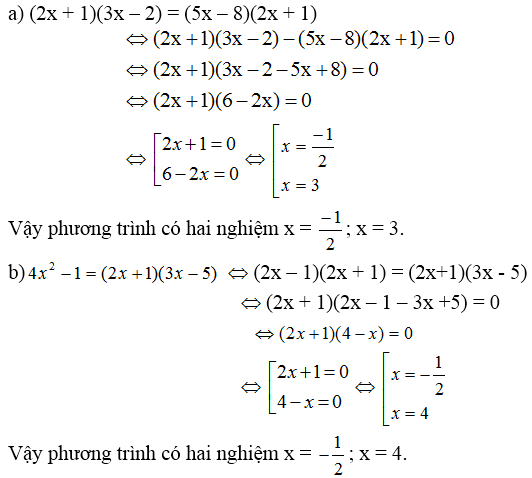
a)(2x+1)(3x-2)=(5x-8)(2x+1)
⇔(2x+1)(3x-2)-(5x-8)(2x+1)=0
⇔(2x+1)(3x-2-5x+8)=0
⇔(2x+1)(-2x+6)=0
⇔2x+1=0 hoặc -2x+6=0
1.2x+1=0⇔2x=-1⇔x=-1/2
2.-2x+6=0⇔-2x=-6⇔x=3
phương trình có 2 nghiệm x=-1/2 và x=3

a) \(x^3-2x^2-5x+6=0\)
\(x^3-x^2-x^2+x-6x+6=0\)
\(x^2\left(x-1\right)-x\left(x-1\right)-6\left(x-1\right)=0\)
\(\left(x-1\right)\left(x^2-x-6\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-1=0\\x^2-x-6=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=1\\x^2-2x+3x-6=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=1\\\left(x+3\right)\left(x-2\right)=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=1\\x=\left\{2;-3\right\}\end{cases}}\)
\(a,x^3-2x^2-5x+6=0\)
\(\Leftrightarrow\left(x^3-x^2\right)-\left(x^2-x\right)-\left(6x-6\right)=0\)
\(\Leftrightarrow x^2\left(x-1\right)-x\left(x-1\right)-6\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2-x-6\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left[\left(x^2-3x\right)+\left(2x-6\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x\left(x-3\right)+2\left(x-3\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(x-3\right)=0\)
\(\Leftrightarrow x-1=0\left(h\right)x+2=0\left(h\right)x-3=0\)
\(\Leftrightarrow x=1\left(h\right)x=-2\left(h\right)x=3\)
Vậy \(x\in\left\{-2;1;3\right\}\)
P/S: (h) là hoặc nhé

Bài làm
~ Bạn Thủy bên dưới có vẻ bị Lag mạnh, bài dễ như này mà cũng dùng denta với đen tiếc. Đéo biết làm thì đừng làm chứ đéo phải làm cái kiểu mà lớp 8 chưa học nhé bạn >.<, câu c dòng thứ hai với dòng thứ 3 không phải là thừa sao? đã vậy câu c làm sai đề nữa, bên trên là 1 - 5x. bên dưới là 1 + 5x . câu cuối cũng sai hằng đẳng thức, phải là +16x chứ hông phỉa -16x.~
a) 2x + 5 = 20 - 3x
<=> 2x + 3x = 20 + 5
<=> 5x = 25
<=> x = 5
Vậy x = 5 là nghiệm phương trình.
b) 4x2 + 5x = 0
<=> x( 4x + 5 ) = 0
<=> \(\orbr{\begin{cases}x=0\\4x+5=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=-\frac{5}{4}\end{cases}}}\)
Vậy S = { 0; -5/4 }
c) \(\left(x-2\right)^2=1-5x\)
<=> \(x^2-4x+4=1-5x\)
<=> x2 - 4x + 5x - 1 + 4 = 0
<=> x2 + x + 3 = 0
<=> \(x^2+x.2.\frac{1}{2}+\frac{1}{4}+\frac{11}{4}=0\)
<=> \(\left(x^2+x+\frac{1}{4}\right)=-\frac{11}{4}\)
\(\Leftrightarrow\left(x+\frac{1}{2}\right)^2=-\frac{11}{4}\)( vô lí )
Vậy phương trình vô nghiệm.
d) x2 + 5x + 6 = 0
<=> x2 + 2x + 3x + 6 = 0
<=> x( x + 2 ) + 3( x + 2 ) = 0
<=> ( x + 3 )( x + 2 ) = 0
<=> \(\orbr{\begin{cases}x+3=0\\x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-3\\x=-2\end{cases}}}\)
Vậy tập nghiệm phương trình S = { -3; -2 }
e) x4 - 5x2 + 4 = 0
<=> x4 - x2 - 4x2 + 4 = 0
<=> x2( x2 - 1 ) - 4( x2 - 1 ) = 0
<=> ( x2 - 1 )( x2 - 4 ) = 0
<=> ( x - 1 )( x + 1 )( x - 2 )( x + 2 ) = 0
<=> \(\orbr{\begin{cases}x-1=0\\x+1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=1\\x=-1\end{cases}}}\)
\(\orbr{\begin{cases}x-2=0\\x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=2\\x=-2\end{cases}}}\)
Vậy tập nghiệm phương trình S = { 1; -1; 2; -2 }
f) 5( x2 - 3x ) = ( 4x + 2 )2 + 1
<=> 5x2 - 15x = 16x2 + 16x + 4 + 1
<=> 5x2 - 16x2 - 15x - 16x - 4 - 1 = 0
<=> -11x2 - 31x - 5 = 0
<=> -( 11x2 + 31x + 5 ) = 0
Ta có:( 11x2 + 31x + 5 ) > 0 V x
=> -( 11x2 + 31x + 5 ) < 0 V x
=> -( 11x2 + 31x + 5 ) = 0 ( vô lí )
Vậy phương trình vô nghiệm.
a, \(2x+5=20-3x\)
\(2x+5-20+3x=0\)
\(5x-15=0\Leftrightarrow5x=15\Leftrightarrow x=3\)
b, \(4x^2+5x=0\)
\(x\left(4x+5\right)=0\)
\(x=0\)
\(4x+5=0\Leftrightarrow4x=-5\Leftrightarrow x=-\frac{5}{4}\)
c, \(\left(x-2\right)^2=1-5x\)
\(\left(x-2\right)=\pm\sqrt{1-5x}\)
\(x-2=\sqrt{1+5x}\)
\(x^2-4x+4=1+5x\)
\(x^2-4x+4-1-5x=0\)
\(x^2-9x+3=0\)
\(\Delta=b^2-4ac=\left(-9\right)^2-4.3.1=81-12=69>0\)
Nên pt có 2 nghiệm phân biệt
\(x_1=\frac{9-\sqrt{69}}{2.1}=\frac{9-\sqrt{69}}{2}\)
\(x_2=\frac{9+\sqrt{69}}{2.1}=\frac{9+\sqrt{69}}{2}\)

chẳng ai giải, thôi mình giải vậy!
a) Đặt \(y=x^2+4x+8\),phương trình có dạng:
\(t^2+3x\cdot t+2x^2=0\)
\(\Leftrightarrow t^2+xt+2xt+2x^2=0\)
\(\Leftrightarrow t\left(t+x\right)+2x\left(t+x\right)=0\)
\(\Leftrightarrow\left(2x+t\right)\left(t+x\right)=0\)
\(\Leftrightarrow\left(2x+x^2+4x+8\right)\left(x^2+4x+8+x\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x=-2\\x=-4\end{cases}}\)vậy tập nghiệm của phương trình là:S={-2;-4}
b) nhân 2 vế của phương trình với 12 ta được:
\(\left(6x+7\right)^2\left(6x+8\right)\left(6x+6\right)=72\)
Đặt y=6x+7, ta được:\(y^2\left(y+1\right)\left(y-1\right)=72\)
giải tiếp ra ta sẽ được S={-2/3;-5/3}
c) \(\left(x-2\right)^4+\left(x-6\right)^4=82\)
S={3;5}
d)s={1}
e) S={1;-2;-1/2}
f) phương trình vô nghiệm
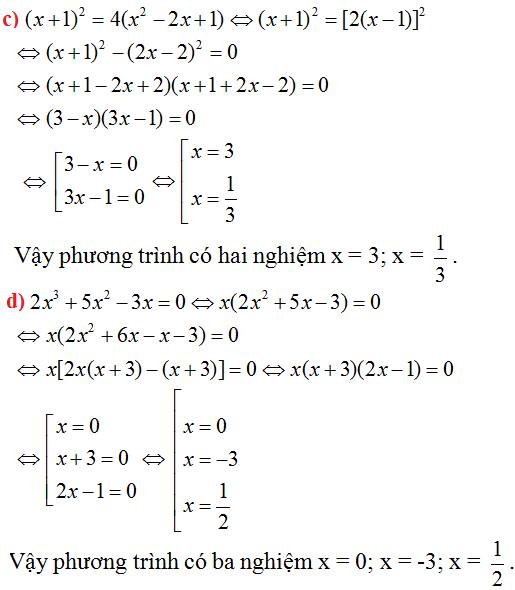
a) \(\left|x-2\right|+3x-9=0\)
\(\Leftrightarrow\left|x-2\right|=9-3x\)
+) Xét \(x\ge2\)
\(pt\Leftrightarrow x-2=9-3x\)
\(\Leftrightarrow x+3x=9+2\)
\(\Leftrightarrow4x=11\)
\(\Leftrightarrow x=\frac{11}{4}\left(tm\right)\)
+) Xét \(x< 2\)
\(pt\Leftrightarrow2-x=9-3x\)
\(\Leftrightarrow-x+3x=9-2\)
\(\Leftrightarrow2x=7\)
\(\Leftrightarrow x=\frac{7}{2}\left(ktm\right)\)
Vậy....