K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
A
1
12 tháng 12 2021
a: Xét tứ giác OBAC có
\(\widehat{OBA}+\widehat{OCA}=180^0\)
Do đó: OBAC là tứ giác nội tiếp
KV
2 tháng 6 2023
x + 3y = x(5y - 1) (1)
1/x - 3/y = -2 (2)
(1) ⇔ x(5y - 1) - x = 3y
⇔ x(5y - 2) = 3y
⇔ x = 3y/(5y - 2) (3)
Thế (3) vào (2) ta được:
(2) ⇔ 1/[3y/(5y - 2)] - 3/y = -2
⇔ (5y - 2)/3y - 3/y = -2
⇔ 5y - 2 - 9 = -6y
⇔ 5y + 6y = 11
⇔ 11y = 11
⇔ y = 1 thế vào (3) ta được:
x = 3.1/(5.1 - 2) = 1
Vậy S = {(1; 1)}
3 tháng 7 2021
1) \(A=\dfrac{x+2+x-\sqrt{x}-x-\sqrt{x}-1}{x\sqrt{x}-1}:\dfrac{\sqrt{x}-1}{5}\)
\(=\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{5}{\sqrt{x}-1}\) \(=\dfrac{5}{x+\sqrt{x}+1}\)
2) Ta thấy \(x+\sqrt{x}+1=\sqrt{x}\left(\sqrt{x}+1\right)+1>1\forall x\)
\(\Rightarrow A< 5\)
27 tháng 7 2021
Đặt \(A=\sqrt[3]{2+\sqrt{5}}+\sqrt[3]{2-\sqrt{5}}\)
\(\Leftrightarrow A^3=2+\sqrt{5}+2-\sqrt{5}+3\cdot\sqrt[3]{\left(2+\sqrt{5}\right)\left(2-\sqrt{5}\right)}\cdot\left(\sqrt[3]{2+\sqrt{5}}+\sqrt[3]{2-\sqrt{5}}\right)\)
\(\Leftrightarrow A^3=4+3\cdot\left(-1\right)\cdot A\)
\(\Leftrightarrow A^3=4-3A\)
\(\Leftrightarrow A^3+3A-4=0\)
\(\Leftrightarrow A^3-A^2+A^2-A+4A-4=0\)
\(\Leftrightarrow A^2\left(A-1\right)+A\left(A-1\right)+4\left(A-1\right)=0\)
\(\Leftrightarrow\left(A-1\right)\left(A^2+A+4\right)=0\)
\(\Leftrightarrow A=1\)
TC
27 tháng 5 2022
\(x+\sqrt{4-x^2}=2\)
\(\Leftrightarrow4-x^2=\left(2-x\right)^2\)
\(\Leftrightarrow4-x^2=4-8x+x^2\)
\(\Leftrightarrow4-x^2-4+8x-x^2=0\)
\(\Leftrightarrow8x-2x^2=0\)
\(\Leftrightarrow2x\left(4-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=0\\4-x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
TC
28 tháng 5 2022
\(x+\sqrt{1-x^2}=1\)
\(\Leftrightarrow1-x^2=\left(1-x\right)^2\)
\(\Leftrightarrow1-x^2=1-2x+x^2\)
\(\Leftrightarrow1-x^2-1+2x-x^2=0\)
\(\Leftrightarrow2x-2x^2=0\)
\(\Leftrightarrow2x\left(1-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=0\\1-x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
T
1
A
0
NT
3
19 tháng 8 2021
\(P=\left(\frac{1}{\sqrt{x}}+\frac{\sqrt{x}}{\sqrt{x}+1}\right):\frac{\sqrt{x}}{x+\sqrt{x}}\)ĐK : x > 0
\(=\left(\frac{\sqrt{x}+1+x}{\sqrt{x}\left(\sqrt{x}+1\right)}\right):\frac{1}{\sqrt{x}+1}=\frac{x+\sqrt{x}+1}{\sqrt{x}}\)
\(P=\frac{\sqrt{x}}{\sqrt{x}-1}+\frac{3}{\sqrt{x}+1}-\frac{6\sqrt{x}-4}{x-1}\)
\(=\frac{x+\sqrt{x}+3\sqrt{x}-3-6\sqrt{x}+4}{x-1}=\frac{x-2\sqrt{x}+1}{x-1}=\frac{\sqrt{x}-1}{\sqrt{x}+1}\)
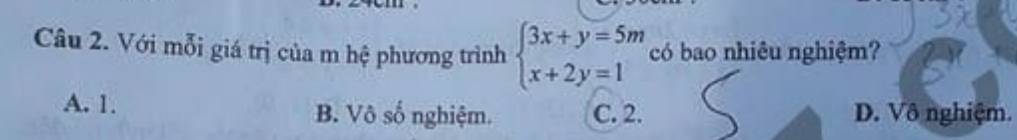
Câu 4:
Có \(36^0+54^0=90^0\Rightarrow sin36^0=cos54^0\)và \(sin54^0=cos36^0\)
\(sin36^0-cos54^0+3tan36^0.tan54^0-sin^236^0-cos^254^0\)
\(=0+3\dfrac{sin36^0}{cos36^0}.\dfrac{sin54^0}{cos54^0}-\left(sin^236^0+cos^254^0\right)\)
\(=3.\dfrac{cos54^0}{cos36^0}.\dfrac{cos36^0}{cos54^0}-2sin^236^0\)\(=3-2sin^236^0\)\(\approx2,3\)
Câu 5:
Vì \(sin\alpha=2>1\)\(\Rightarrow\alpha\in\varnothing\)
Không tính được \(cos\alpha\)
Câu 6:
\(cot\alpha=\dfrac{1}{tan\alpha}=\dfrac{1}{2}\)
Câu 7:
\(\dfrac{sin^2\alpha-cos^2\alpha}{1-2cos^2\alpha}=\dfrac{\left(sin^2\alpha+cos^2\alpha\right)-2cos^2\alpha}{1-2cos^2\alpha}=\dfrac{1-2cos^2\alpha}{1-2cos^2\alpha}=1\) ( dpcm)
Dậy sớm z UwU