K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
LK
0
VN
2
DH
7 tháng 9 2021
(2x - 4)^2 = -8 <=> (2x)^2 - 2.2x.(-4) + 16 = -8 <=> 4x^2 - 8x + 16 = -8 => 4x^2 - 8x + 24 = 0
Tính Delta là ra nhé
TT
1
25 tháng 9 2016
\(\left|x-4\right|=2x-1\)
\(\Rightarrow\orbr{\begin{cases}x-4=-\left(2x-1\right)\\x-4=2x-1\end{cases}}\)
Với x - 4 = -(2x - 1)
=> x - 4 = -2x + 1
=> -4 - 1 = -2x - x
=> -5 = -3x
=> x = 5/3
Với x - 4 = 2x - 1
=> -4 + 1 = 2x - x
=> -3 = x
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 12 2021
\(E=\dfrac{\dfrac{5}{2}\left(2x^2+3\right)+\dfrac{15}{2}}{2x^2+3}=\dfrac{5}{2}+\dfrac{15}{2\left(2x^2+3\right)}\)
Do \(2x^2+3\ge3;\forall x\Rightarrow\dfrac{15}{2\left(2x^2+3\right)}\le\dfrac{15}{2.3}=\dfrac{5}{2}\)
\(\Rightarrow E\le\dfrac{5}{2}+\dfrac{5}{2}=5\)
\(E_{max}=5\) khi \(x=0\)
5 tháng 4 2021
Đặt E(x)=0
\(\Leftrightarrow2x^2-3x=0\)
\(\Leftrightarrow x\left(2x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\2x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\2x=3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{3}{2}\end{matrix}\right.\)
Vậy: \(S=\left\{0;\dfrac{3}{2}\right\}\)
5 tháng 4 2021
Đặt E(x)=0
⇔2x2−3x=0⇔2x2−3x=0
⇔x(2x−3)=0⇔x(2x−3)=0
⇔[x=02x−3=0⇔[x=02x=3⇔⎡⎣x=0x=32⇔[x=02x−3=0⇔[x=02x=3⇔[x=0x=32
Vậy: S={0;32}
NT
2
16 tháng 6 2020
H(x) = 2x2 - 2x
H(x) = 0 <=> 2x2 - 2x = 0
<=> x( 2x - 2 ) = 0
<=> x = 0 hoặc 2x - 2 = 0
<=> x = 0 hoặc x = 1
Vậy nghiệm của H(x) là 0 và 1
16 tháng 6 2020
\(H\left(x\right)=2x^2-2x=2x\left(x-1\right)\)
Để H(x) có nghiệm => 2x(x-1)=0
\(\Leftrightarrow\orbr{\begin{cases}2x=0\\x-1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=1\end{cases}}}\)
Vậy x=0; x=1
17 tháng 10 2018
a, 1 - 2x < 7
=> -2x < 6
=> x < -3
=> x thuộc {-4; -5; -6; ...}
b, \(\left(x-1\right)\left(x-2\right)>0\)
th1 :
\(\hept{\begin{cases}x-1< 0\\x-2< 0\end{cases}\Rightarrow\hept{\begin{cases}x< 1\\x< 2\end{cases}\Rightarrow}x< 1\Rightarrow x\in\left\{0;-1;-2;...\right\}}\)
th2 :
\(\hept{\begin{cases}x-1>0\\x-2>0\end{cases}\Rightarrow\hept{\begin{cases}x>1\\x>2\end{cases}\Rightarrow}x>2\Rightarrow x\in\left\{3;4;5;...\right\}}\)
vậy_
c tương tự b
T
17 tháng 10 2018
\(a.1-2x< 7\Leftrightarrow2x< 7+1=8\Leftrightarrow x< 8:2\Leftrightarrow x< 4\)
Vậy x < 4
\(b.\left(x-1\right)\left(x-2\right)>0\)
\(\Leftrightarrow\orbr{\begin{cases}x-1>0;x-2>0\\x-1< 0;x-2< 0\end{cases}}\)
\(TH1\Leftrightarrow\orbr{\begin{cases}x-1>0\\x-2>0\end{cases}\Leftrightarrow\orbr{\begin{cases}x>0+1=1\\x>0+2=2\end{cases}\Rightarrow x>2}}\)
\(TH2\Leftrightarrow\orbr{\begin{cases}x-1< 0\\x-2< 0\end{cases}\Leftrightarrow\orbr{\begin{cases}x< 0+1=1\\x< 0+2=2\end{cases}\Rightarrow}}x< 2\)
Vậy \(x\ne2\)
TQ
1
LH
5 tháng 8 2016
Ta có: \(6x^2\ge0\)
\(2x< 6x^2\)
\(\Rightarrow6x^2+2x\ge0\)
\(\Rightarrow6x^2+2x+2017\ge2017\)
Vậy không tồn tại x khi đa thức trên bằng 0
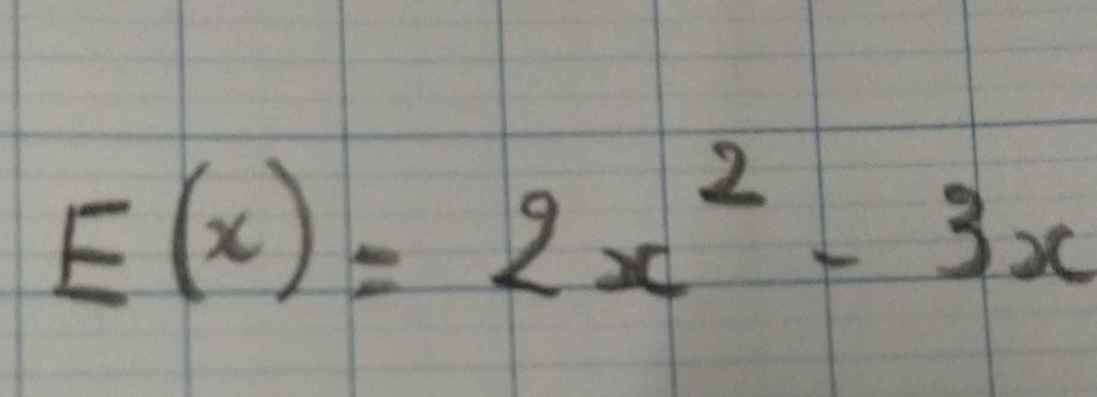
x vô nghiệm nhé bạn
mũ 2 rồi mà còn -8 đc
xin tiick
Do -8<0 mà (2x-4)^2>=0 nên PT vô nghiệm