K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NQ
Nguyễn Quốc Đạt
CTVVIP
14 tháng 10 2023
a)
\(175\cdot19+38\cdot175+43\cdot175\\ =175\cdot19+175\cdot38+175\cdot43\\ =175\cdot\left(19+38+43\right)\\ =175\cdot100\\ =17500\)
b)
\(125\cdot75+125\cdot13-80\cdot125\\ =125\cdot75+125\cdot13-125\cdot80\\ =125\cdot\left(75+13-80\right)\\ =125\cdot10\\ =125\cdot8\\ =1000\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
14 tháng 10 2023
a, 175. 19 + 38. 175 + 43. 175
= 175. 19 + 175. 38 + 175. 43
= 175.(19 + 38 + 43)
= 175. 100
= 17500
NM
2
NQ
Nguyễn Quốc Đạt
CTVVIP
5 tháng 12 2023
2/
Xét phân số \(\dfrac{2n-3}{n+1}=\dfrac{2n+2-5}{n+1}=\dfrac{2n+2}{n+1}-\dfrac{5}{n+1}=\dfrac{2\left(n+1\right)}{n+1}-\dfrac{5}{n+1}=2-\dfrac{5}{n+1}\)
\(n\in Z\Rightarrow2n-3\inƯ\left(5\right)=\left\{-1;-5;1;5\right\}\)
Ta có bảng:
| 2n - 3 | -1 | -5 | 1 | 5 |
| n | 1 | -1 | 2 | 4 |
Vậy \(n\in\left\{-1;1;2;4\right\}\)
NQ
Nguyễn Quốc Đạt
CTVVIP
5 tháng 12 2023
1/
(x + 1) + (x + 3) + (x + 5) + ... + (x + 999) = 500
<=> (x + x + x + ... + x) + (1 + 3 + 5 + ... + 999) = 500
Xét tổng A = 1 + 3 + 5 + ... + 999
Số số hạng của A là: (999 - 1) : 2 + 1 = 500
Tổng A là: (999 + 1) x 500 : 2 = 250 000
Do A có 500 số hạng nên có 500 ẩn x.
Vậy ta có: 500x + 250 000 = 500
=> 500x = -249 500
=> x = 499
Vậy x = 499
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 1 2024
\(\dfrac{1}{n\left(n+1\right)}=\dfrac{1+n-n}{n\left(n+1\right)}=\dfrac{n+1}{n\left(n+1\right)}-\dfrac{n}{n\left(n+1\right)}=\dfrac{1}{n}-\dfrac{1}{n+1}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
29 tháng 1 2024
Bài 2:
a; \(x\) - \(\dfrac{1}{2}\) = \(\dfrac{3}{10}\).\(\dfrac{5}{6}\)
\(x\) - \(\dfrac{1}{2}\) = \(\dfrac{1}{4}\)
\(x\) = \(\dfrac{1}{4}\) + \(\dfrac{1}{2}\)
\(x\) = \(\dfrac{3}{4}\)
Vậy \(x\) = \(\dfrac{3}{4}\)
b; \(\dfrac{x}{5}\) = \(\dfrac{-3}{14}\) \(\times\) \(\dfrac{7}{3}\)
\(\dfrac{x}{5}\) = \(\dfrac{-1}{2}\)
\(x\) = \(\dfrac{-1}{2}\) \(\times\) 5
\(x\) = \(\dfrac{-5}{2}\)
Vậy \(x\) = \(\dfrac{-5}{2}\);
c; \(x\) : \(\dfrac{4}{11}\) = \(\dfrac{11}{4}\) \(\times\) 2
\(x\) : \(\dfrac{4}{11}\) = \(\dfrac{11}{2}\)
\(x\) = \(\dfrac{11}{2}\) \(\times\) \(\dfrac{4}{11}\)
\(x\) = 2
Vậy \(x\) = 2
d; \(x^2\) + \(\dfrac{9}{-25}\) = \(\dfrac{2}{5}\) : \(\dfrac{5}{8}\)
\(x^2\) - \(\dfrac{9}{25}\) = \(\dfrac{16}{25}\)
\(x^2\) = \(\dfrac{16}{25}\) + \(\dfrac{9}{25}\)
\(x^2\) = \(\dfrac{25}{25}\)
\(x^2\) = 1
\(\left[{}\begin{matrix}x=-1\\x=1\end{matrix}\right.\)
Vậy \(x\)\(\in\) {-1; 1}
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
29 tháng 1 2024
Bài 3:
a; A = \(\dfrac{2}{13}\)\(\times\) \(\dfrac{5}{9}\)+ \(\dfrac{2}{13}\)\(\times\)\(\dfrac{4}{9}\) + \(\dfrac{11}{13}\)
A = \(\dfrac{2}{13}\) \(\times\)(\(\dfrac{5}{9}\) + \(\dfrac{4}{9}\)) + \(\dfrac{11}{13}\)
A = \(\dfrac{2}{13}\) \(\times\) \(\dfrac{9}{9}\) + \(\dfrac{11}{13}\)
A = \(\dfrac{2}{13}\) + \(\dfrac{11}{13}\)
A = 1
b; B = \(\dfrac{1}{10}\).\(\dfrac{4}{11}\) + \(\dfrac{1}{10}\).\(\dfrac{8}{11}\) - \(\dfrac{1}{10}\).\(\dfrac{1}{11}\)
B = \(\dfrac{1}{10}\) x (\(\dfrac{4}{11}\) + \(\dfrac{8}{11}\) - \(\dfrac{1}{11}\))
B = \(\dfrac{1}{10}\) x (\(\dfrac{12}{11}\) - \(\dfrac{1}{11}\))
B = \(\dfrac{1}{10}\) x \(\dfrac{11}{11}\)
B = \(\dfrac{1}{10}\)
3 tháng 2 2024
a) \(\dfrac{5}{11}\cdot\dfrac{5}{7}+\dfrac{5}{11}\cdot\dfrac{2}{7}+\dfrac{6}{11}=\dfrac{5}{11}\cdot\left(\dfrac{5}{7}+\dfrac{2}{7}\right)+\dfrac{6}{11}=\dfrac{5}{11}\cdot1+\dfrac{6}{11}=\dfrac{5}{11}+\dfrac{6}{11}=\dfrac{11}{11}=1\)
b) \(\dfrac{3}{13}\cdot\dfrac{6}{11}+\dfrac{3}{13}\cdot\dfrac{9}{11}-\dfrac{3}{13}\cdot\dfrac{4}{11}=\dfrac{3}{13}\cdot\left(\dfrac{6}{11}+\dfrac{9}{11}-\dfrac{4}{11}\right)=\dfrac{3}{13}\cdot\dfrac{11}{11}=\dfrac{3}{13}\cdot1=\dfrac{3}{13}\)
c) \(\dfrac{-5}{6}\cdot\dfrac{4}{19}+\dfrac{7}{12}\cdot\dfrac{4}{-19}-\dfrac{40}{57}=\dfrac{-5}{6}\cdot\dfrac{4}{19}+\dfrac{-7}{12}\cdot\dfrac{4}{19}-\dfrac{40}{57}=\dfrac{4}{19}\cdot\left(\dfrac{-5}{6}+\dfrac{-7}{12}\right)-\dfrac{40}{57}\)
\(=\dfrac{4}{19}\cdot\dfrac{-17}{12}-\dfrac{40}{47}=\dfrac{-17}{57}-\dfrac{40}{57}=\dfrac{-57}{57}=-1\)
d) \(\left(\dfrac{11}{4}\cdot\dfrac{-5}{9}+\dfrac{4}{9}\cdot\dfrac{11}{-4}\right)\cdot\dfrac{8}{33}=\left(\dfrac{11}{4}\cdot\dfrac{-5}{9}+\dfrac{-4}{9}\cdot\dfrac{11}{4}\right)\cdot\dfrac{8}{33}=\dfrac{11}{4}\cdot\dfrac{8}{33}\cdot\left(\dfrac{-5}{9}+\dfrac{-4}{9}\right)\)
\(=\dfrac{11}{4}\cdot\dfrac{8}{33}\cdot1=\dfrac{11\cdot8}{4\cdot33}=\dfrac{2}{3}\)
e) \(\left(\dfrac{12}{61}-\dfrac{31}{22}+\dfrac{14}{91}\right)\cdot\left(\dfrac{1}{2}-\dfrac{1}{3}-\dfrac{1}{6}\right)=\left(\dfrac{12}{61}-\dfrac{31}{22}+\dfrac{14}{91}\right)\cdot\left(\dfrac{1}{6}-\dfrac{1}{6}\right)\)
\(=\left(\dfrac{12}{61}-\dfrac{31}{22}+\dfrac{14}{91}\right)\cdot0=0\)
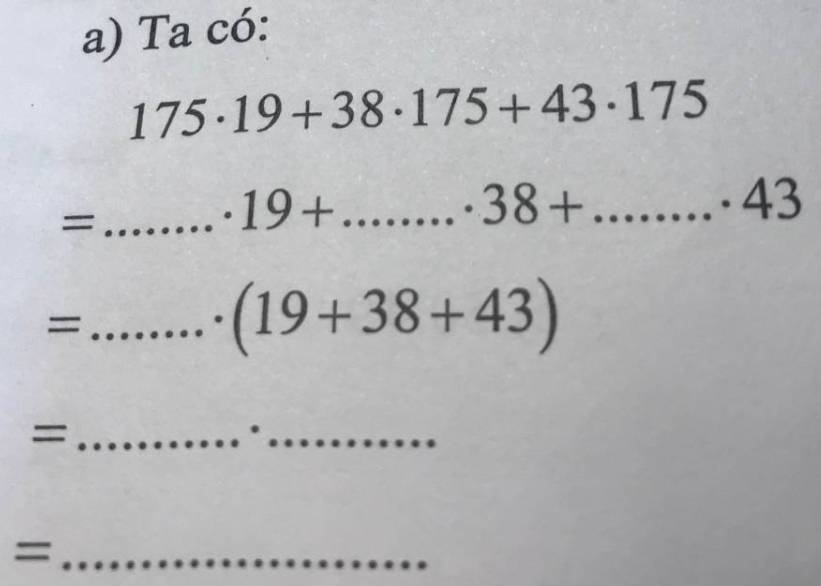
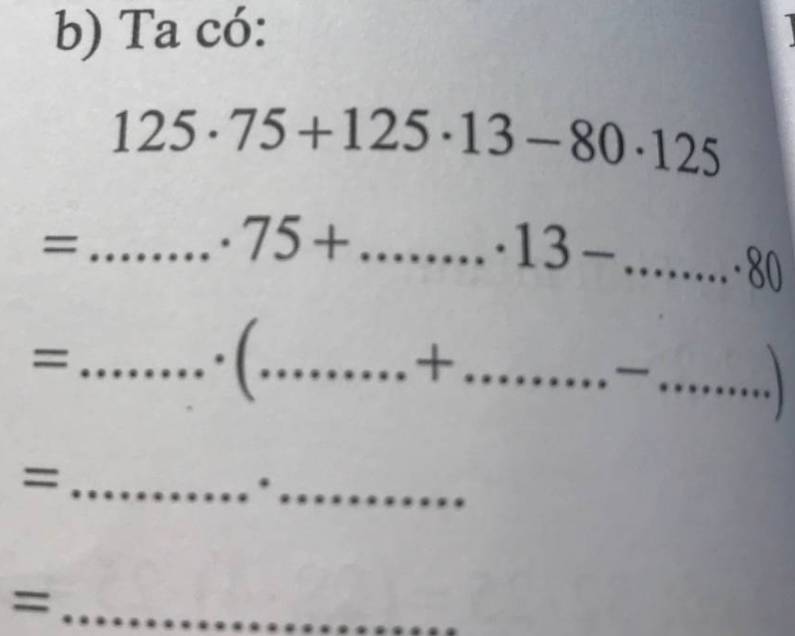
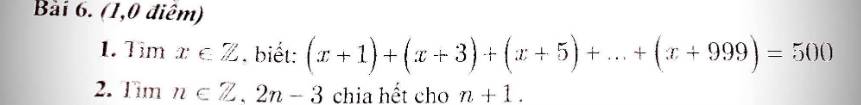
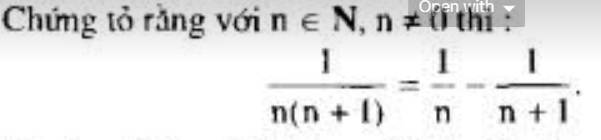
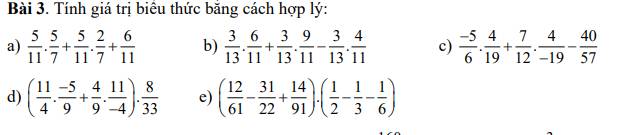
Lời giải:
Gọi số thành viên nhóm tình nguyện là $a$. Theo bài ra:
$200< a< 300$
$a-3\vdots 5,6,7$
$\Rightarrow a-3$ là BC(5,6,7)
$\Rightarrow a-3\vdots BCNN(5,6,7)$
$\Rightarrow a-3\vdots 210$
$\Rightarrow a-3\in\left\{0; 210; 420;....\right\}$
$\Rightarrow a\in\left\{3;213; 423;...\right\}$
Mà $200< a< 300$ nên $a=213$
vì số thành viên khi xếp thành hàng mỗi hàng 5 người hoặc mỗi hàng 6 người hoặc mỗi hàng 7 người đều dư 3.
vậy giả sử số thành viên bớt đi 3 người thì sẽ xế vừa đủ vào các hàng. hay nói cách khác khi số thành viên bớt đi 3 người thì số thành viên là bội chung của 5; 6; 7
bội chung nhỏ nhất của 5; 6; 7 là 5.6.7 = 210
vì số thành viên của đội lớn hơn 200 và nhỏ hơn 300 nên số thành viên của đội là 210 + 3 = 213 (thành viên)
đs.......