2023x26+55x2023-2023+2023x20
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HL
1
MH
27 tháng 1 2023
\(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}=\dfrac{1}{2023}=\dfrac{1}{a+b+c}\)
\(\dfrac{a+b}{ab}+\dfrac{a+b}{c\left(a+b+c\right)}=0\)
\(\left(a+b\right)\left(\dfrac{1}{ab}+\dfrac{1}{c\left(a+b+c\right)}\right)=0\)
\(\left(a+b\right)\left[\dfrac{ab+bc+ca+c^2}{abc\left(a+b+c\right)}\right]=0\)
\(\left(a+b\right)\left(b+c\right)\left(c+a\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}a=-b\\b=-c\\c=-a\end{matrix}\right.\)
Đến đây bạn thay vào nữa là được nhé
20 tháng 4 2023
Sửa đề: \(B=\left(\dfrac{2008}{2023}-\dfrac{2023}{2008}\right)-\left(\dfrac{-15}{2003}-\dfrac{15}{2008}\right)\)
\(=\dfrac{2008}{2023}-\dfrac{2023}{2008}+\dfrac{15}{2003}+\dfrac{15}{2008}\)
=1-1
=0
ND
1
28 tháng 2 2023
\(y\times2023-y=2023\times2021+2023\)
\(y\times\left(2023-1\right)=2023\times\left(2021+1\right)\)
\(y\times2022=2023\times2022\)
\(y=2023\times2022\div2022\)
\(y=2023\)
CH
9 tháng 11 2023
\(\text{ 2023 x 10 - 2023 x 8 - 2023}\)
\(\text{= 2023 x (10-8-1)}\)
\(\text{= 2023 x 1}\)
\(\text{= 2028}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
9 tháng 11 2023
2023 \(\times\) 10 - 2023 \(\times\) 8 - 2023
= 2023 \(\times\) 10 - 2023 \(\times\) 8 - 2023 \(\times\) 1
= 2023 \(\times\) (10 - 8 - 1)
= 2023 x 1
= 2023
12 tháng 8 2023
\(\dfrac{x-2023}{6}+\dfrac{x-2023}{10}+\dfrac{x-2023}{15}+\dfrac{x-2023}{21}=\dfrac{8}{21}\)
\(\left(x-2023\right)\left(\dfrac{1}{6}+\dfrac{1}{10}+\dfrac{1}{15}+\dfrac{1}{21}\right)=\dfrac{8}{21}\)
\(\left(x-2023\right).\dfrac{8}{21}=\dfrac{8}{21}\)
\(x-2023=1\)
\(x=2024\)
Vậy..............
12 tháng 8 2023
\(...\Rightarrow\left(x-2023\right)\left(\dfrac{1}{6}+\dfrac{1}{10}+\dfrac{1}{15}+\dfrac{1}{21}\right)=\dfrac{8}{21}\)
\(\Rightarrow\left(x-2023\right)\left(\dfrac{35+21+14+1}{210}\right)=\dfrac{8}{21}\)
\(\Rightarrow\left(x-2023\right).\dfrac{71}{210}=\dfrac{8}{21}\)
\(\Rightarrow\left(x-2023\right).\dfrac{71}{210}=\dfrac{8}{21}.\dfrac{210}{71}=\dfrac{80}{71}\)
\(\Rightarrow x-2023=\dfrac{80}{71}\Rightarrow x=\dfrac{80}{71}+2023=\dfrac{143713}{71}\)
HK
Cho \(A=\dfrac{2023^{30}+5}{2023^{31}+5}\) và \(B=\dfrac{2023^{31}+5}{2023^{32}+5}\). So sánh A và B
2
17 tháng 4 2023
Áp dụng tính chất : Nếu \(\dfrac{a}{b}< 1\) thì \(\dfrac{a}{b}< \dfrac{a+n}{b+n}\) ( a; b; n ϵ N , b; n ≠ 0 )
Ta có \(\dfrac{2023^{31}+5}{2023^{32}+5}< 1\)
⇒ \(B=\dfrac{2023^{31}+5}{2023^{32}+5}< \dfrac{2023^{31}+5+2018}{2023^{32}+5+2018}=\dfrac{2023^{31}+2023}{2023^{32}+2023}=\dfrac{2023\left(2023^{30}+1\right)}{2023\left(2023^{31}+1\right)}=\dfrac{2023^{30}+1}{2023^{31}+1}=A\)Vậy A > B
XO
17 tháng 4 2023
Ta có 2023A = \(\dfrac{2023.\left(2023^{30}+5\right)}{2023^{31}+5}=\dfrac{2023^{31}+5.2023}{2023^{31}+5}\)
\(=1+\dfrac{2022.5}{2023^{31}+5}\)
Lại có 2023B = \(\dfrac{2023.\left(2023^{31}+5\right)}{2023^{32}+5}=\dfrac{2023^{32}+2023.5}{2023^{32}+5}\)
\(=1+\dfrac{2022.5}{2023^{32}+5}\)
Dễ thấy 202331 + 5 < 202332 + 5
\(\Leftrightarrow\dfrac{2022.5}{2023^{31}+5}>\dfrac{2022.5}{2023^{32}+5}\)
\(\Leftrightarrow1+\dfrac{2022.5}{2023^{31}+5}>1+\dfrac{2022.5}{2023^{32}>5}\)
\(\Leftrightarrow2023A>2023B\Leftrightarrow A>B\)
LN
0
TL
1
23 tháng 2 2023
\(2023A=\dfrac{2023^{31}+4046}{2023^{31}+2}=1+\dfrac{4044}{2023^{31}+2}\)
\(2023B=\dfrac{2023^{32}+4046}{2023^{32}+2}=1+\dfrac{4044}{2023^{32}+2}\)
mà 2023^31+2<2023^32+2
nên A>B
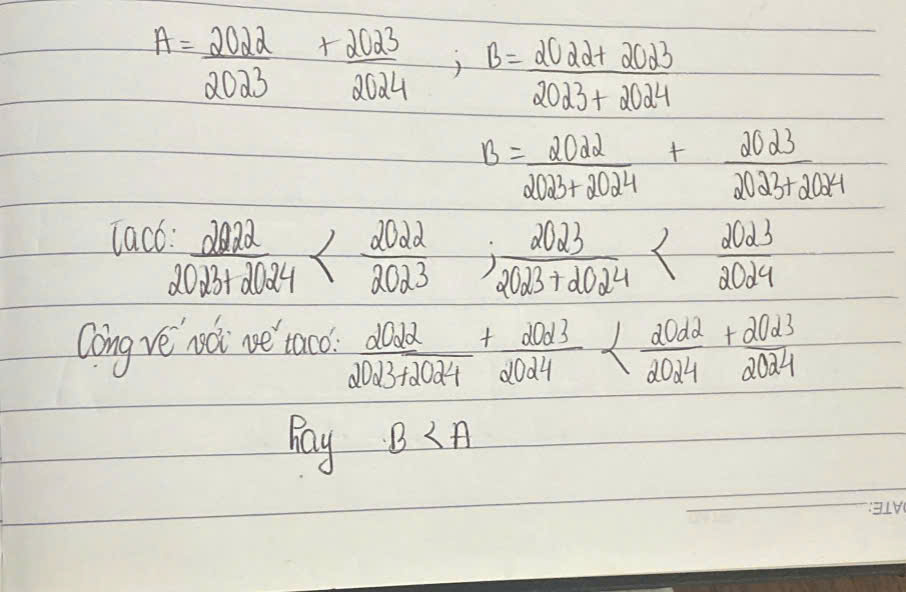
\(\) 2023 x 26 + 55 x 2023 - 2023 + 2023 x 20
= 2023 x ( 26 + 55 - 1 + 20 )
= 2023 x 100
= 202300
2023x(26+50+55-1)
R
=2023x130
=tự biết😅