giải bất phương trình x/x-2 + x+2/x > 2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
11 tháng 9 2021
\(a,f'\left(x\right)=3x^2-6x\\ f'\left(x\right)\le0\Leftrightarrow3x^2-6x\le0\\ \Leftrightarrow3x\left(x-2\right)\le0\Leftrightarrow0\le x\le2\)
AH
Akai Haruma
Giáo viên
11 tháng 9 2021
Lời giải:
a. $f'(x)\leq 0$
$\Leftrightarrow 3x^2-6x\leq 0$
$\Leftrightarrow x(x-2)\leq 0$
$\Leftrightarrow 0\leq x\leq 2$
b.
$f'(x)=x^2-3x+2=0$
$\Leftrightarrow 3x^2-6x=x^2-3x+2=0$
$\Leftrightarrow 3x(x-2)=(x-1)(x-2)=0$
$\Leftrightarrow x-2=0$
$\Leftrightarrow x=2$
c.
$g(x)=f(1-2x)+x^2-x+2022$
$g'(x)=(1-2x)'f(1-2x)'_{1-2x}+2x-1$
$=-2[3(1-2x)^2-6(1-2x)]+2x-1$
$=-24x^2+2x+5$
$g'(x)\geq 0$
$\Leftrightarrow -24x^2+2x+5\geq 0$
$\Leftrightarrow (5-12x)(2x-1)\geq 0$
$\Leftrightarrow \frac{-5}{12}\leq x\leq \frac{1}{2}$
H
11 tháng 5 2023
`|5x| = - 3x + 2`
Nếu `5x>=0<=> x>=0` thì phương trình trên trở thành :
`5x =-3x+2`
`<=> 5x +3x=2`
`<=> 8x=2`
`<=> x= 2/8=1/4` ( thỏa mãn )
Nếu `5x<0<=>x<0` thì phương trình trên trở thành :
`-5x = -3x+2`
`<=>-5x+3x=2`
`<=> 2x=2`
`<=>x=1` ( không thỏa mãn )
Vậy pt đã cho có nghiệm `x=1/4`
__
`6x-2<5x+3`
`<=> 6x-5x<3+2`
`<=>x<5`
Vậy bpt đã cho có tập nghiệm `x<5`
HH
2
24 tháng 7 2021
\(\left|x-5\right|=2x\)ĐK : x>=0
TH1 : x - 5 = 2x <=> x = -5 ( loại )
TH2 : x - 5 = -2x <=> 3x = 5 <=> x = 5/3 ( tm )
Vậy tập nghiệm pt là S = { 5/3 }
\(\left(x-2\right)^2+2\left(x-1\right)\le x^2+4\)
\(\Leftrightarrow x^2-4x+4+2x-2-x^2-4\le0\)
\(\Leftrightarrow-2x-2\le0\Leftrightarrow x+1\ge0\Leftrightarrow x\ge-1\)
Vậy tập nghiệm bft là S = { x | x > = -1 }
25 tháng 7 2021
Ta có: \(\left|x-5\right|=2x\)
\(\Leftrightarrow\left[{}\begin{matrix}x-5=2x\left(x\ge5\right)\\x-5=-2x\left(x< 5\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x-2x=5\\x+2x=5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}-x=5\\3x=5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-5\left(loại\right)\\x=\dfrac{5}{3}\left(nhận\right)\end{matrix}\right.\)
HT
2
KY
18 tháng 9 2019
\(\frac{9}{x^2-4}=\frac{x-1}{x+2}+\frac{3}{x-2}\)
\(ĐKXĐ:x\ne\pm2\)
\(pt\Leftrightarrow\frac{9}{x^2-4}=\frac{x^2-3x+2}{x^2-4}+\frac{3x+6}{x^2-4}\)
\(\Leftrightarrow\frac{9}{x^2-4}=\frac{x^2+8}{x^2-4}\)
\(\Leftrightarrow x^2+8=9\Leftrightarrow x=\pm1\left(tm\right)\)
Vậy pt có 2 nghiệm là 1 và -1
18 tháng 9 2019
Điều kện : \(x+2\ne0\) và \(x-2\ne0\Leftrightarrow x=\pm2\)
( Khi đó \(x^2-4=\left(x+2\right)\left(x-2\right)\ne0\) )
\(\frac{9}{x^2-4}=\frac{x-1}{x+2}+\frac{3}{x-2}\)
\(\Leftrightarrow\frac{9}{\left(x-2\right)\left(x+2\right)}=\frac{\left(x-1\right)\left(x-2\right)+3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Rightarrow x^2-3x+2+3x+6=9\Leftrightarrow x^2=1\Leftrightarrow x=\pm1\)
Vậy tập nghiệm của PT là: \(S=\left\{-1;1\right\}\)
Chúc bạn học tốt !!!
15 tháng 5 2021
`x/(x-2)+(x+2)/x>2`
`<=>(x^2+x^2-4-2(x^2-4))/(x(x-2))>0`
`<=>4/(x(x-2))>0`
`<=>x(x-2)>0``
`<=>` $\left[ \begin{array}{l}\begin{cases}x>0\\x>2\end{cases}\\\begin{cases}x<0\\x<2\end{cases}\end{array} \right.$
`<=>` $\left[ \begin{array}{l}x>2\\x<0\end{array} \right.$
KH
1
14 tháng 1 2022
\(\Leftrightarrow x^2-4x+4+2x^2-4x+2-x^2< =4\)
=>-8x<=-2
hay x>=1/4
. Ta có:
CM
9 tháng 3 2017
a) Sai lầm là coi -2 là hạng từ và chuyển vế hạng tử này trong khi -2 là một nhân tử.
Lời giải đúng:
-2x > 23
⇔ x < 23 : (-2) (chia cho số âm nên đổi chiều)
⇔ x < -11,5
Vậy nghiệm của bất phương trình là x < -11,5
b) Sai lầm là nhân hai vế của bất phương trình với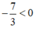 
mà không đổi chiều bất phương trình.
Lời giải đúng:
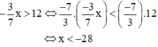
Vậy nghiệm của bất phương trình là x < -28
PT
1
CM
9 tháng 3 2018
Ta có: (x – 2)(x + 2) > x(x – 4) ⇔ x 2 – 4 > x 2 – 4x
⇔ x 2 – 4 – x 2 + 4x > 0
⇔ 4x – 4 > 0 ⇔ x > 1
Vậy tập nghiệm của bất phương trình là: {x|x > 1}
đk: \(\hept{\begin{cases}x\ne0\\x\ne2\end{cases}}\)
Ta có: \(\frac{x}{x-2}+\frac{x+2}{x}>2\)
\(\Leftrightarrow\frac{x^2+\left(x-2\right)\left(x+2\right)-2x\left(x-2\right)}{x\left(x-2\right)}>0\)
\(\Leftrightarrow\frac{x^2+x^2-4-2x^2+4x}{x\left(x-2\right)}>0\)
\(\Leftrightarrow\frac{4x-4}{x\left(x-2\right)}>0\Rightarrow\frac{x-1}{x\left(x-2\right)}>0\)
Nếu \(x-1>0\) và \(x\left(x-2\right)>0\)
=> \(x>1\) và \(\orbr{\begin{cases}x< 0\\x>2\end{cases}}\) => x > 2
Nếu \(\hept{\begin{cases}x-1< 0\\x\left(x-2\right)< 0\end{cases}}\Leftrightarrow\hept{\begin{cases}x< 1\\0< x< 2\end{cases}}\Leftrightarrow0< x< 1\)
Vậy \(\orbr{\begin{cases}x>2\\0< x< 1\end{cases}}\)
\(\frac{x}{x-2}+\frac{x+2}{x}>2\Leftrightarrow\frac{x^2+x^2-4}{x\left(x-2\right)}>2\)
\(\Leftrightarrow\frac{2x^2-4-2x\left(x-2\right)}{x\left(x-2\right)}>0\Leftrightarrow\frac{-4+4x}{x\left(x-2\right)}>0\)
TH1 : \(\hept{\begin{cases}-4\left(1-x\right)>0\\x\left(x-2\right)>0\end{cases}\Leftrightarrow\hept{\begin{cases}x< 1\\x>2\end{cases}}}\)
TH2 : \(\hept{\begin{cases}-4\left(1-x\right)< 0\\x\left(x-2\right)< 0\end{cases}\Leftrightarrow\hept{\begin{cases}x< 1\\x< 0\end{cases}\Leftrightarrow x< 0}}\)
\(\Leftrightarrow x>2;x< 0\)
Vậy tập nghiệm của bất pt là S = { \(x>2;x< 0\)}