Cho hình chóp S.ABCD có đáy là hình bình hành. Lấy điểm M sao cho \(\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+\overrightarrow{MD}+\overrightarrow{MS}=\overrightarrow{0}\) . Mặt phẳng đi qua AM cắt SB, SC, SD thứ tự tại B'; C'; D'. Tính \(\dfrac{BB'}{SB'}+\dfrac{CC'}{SC'}+\dfrac{DD'}{SD'}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
Ta có: \(\overrightarrow {AM} = - \overrightarrow {MA} ,\;\overrightarrow {DM} = - \overrightarrow {MD} \)
\( \Rightarrow \overrightarrow {MB} - \overrightarrow {MA} = \overrightarrow {MB} + \overrightarrow {AM} = \overrightarrow {AM} + \overrightarrow {MB} = \overrightarrow {AB} \)
Tương tự ta có: \(\overrightarrow {MC} - \overrightarrow {MD} = \overrightarrow {MC} + \overrightarrow {DM} = \overrightarrow {DM} + \overrightarrow {MC} = \overrightarrow {DC} \)
Mà \(\overrightarrow {AB} = \overrightarrow {DC} \)(do ABCD là hình bình hành)
\( \Rightarrow \overrightarrow {MB} - \overrightarrow {MA} = \overrightarrow {MC} - \overrightarrow {MD} \) (đpcm)
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
Cách 1:
Do ABCD là hình bình hành nên \(\overrightarrow {AB} = \overrightarrow {DC} \)
\(\begin{array}{l} \Rightarrow \overrightarrow {AM} + \overrightarrow {MB} = \overrightarrow {DM} + \overrightarrow {MC} \\ \Leftrightarrow - \overrightarrow {MA} + \overrightarrow {MB} = - \overrightarrow {MD} + \overrightarrow {MC} \\ \Leftrightarrow \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \end{array}\)
Cách 2:
Ta có: \(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \Leftrightarrow \overrightarrow {MA} - \overrightarrow {MB} = \overrightarrow {MD} - \overrightarrow {MC} \) (*)
Áp dụng quy tắc hiệu ta có: \(\overrightarrow {MA} - \overrightarrow {MB} = \overrightarrow {BA} ;\;\;\overrightarrow {MD} - \overrightarrow {MC} = \overrightarrow {CD} \)
Do đó (*) \( \Leftrightarrow \overrightarrow {BA} = \overrightarrow {CD} \) (luôn đúng do ABCD là hình bình hành)
Cách 3:
Ta có:
\(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {BA} + \overrightarrow {MD} + \overrightarrow {DC} = \overrightarrow {MB} + \overrightarrow {MD} + \left( {\overrightarrow {BA} + \overrightarrow {DC} } \right)\)
Vì ABCD là hình bình hành nên \(\overrightarrow {AB} = \overrightarrow {DC} \)\( \Rightarrow - \overrightarrow {BA} = \overrightarrow {DC} \) hay \(\overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow 0 \)
\( \Rightarrow \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \) (đpcm)
HQ
Hà Quang Minh
Giáo viên
25 tháng 9 2023
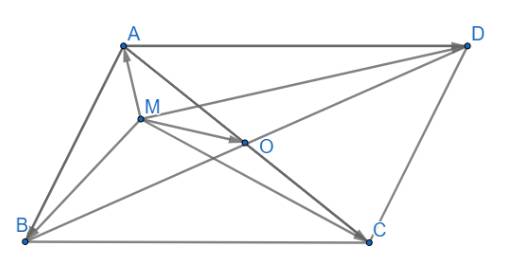
a) \(\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} + \overrightarrow {MD} = 4\overrightarrow {MO} \)
\( \Leftrightarrow \overrightarrow {MO} + \overrightarrow {OA} + \overrightarrow {MO} + \overrightarrow {OB} + \overrightarrow {MO} + \overrightarrow {OC} + \overrightarrow {MO} + \overrightarrow {OD} = 4\overrightarrow {MO} \)
\( \Leftrightarrow 4\overrightarrow {MO} + \left( {\overrightarrow {OA} + \overrightarrow {OB} } \right) + \left( {\overrightarrow {OC} + \overrightarrow {OD} } \right) = 4\overrightarrow {MO} \)
\( \Leftrightarrow 4\overrightarrow {MO} + \overrightarrow 0 + \overrightarrow 0 = 4\overrightarrow {MO} \\ \Leftrightarrow 4\overrightarrow {MO} = 4\overrightarrow {MO} \) (luôn đúng)
(vì O là giao điểm 2 đường chéo nên là trung điểm của AB, CD)
b) ABCD là hình bình hành nên ta có \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} \)
Suy ra \(\)\(\overrightarrow {AB} + \overrightarrow {AC} + \overrightarrow {AD} = \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right) + \overrightarrow {AC} = \overrightarrow {AC} + \overrightarrow {AC} = 2\overrightarrow {AC} \) (đpcm)
10 tháng 12 2023
\(\overrightarrow{MA}-\overrightarrow{MB}=\overrightarrow{BM}+\overrightarrow{MA}=\overrightarrow{BA}\)(2)
\(\overrightarrow{MD}-\overrightarrow{MC}=\overrightarrow{CM}+\overrightarrow{MD}=\overrightarrow{CD}\)(1)
Vì ABCD là hình vuông nên \(\overrightarrow{BA}=\overrightarrow{CD}\left(3\right)\)
Từ (1),(2),(3) suy ra \(\overrightarrow{MA}-\overrightarrow{MB}=\overrightarrow{MD}-\overrightarrow{MC}\)
=>\(\overrightarrow{MA}+\overrightarrow{MC}=\overrightarrow{MB}+\overrightarrow{MD}\)
HQ
Hà Quang Minh
Giáo viên
25 tháng 9 2023
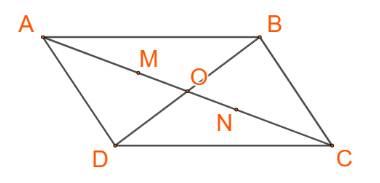
a) Áp dụng tính chất trọng tâm ta có: \(\overrightarrow {MA} + \overrightarrow {MD} + \overrightarrow {MB} = \overrightarrow 0 \)
Suy ra M là trọng tâm của tam giác ADB
Vậy M nằm trên đoạn thẳng AO sao cho \(AM = \frac{2}{3}AO\)
b) Tiếp tục áp dụng tính chất trọng tâm \(\overrightarrow {ND} + \overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \)
Suy ra N là trọng tâm của tam giác BCD
Vậy N nằm trên đoạn thẳng OD sao cho \(ON = \frac{1}{3}OD\)
c) Áp dụng tính chất trung điểm ta có: \(\overrightarrow {PM} + \overrightarrow {PN} = \overrightarrow 0 \)
Suy ra P là trung điểm của đoạn thẳng MN
Vậy điểm P trùng với điểm O.
BT
12 tháng 5 2017
Do là giao điểm của hai đường chéo hình bình hành nên:
\(\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+\overrightarrow{MD}\)\(=\overrightarrow{MO}+\overrightarrow{OA}+\overrightarrow{MO}+\overrightarrow{OB}+\overrightarrow{MO}+\overrightarrow{OC}+\overrightarrow{MO}+\overrightarrow{OD}\)
\(=4\overrightarrow{MO}+\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}\)
\(=4\overrightarrow{MO}\) (ĐPCM).
Gọi O là giao điểm AC và BD, theo t/c hình bình hành \(\Rightarrow O\) là trung điểm AC và BD
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{OA}+\overrightarrow{OC}=\overrightarrow{0}\\\overrightarrow{OB}+\overrightarrow{OD}=\overrightarrow{0}\end{matrix}\right.\)
Từ giả thiết:
\(\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+\overrightarrow{MD}+\overrightarrow{MS}=\overrightarrow{0}\)
\(\Leftrightarrow\overrightarrow{MO}+\overrightarrow{OA}+\overrightarrow{MO}+\overrightarrow{OB}+\overrightarrow{MO}+\overrightarrow{OC}+\overrightarrow{MO}+\overrightarrow{OD}+\overrightarrow{MO}+\overrightarrow{OS}=\overrightarrow{0}\)
\(\Leftrightarrow5.\overrightarrow{MO}+\overrightarrow{OS}=0\)
\(\Leftrightarrow\overrightarrow{OM}=\dfrac{1}{5}\overrightarrow{OS}\)
Hay M là điểm thuộc đoạn thẳng OS sao cho \(OM=\dfrac{1}{5}OS\) \(\Rightarrow SM=4MO\)
Do M thuộc OS \(\Rightarrow M\in\left(SAC\right)\), kéo dài AM cắt SC tại \(C'\) \(\Rightarrow C'\) là điểm cố định (bất chấp vị trí mặt phẳng (P))
Áp dụng định lý Menelaus trong tam giác SOC với 3 điểm A, M, C' thẳng hàng:
\(\dfrac{MS}{MO}.\dfrac{OA}{AC}.\dfrac{CC'}{C'S}=1\Rightarrow4.\dfrac{1}{2}.\dfrac{CC'}{C'S}=1\Rightarrow\dfrac{CC'}{SC'}=\dfrac{1}{2}\)
Bây giờ tới B' và D'.
Cách đơn giản nhất là đề ko cho biết rõ về mp (P), nó chỉ cần chứa AM là đủ, do đó ta chọn vị trí đơn giản nhất của (P) để tính, đó là (P) song song BD. Khi đó, qua M kẻ đường thẳng song song BD lần lượt cắt SB, SD tại B' và D'
Theo định lý Talet:
\(\dfrac{BB'}{SB'}=\dfrac{DD'}{SD'}=\dfrac{MO}{SM}=\dfrac{1}{4}\)
\(\Rightarrow\dfrac{BB'}{SB'}+\dfrac{CC'}{SC'}+\dfrac{DD'}{SD'}=\dfrac{1}{4}+\dfrac{1}{2}+\dfrac{1}{4}=1\)
Trong trường hợp ko muốn làm kiểu chọn mp đặc biệt này thì ta có thể chọn vị trí bất kì cho B', nhưng sẽ tốn thời gian hơn nhiều. Nếu em cần thì cũng có thể giải quyết theo cách ấy.