Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Do là giao điểm của hai đường chéo hình bình hành nên:
\(\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}+\overrightarrow{MD}\)\(=\overrightarrow{MO}+\overrightarrow{OA}+\overrightarrow{MO}+\overrightarrow{OB}+\overrightarrow{MO}+\overrightarrow{OC}+\overrightarrow{MO}+\overrightarrow{OD}\)
\(=4\overrightarrow{MO}+\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}\)
\(=4\overrightarrow{MO}\) (ĐPCM).

Ta có: \(\overrightarrow {AM} = - \overrightarrow {MA} ,\;\overrightarrow {DM} = - \overrightarrow {MD} \)
\( \Rightarrow \overrightarrow {MB} - \overrightarrow {MA} = \overrightarrow {MB} + \overrightarrow {AM} = \overrightarrow {AM} + \overrightarrow {MB} = \overrightarrow {AB} \)
Tương tự ta có: \(\overrightarrow {MC} - \overrightarrow {MD} = \overrightarrow {MC} + \overrightarrow {DM} = \overrightarrow {DM} + \overrightarrow {MC} = \overrightarrow {DC} \)
Mà \(\overrightarrow {AB} = \overrightarrow {DC} \)(do ABCD là hình bình hành)
\( \Rightarrow \overrightarrow {MB} - \overrightarrow {MA} = \overrightarrow {MC} - \overrightarrow {MD} \) (đpcm)

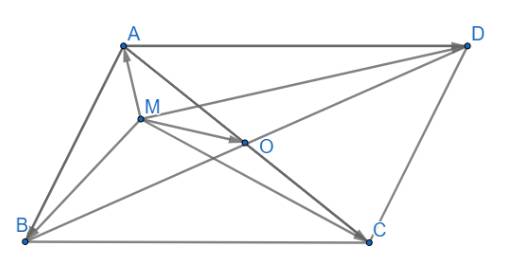
a) \(\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} + \overrightarrow {MD} = 4\overrightarrow {MO} \)
\( \Leftrightarrow \overrightarrow {MO} + \overrightarrow {OA} + \overrightarrow {MO} + \overrightarrow {OB} + \overrightarrow {MO} + \overrightarrow {OC} + \overrightarrow {MO} + \overrightarrow {OD} = 4\overrightarrow {MO} \)
\( \Leftrightarrow 4\overrightarrow {MO} + \left( {\overrightarrow {OA} + \overrightarrow {OB} } \right) + \left( {\overrightarrow {OC} + \overrightarrow {OD} } \right) = 4\overrightarrow {MO} \)
\( \Leftrightarrow 4\overrightarrow {MO} + \overrightarrow 0 + \overrightarrow 0 = 4\overrightarrow {MO} \\ \Leftrightarrow 4\overrightarrow {MO} = 4\overrightarrow {MO} \) (luôn đúng)
(vì O là giao điểm 2 đường chéo nên là trung điểm của AB, CD)
b) ABCD là hình bình hành nên ta có \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC} \)
Suy ra \(\)\(\overrightarrow {AB} + \overrightarrow {AC} + \overrightarrow {AD} = \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right) + \overrightarrow {AC} = \overrightarrow {AC} + \overrightarrow {AC} = 2\overrightarrow {AC} \) (đpcm)

VT=\(\overrightarrow{MB}\)+\(\overrightarrow{BA}\)+\(\overrightarrow{MD}\)+\(\overrightarrow{DC}\)
=(\(\overrightarrow{MB}\)+\(\overrightarrow{MD}\))+(\(\overrightarrow{BA}\)+\(\overrightarrow{DC}\))
=\(\overrightarrow{MB}\)+\(\overrightarrow{MD}\)+\(\overrightarrow{0}\) (vì \(\overrightarrow{BA}\) và \(\overrightarrow{DC}\) đối nhau)
=\(\overrightarrow{MB}\)+\(\overrightarrow{MD}\)(đpcm)

\(\overrightarrow{MA}-\overrightarrow{MB}=\overrightarrow{BM}+\overrightarrow{MA}=\overrightarrow{BA}\)(2)
\(\overrightarrow{MD}-\overrightarrow{MC}=\overrightarrow{CM}+\overrightarrow{MD}=\overrightarrow{CD}\)(1)
Vì ABCD là hình vuông nên \(\overrightarrow{BA}=\overrightarrow{CD}\left(3\right)\)
Từ (1),(2),(3) suy ra \(\overrightarrow{MA}-\overrightarrow{MB}=\overrightarrow{MD}-\overrightarrow{MC}\)
=>\(\overrightarrow{MA}+\overrightarrow{MC}=\overrightarrow{MB}+\overrightarrow{MD}\)

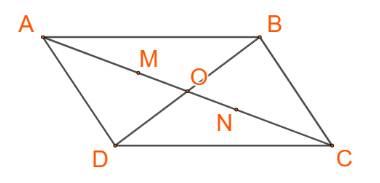
a) Áp dụng tính chất trọng tâm ta có: \(\overrightarrow {MA} + \overrightarrow {MD} + \overrightarrow {MB} = \overrightarrow 0 \)
Suy ra M là trọng tâm của tam giác ADB
Vậy M nằm trên đoạn thẳng AO sao cho \(AM = \frac{2}{3}AO\)
b) Tiếp tục áp dụng tính chất trọng tâm \(\overrightarrow {ND} + \overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \)
Suy ra N là trọng tâm của tam giác BCD
Vậy N nằm trên đoạn thẳng OD sao cho \(ON = \frac{1}{3}OD\)
c) Áp dụng tính chất trung điểm ta có: \(\overrightarrow {PM} + \overrightarrow {PN} = \overrightarrow 0 \)
Suy ra P là trung điểm của đoạn thẳng MN
Vậy điểm P trùng với điểm O.

Tham khảo:
a) Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \overrightarrow {MA} + \left( {\overrightarrow {MA} + \overrightarrow {AB} } \right) + 2\left( {\overrightarrow {MA} + \overrightarrow {AC} } \right) = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MA} + \overrightarrow {AB} + 2\overrightarrow {AC} = \overrightarrow 0 \\ \Leftrightarrow 4\overrightarrow {AM} = \overrightarrow {AB} + 2\overrightarrow {AC} \\ \Leftrightarrow \overrightarrow {AM} = \frac{1}{4}\overrightarrow {AB} + \frac{1}{2}\overrightarrow {AC} \end{array}\)
Trên cạnh AB, AC lấy điểm D, E sao cho \(AD = \frac{1}{4}AB;\;\,AE = \frac{1}{2}AC\)
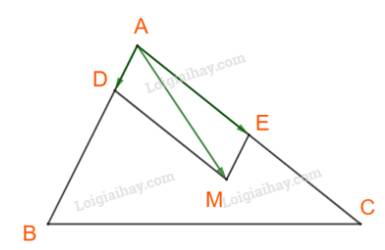
Khi đó \(\overrightarrow {AM} = \overrightarrow {AD} + \overrightarrow {AE} \) hay M là đỉnh thứ tư của hình bình hành AEMD.
b) Chứng minh rằng với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \)
Với mọi điểm O, ta có: \(\left\{ \begin{array}{l}\overrightarrow {OA} = \overrightarrow {OM} + \overrightarrow {MA} ;\;\\\overrightarrow {OB} = \overrightarrow {OM} + \overrightarrow {MB} ;\;\,\\\overrightarrow {OC} = \overrightarrow {OM} + \overrightarrow {MC} \end{array} \right.\)
\(\begin{array}{l} \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = \left( {\overrightarrow {OM} + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM} + \overrightarrow {MB} } \right) + 2\left( {\overrightarrow {OM} + \overrightarrow {MC} } \right)\\ = 4\overrightarrow {OM} + \left( {\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} } \right) = 4\overrightarrow {OM} + \overrightarrow 0 = 4\overrightarrow {OM} .\end{array}\)
Vậy với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \).
Tham khảo cách 2 câu a:
Cách 2:
Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \left( {\overrightarrow {MC} + \overrightarrow {CA} } \right) + \left( {\overrightarrow {MC} + \overrightarrow {CB} } \right) + 2\overrightarrow {MC} = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MC} + \overrightarrow {CA} + \overrightarrow {CB} = \overrightarrow 0 \\ \Leftrightarrow 4.\overrightarrow {CM} = \overrightarrow {CA} + \overrightarrow {CB} \end{array}\)
Gọi D là đỉnh thứ tư của hình bình hành ACBD.
Khi đó: \(\overrightarrow {CD} = \overrightarrow {CA} + \overrightarrow {CB} \)\( \Rightarrow 4.\overrightarrow {CM} = \overrightarrow {CD} \)
\( \Leftrightarrow \overrightarrow {CM} = \frac{1}{4}\overrightarrow {CD} \Leftrightarrow \overrightarrow {CM} = \frac{1}{2}\overrightarrow {CO} \)
Với O là tâm hình bình hành ACBD, cũng là trung điểm đoạn AB.
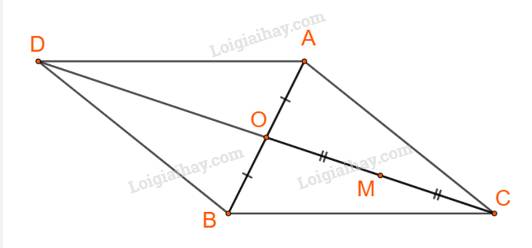
Vậy M là trung điểm của trung tuyến kẻ từ C của tam giác ABC.


Cách 1:
Do ABCD là hình bình hành nên \(\overrightarrow {AB} = \overrightarrow {DC} \)
\(\begin{array}{l} \Rightarrow \overrightarrow {AM} + \overrightarrow {MB} = \overrightarrow {DM} + \overrightarrow {MC} \\ \Leftrightarrow - \overrightarrow {MA} + \overrightarrow {MB} = - \overrightarrow {MD} + \overrightarrow {MC} \\ \Leftrightarrow \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \end{array}\)
Cách 2:
Ta có: \(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \Leftrightarrow \overrightarrow {MA} - \overrightarrow {MB} = \overrightarrow {MD} - \overrightarrow {MC} \) (*)
Áp dụng quy tắc hiệu ta có: \(\overrightarrow {MA} - \overrightarrow {MB} = \overrightarrow {BA} ;\;\;\overrightarrow {MD} - \overrightarrow {MC} = \overrightarrow {CD} \)
Do đó (*) \( \Leftrightarrow \overrightarrow {BA} = \overrightarrow {CD} \) (luôn đúng do ABCD là hình bình hành)
Cách 3:
Ta có:
\(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {BA} + \overrightarrow {MD} + \overrightarrow {DC} = \overrightarrow {MB} + \overrightarrow {MD} + \left( {\overrightarrow {BA} + \overrightarrow {DC} } \right)\)
Vì ABCD là hình bình hành nên \(\overrightarrow {AB} = \overrightarrow {DC} \)\( \Rightarrow - \overrightarrow {BA} = \overrightarrow {DC} \) hay \(\overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow 0 \)
\( \Rightarrow \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD} \) (đpcm)