\(2\sqrt{3}\) - \(\sqrt{4+x^2}\) =0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1,\(K=\sqrt{3-\sqrt{5}}+\sqrt{3+\sqrt{x}}\)
\(=\dfrac{1}{\sqrt{2}}\left(\sqrt{6-2\sqrt{5}}+\sqrt{6+2\sqrt{5}}\right)\)\(=\dfrac{1}{\sqrt{2}}\left(\sqrt{\left(\sqrt{5}-1\right)^2}+\sqrt{\left(\sqrt{5}+1\right)^2}\right)\)
\(=\dfrac{1}{\sqrt{2}}\left(\left|\sqrt{5}-1\right|+\sqrt{5}+1\right)\)\(=\dfrac{1}{\sqrt{2}}\left|\sqrt{5}-1+\sqrt{5}+1\right|=\dfrac{1}{\sqrt{2}}.2\sqrt{5}\)\(=\sqrt{10}\)
2, \(\sqrt{x-3}-2\sqrt{x^2-3x}=0\left(đk:x\ge3\right)\)
\(\Leftrightarrow\sqrt{x-3}\left(1-2\sqrt{x}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-3}=0\\1-2\sqrt{x}=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=\left(\dfrac{1}{2}\right)^2=\dfrac{1}{4}\left(ktm\right)\end{matrix}\right.\)
Vậy pt có nghiệm x=3
3, \(\dfrac{9x-7}{\sqrt{7x+5}}=\sqrt{7x+5}\left(đk:x>-\dfrac{5}{7}\right)\)
\(\Leftrightarrow9x-7=7x+5\)
\(\Leftrightarrow x=6\left(tm\right)\)
4, \(x-5\sqrt{x}+4=0\)(đk: \(x\ge0\))
\(\Leftrightarrow\left(\sqrt{x}-1\right)\left(\sqrt{x}-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=1\\\sqrt{x}=4\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=16\end{matrix}\right.\) (tm)
Vậy...
1) Bạn tự làm
2) ĐK: \(x\ge3\)
PT \(\Leftrightarrow\sqrt{x-3}\left(1-2\sqrt{x}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-3}=0\\2\sqrt{x}=1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=\dfrac{1}{4}\left(loại\right)\end{matrix}\right.\)
Vậy ...
3) ĐK: \(x>-\dfrac{5}{7}\)
PT \(\Rightarrow9x-7=7x+5\) \(\Leftrightarrow x=6\)
Vậy ...
4) ĐK: \(x\ge0\)
PT \(\Leftrightarrow x-4\sqrt{x}-\sqrt{x}+4=0\)
\(\Leftrightarrow\left(\sqrt{x}-4\right)\left(\sqrt{x}-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=4\\\sqrt{x}=1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=16\\x=1\end{matrix}\right.\)
Vậy ...

1. ĐKXĐ: $x\geq 4$
PT $\Leftrightarrow \sqrt{x-1}=5-\sqrt{x-4}$
$\Rightarrow x-1=25+x-4-10\sqrt{x-4}$
$\Leftrightarrow 22=10\sqrt{x-4}$
$\Leftrightarrow 2,2=\sqrt{x-4}$
$\Leftrightarrow 4,84=x-4\Leftrightarrow x=8,84$
(thỏa mãn)
2. ĐKXĐ: $x\geq 0$
PT $\Leftrightarrow (2x-2\sqrt{x})-(5\sqrt{x}-5)=0$
$\Leftrightarrow 2\sqrt{x}(\sqrt{x}-1)-5(\sqrt{x}-1)=0$
$\Leftrightarrow (\sqrt{x}-1)(2\sqrt{x}-5)=0$
$\Leftrightarrow \sqrt{x}-1=0$ hoặc $2\sqrt{x}-5=0$
$\Leftrightarrow x=1$ hoặc $x=\frac{25}{4}$ (tm)
3. ĐKXĐ: $x\geq 3$
Bình phương 2 vế thu được:
$3x-2+2\sqrt{(2x+1)(x-3)}=4x$
$\Leftrightarrow 2\sqrt{(2x+1)(x-3)}=x+2$
$\Leftrightarrow 4(2x+1)(x-3)=(x+2)^2$
$\Leftrightarrow 4(2x^2-5x-3)=x^2+4x+4$
$\Leftrightarrow 7x^2-24x-16=0$
$\Leftrightarrow (x-4)(7x+4)=0$
Do $x\geq 3$ nên $x=4$
Thử lại thấy thỏa mãn
Vậy $x=4$

1.
ĐKXĐ: \(x\ge3\)
Đặt \(\sqrt{x-3}=t\ge0\Rightarrow x=t^2+3\)
Pt trở thành:
\(t^2+3-7t-9=0\)
\(\Leftrightarrow t^2-7t-6=0\)
\(\Rightarrow\left[{}\begin{matrix}t=\dfrac{7-\sqrt{73}}{2}< 0\left(loại\right)\\t=\dfrac{7+\sqrt{73}}{2}\end{matrix}\right.\)
\(\Rightarrow\sqrt{x-3}=\dfrac{7+\sqrt{73}}{2}\)
\(\Rightarrow x=\dfrac{67+7\sqrt{73}}{2}\)
Nghiệm xấu quá, em nói giáo viên ra đề kiểm tra lại đề là \(x-7\sqrt{x-3}-9=0\) hay \(x-7\sqrt{x-3}+9=0\) nhé
2.
ĐKXĐ: \(x\ge2\)
\(\sqrt{x+3}+\sqrt{x-2}=5\)
\(\Leftrightarrow2x+1+2\sqrt{\left(x+3\right)\left(x-2\right)}=25\)
\(\Leftrightarrow\sqrt{x^2+x-6}=12-x\) (\(x\le12\))
\(\Rightarrow x^2+x-6=\left(12-x\right)^2\)
\(\Leftrightarrow x^2+x-6=144-24x+x^2\)
\(\Rightarrow x=6\)
Cách 2:
\(\Leftrightarrow\sqrt{x+3}-3+\sqrt{x-2}-2=0\)
\(\Leftrightarrow\dfrac{x-6}{\sqrt{x+3}+3}+\dfrac{x-6}{\sqrt{x-2}+2}=0\)
\(\Leftrightarrow\left(x-6\right)\left(\dfrac{1}{\sqrt{x+3}+3}+\dfrac{1}{\sqrt{x-2}+2}\right)=0\)
\(\Leftrightarrow x=6\)

2. ĐKXĐ: $x\geq 2$
PT \(\Rightarrow x+3=(5-\sqrt{x-2})^2\)
\(\Leftrightarrow x+3=25+x-2-10\sqrt{x-2}\)
\(\Leftrightarrow 20=10\sqrt{x-2}\Leftrightarrow x-2=4\Leftrightarrow x=6\)
Thử lại thấy thỏa mãn
Vậy $x=6$
3. ĐKXĐ: $x\geq -4$
PT $\Leftrightarrow \sqrt{(x+4)-4\sqrt{x+4}+4}=3$
$\Leftrightarrow \sqrt{(\sqrt{x+4}-2)^2}=3$
$\Leftrightarrow |\sqrt{x+4}-2|=3$
$\Leftrightarrow \sqrt{x+4}-2=\pm 3$. TH $\sqrt{x+4}-2=-3$ loại vì $\sqrt{x+4}-2\geq -2> -3$
Do đó: $\sqrt{x+4}-2=3$
$\Leftrightarrow \sqrt{x+4}=5$
$\Leftrightarrow x+4=25$
$\Leftrightarrow x=21$ (thỏa mãn)
Vậy $x=21$
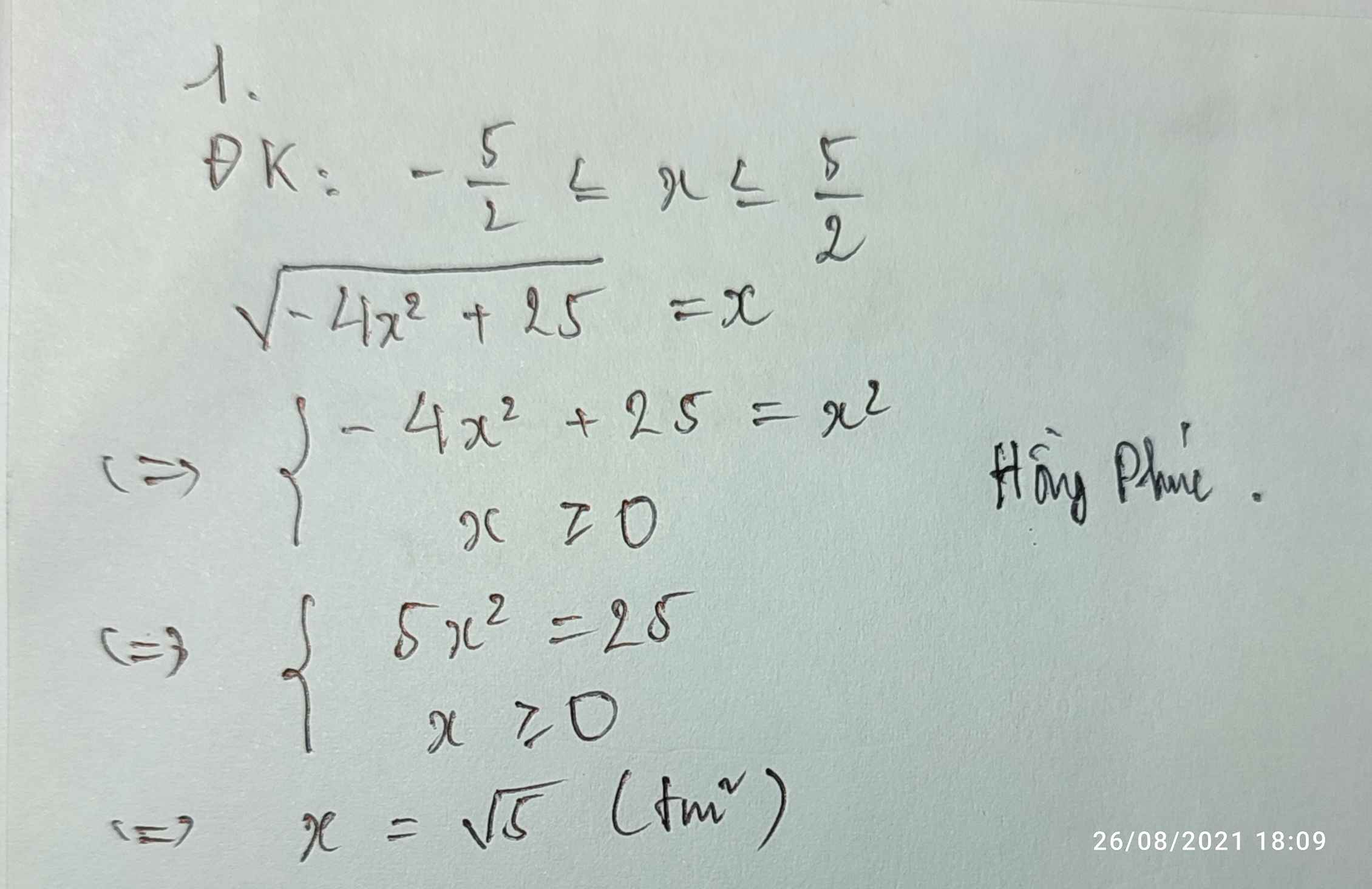
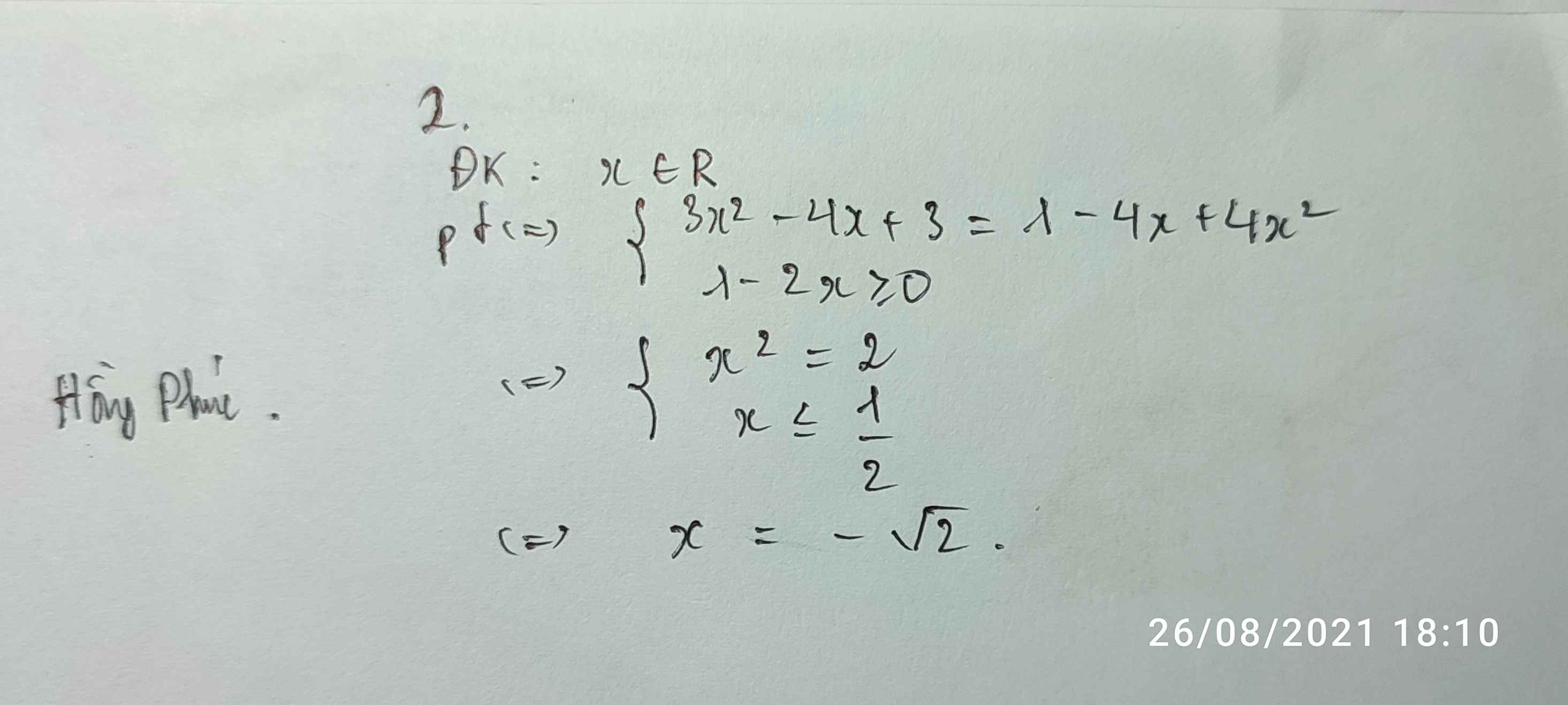
2\(\sqrt{3}\) - \(\sqrt{4+x^2}\) = 0 vì 4 + \(x^2\) ≥ 0 ∀ \(x\) vậy \(\sqrt{4+x^2}\) có nghĩa với ∀\(x\)
2\(\sqrt{3}\) - \(\sqrt{4+x^2}\) = 0
\(\sqrt{4+x^2}\) = 2\(\sqrt{3}\)
4 + \(x^2\) = 12
\(x^2\) = 12 - 4
\(x^2\) = 8
\(\left[{}\begin{matrix}x=-\sqrt{8}\\x=\sqrt{8}\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-2\sqrt{2}\\x=2\sqrt{2}\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-2\sqrt{2}\\x=2\sqrt{2}\end{matrix}\right.\)