Cho hai vecto \(\overrightarrow a ,\overrightarrow b \). Lấy một điểm A tùy ý.
a) Vẽ \(\overrightarrow {AB} = a\), \(\overrightarrow {BC} = b\)
b) Tổng của hai vecto \(\overrightarrow a \) và \(\overrightarrow b \)bằng vecto nào?
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
a) Đặt D, E lần lượt là điểm đầu và điểm cuối của vecto \(\overrightarrow a \).
Ta có: \(\overrightarrow {MA} = \overrightarrow a \)hay \(\overrightarrow {MA} = \overrightarrow {DE} \)
\( \Leftrightarrow MAED\) là hình bình hành.
Do đó A là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow a \)và điểm M.
Tương tự ta có:
B là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow b \)và điểm M.
Lại có: \(\overrightarrow {MC} = - \overrightarrow b = - \overrightarrow {MB} \) do đó \(MC = MB\) và hai vecto \(\overrightarrow {MB} ,\overrightarrow {MC} \) ngược hướng nhau.
Hay M là trung điểm đoạn thẳng BC.
b) Lấy N là đỉnh thứ tư của hình bình hành AMCN.
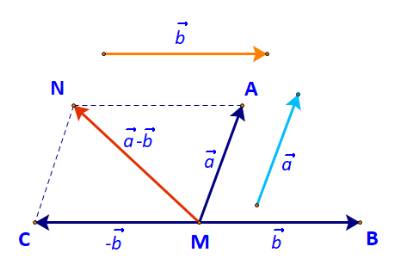
Khi đó ta có: \(\overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MN} \)
Mà: \(\overrightarrow {MA} = \overrightarrow a ;\;\overrightarrow {MC} = - \overrightarrow b \)
\( \Rightarrow \overrightarrow a + ( - \overrightarrow b ) = \overrightarrow {MN} \).
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
Cách 1:
Gọi O là giao điểm của AC và BD.
Ta có:
\(\begin{array}{l}\overrightarrow {AG} = \overrightarrow {AB} + \overrightarrow {BG} = \overrightarrow a + \overrightarrow {BG} ;\\\overrightarrow {CG} = \overrightarrow {CB} + \overrightarrow {BG} = \overrightarrow {DA} + \overrightarrow {BG} = - \overrightarrow b + \overrightarrow {BG} ;\end{array}\)(*)
Lại có: \(\overrightarrow {BD} =\overrightarrow {BA} + \overrightarrow {AD} = - \overrightarrow a + \overrightarrow b \).
\(\overrightarrow {BG} ,\overrightarrow {BD} \) cùng phương và \(\left| {\overrightarrow {BG} } \right| = \frac{2}{3}BO = \frac{1}{3}\left| {\overrightarrow {BD} } \right|\)
\( \Rightarrow \overrightarrow {BG} = \frac{1}{3}\overrightarrow {BD} = \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right)\)
Do đó (*) \( \Leftrightarrow \left\{ \begin{array}{l}\overrightarrow {AG} = \overrightarrow a + \overrightarrow {BG} = \overrightarrow a + \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\\\overrightarrow {CG} = -\overrightarrow b + \overrightarrow {BG} = -\overrightarrow b + \frac{1}{3}\left( { - \overrightarrow a + \overrightarrow b } \right) = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b ;\end{array} \right.\)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b .\)
24 tháng 9 2023
Cách 2:
Gọi AE, CF là các trung tuyến trong tam giác ABC.
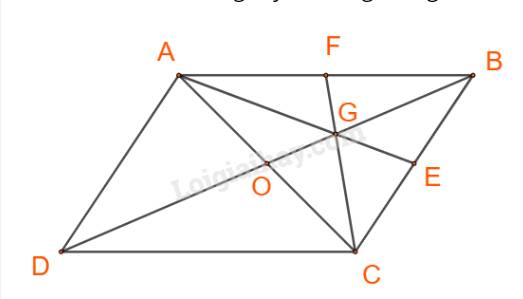
Ta có:
\(\overrightarrow {AG} = \frac{2}{3}\overrightarrow {AE} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\overrightarrow {AB} + \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right)} \right] \\= \frac{1}{3}\left( {2\overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b \)
\(\overrightarrow {CG} = \frac{2}{3}\overrightarrow {CF} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {CA} + \overrightarrow {CB} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\left( {\overrightarrow {CB} + \overrightarrow {CD} } \right) + \overrightarrow {CB} } \right] = \frac{1}{3}\left( {2\overrightarrow {CB} + \overrightarrow {CD} } \right) = \frac{1}{3}\left( { - 2\overrightarrow {AD} - \overrightarrow {AB} } \right) = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b \)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = - \frac{1}{3}\overrightarrow a - \frac{2}{3}\overrightarrow b .\)
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
Bước 1: Dựng hình bình hành có cạnh song song với giá của vecto \(\overrightarrow a ,\;\overrightarrow b \) và đường chéo là vecto \(\overrightarrow u ,\;\overrightarrow v \).
Ta dựng được hình hình hành ABCD và DEGH. Trong đó: DC và DE nằm trên giá của vecto \(\overrightarrow a \), DA và DH nằm trên giá của vecto \(\overrightarrow b \), còn vecto \(\overrightarrow u ,\;\overrightarrow v \) lần lượt là hai dường chéo.
Dễ thấy: \(\overrightarrow u = \overrightarrow {DA} + \overrightarrow {DC} ,\;\overrightarrow v = \overrightarrow {DH} + \overrightarrow {DE} \)
Mà \(\overrightarrow {DA} = 3\overrightarrow b ,\;\overrightarrow {DC} = \overrightarrow a \;,\;\overrightarrow {DH} = 3\overrightarrow b ,\;\overrightarrow {DE} = - 2\overrightarrow a .\)
\( \Rightarrow \overrightarrow u = 2\overrightarrow b + \overrightarrow a ,\;\,\overrightarrow v = 3\overrightarrow b - 2\overrightarrow a \)
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
\(\begin{array}{l}{ + \, (\overrightarrow a + \overrightarrow b )^2} = (\overrightarrow a + \overrightarrow b )(\overrightarrow a + \overrightarrow b )\\ = \overrightarrow a .(\overrightarrow a + \overrightarrow b ) + \overrightarrow b .(\overrightarrow a + \overrightarrow b ) \\= {\overrightarrow a ^2} + \overrightarrow a .\overrightarrow b + \overrightarrow b .\overrightarrow a + {\overrightarrow b ^2} \\= {\overrightarrow a ^2} + 2\overrightarrow a .\overrightarrow b + {\overrightarrow b ^2}.\\ + \, {(\overrightarrow a - \overrightarrow b )^2} =(\overrightarrow a - \overrightarrow b )(\overrightarrow a - \overrightarrow b )\\ = \overrightarrow a .(\overrightarrow a - \overrightarrow b ) - \overrightarrow b .(\overrightarrow a - \overrightarrow b ) \\= {\overrightarrow a ^2} - \overrightarrow a .\overrightarrow b - \overrightarrow b .\overrightarrow a + {\overrightarrow b ^2} \\= {\overrightarrow a ^2} - 2\overrightarrow a .\overrightarrow b + {\overrightarrow b ^2}. \\ + \, (\overrightarrow a - \overrightarrow b )(\overrightarrow a + \overrightarrow b ) \\= \overrightarrow a .(\overrightarrow a - \overrightarrow b ) + \overrightarrow b .(\overrightarrow a - \overrightarrow b ) \\= {\overrightarrow a ^2} - \overrightarrow a .\overrightarrow b + \overrightarrow b .\overrightarrow a - {\overrightarrow b ^2} \\= {\overrightarrow a ^2} - {\overrightarrow b ^2}.\end{array}\)
24 tháng 9 2023
Tham khảo:
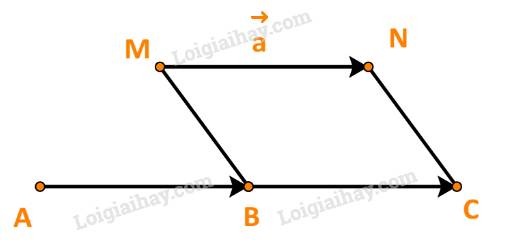
Gọi M, N lần lượt là điểm đầu và điểm cuối của vecto \(\overrightarrow a \).
Từ B, M, N ta dựng hình bình hành BMNC.
Khi đó: \(\overrightarrow {MN} = \overrightarrow {BC} \) hay \(\overrightarrow a = \overrightarrow {BC} \).
\( \Rightarrow \overrightarrow a + \overrightarrow a = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)
a) Vì \(\overrightarrow {AB} = \overrightarrow a = \overrightarrow {BC} \) nên A, B, C thẳng hàng và B là trung điểm của AC.
Vậy \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow {AB} \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow {AB} } \right|\)
b) Ta có: \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow {AB} \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow {AB} } \right|\)
Mà \(\overrightarrow {AB} = \overrightarrow a \) nên: \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow a \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow a } \right|\).
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
a) Ta có: \(\left\{ \begin{array}{l}AD//BC\\AD = BC\end{array} \right.\) (do tứ giác ABCD là hình bình hành)
\( \Rightarrow \overrightarrow {AD} = \overrightarrow {BC} \)
b) Ta có: \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)
14 tháng 1 2021
\(\overrightarrow{x}\) ⊥ \(\overrightarrow{y}\)
⇒ \(\left(\overrightarrow{a}+\overrightarrow{b}\right)\left(\overrightarrow{2a}-\overrightarrow{b}\right)=0\). Đặt \(\left|\overrightarrow{a}\right|=a;\left|\overrightarrow{b}\right|=b\)
⇒ 2a2 - \(\overrightarrow{a}.\overrightarrow{b}\) + 2\(\overrightarrow{a}.\overrightarrow{b}\) - b2 = 0
⇒ \(\overrightarrow{a}.\overrightarrow{b}\) = b2 - 2a2 = 4 - 4 = 0
⇒ \(\left(\overrightarrow{a};\overrightarrow{b}\right)=90^0\)
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
a) Nếu A, B, C thẳng hàng thì đường thẳng AB trùng đường thẳng AC, do đó hai vecto \(\overrightarrow {AB} ,\overrightarrow {AC} \) cùng phương.
b) Nếu hai vecto \(\overrightarrow {AB} ,\overrightarrow {AC} \) cùng phương thì đường thẳng AB trùng đường thẳng AC, do đó ba điểm A, B, C có thẳng hàng.
a) Gọi M, N lần lượt là điểm đầu và điểm cuối của vecto \(\overrightarrow a \).
Vì \(\overrightarrow a = \overrightarrow {AB} \Leftrightarrow \overrightarrow {MN} = \overrightarrow {AB} \) nên tứ giác MNBA là hình bình hành.
Nói cách khác B là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow a \) và điểm A.
Tương tự, C là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow b \) và điểm B.
b) Dễ thấy: tổng của hai vecto \(\overrightarrow {AB} \) và \(\overrightarrow {BC} \) là vecto \(\overrightarrow {AC} \).
Do đó tổng của hai vecto \(\overrightarrow a \) và \(\overrightarrow b \)bằng vecto \(\overrightarrow {AC} \).
Ta có viết: \(\overrightarrow a + \overrightarrow b = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)