tìm x,y \(\dfrac{x}{9}-\dfrac{3}{y}=\dfrac{1}{18}\)
Giải chi tiết giúp mik vs ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
26 tháng 2 2022
\(a.\dfrac{12}{3}=\dfrac{20}{5}=4\\ b.\dfrac{9}{-3}=\dfrac{-15}{5}=-3\)
26 tháng 2 2022
a, Xét \(\dfrac{x}{3}=4\Rightarrow x=12;\dfrac{20}{y}=4\Rightarrow y=\dfrac{20}{4}=5\)
b, \(\dfrac{9}{-x}=-3\Rightarrow-x=-3\Leftrightarrow x=3\)
\(\dfrac{y}{5}=-3\Rightarrow y=-15\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
\(\dfrac{x}{y+z+1}\) = \(\dfrac{y}{x+z+2}\) = \(\dfrac{z}{x+y-3}\) = \(x+y+z\)
Áp dụng tính chất dãy tỉ số bằng nhau ta có:
\(\dfrac{x}{y+z+1}\)=\(\dfrac{y}{x+z+2}\)=\(\dfrac{z}{x+y-3}\)=\(\dfrac{x+y+z}{y+z+1+x+z+2+x+y-3}\)
\(x+y+z\) = \(\dfrac{x+y+z}{2.\left(x+y+z\right)}\) = \(\dfrac{1}{2}\) (1)
\(\dfrac{x}{y+z+1}\) = \(\dfrac{1}{2}\) ⇒ 2\(x\) = y+z+1
⇒ 2\(x\) + \(x\) = \(x+y+z+1\) (2)
Thay (1) vào (2) ta có: 2\(x\) + \(x\) = \(\dfrac{1}{2}\) + 1
3\(x\) = \(\dfrac{3}{2}\) ⇒ \(x=\dfrac{1}{2}\)
\(\dfrac{y}{x+z+2}\) = \(\dfrac{1}{2}\) ⇒ 2y = \(x+z+2\) ⇒ 2y+y = \(x+y+z+2\) (3)
Thay (1) vào (3) ta có: 2y + y = \(\dfrac{1}{2}\) + 2
3y = \(\dfrac{5}{2}\) ⇒ y = \(\dfrac{5}{6}\)
Thay \(x=\dfrac{1}{2};y=\dfrac{5}{6}\) vào (1) ta có: \(\dfrac{1}{2}+\dfrac{5}{6}+z\) = \(\dfrac{1}{2}\)
\(\dfrac{5}{6}\) + z = 0 ⇒ z = - \(\dfrac{5}{6}\)
Kết luận: (\(x;y;z\)) = (\(\dfrac{1}{2}\); \(\dfrac{5}{6}\); - \(\dfrac{5}{6}\))
16 tháng 8 2023
TH1: x + y + z 0
Áp dụng tính chất dãy tỉ số bằng nhau, ta có:
= = =
= = =
⇒ x + y + z =
⇒ x + y = - z
x + z = - y
y + z = - x
Thay y + z + 1 = - x + 1
⇒ =
⇒ 2x = - x + 1
⇒ 2x + x = + 1
⇒ 3x =
⇒ x =
Thay x + z + 2 = - y + 2
⇒ =
⇒ 2y = - y + 2
⇒ 2y + y = + 2
⇒ 3y =
⇒ y =
Thay x + y - 3 = - z - 3
⇒ \frac{1}{2}$
⇒ 2z = - z - 3
⇒ 2z + z = - 3
⇒ 3z =
⇒ z =
TH2: x + y + z = 0
⇒ = = = 0
⇒ x = y = z = 0
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
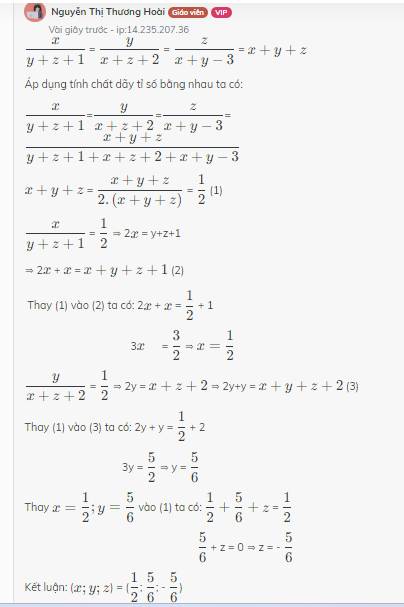
https://olm.vn/cau-hoi/tim-tat-ca-cac-so-xyz-biet-dfracxyz1dfracyxz2dfraczxy-3xyz-giair-chi-tiet-ho-e-vs-a.8297156371934
AM
6 tháng 2 2022
\(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{5}{6}\\\dfrac{\dfrac{2}{3}}{x}+\dfrac{\dfrac{2}{3}}{y}+\dfrac{\dfrac{8}{9}}{y}=1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{5}{6}\\\dfrac{\dfrac{2}{3}}{x}+\dfrac{\dfrac{14}{9}}{y}=1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{5}{6}\left(1\right)\\\dfrac{2}{3x}+\dfrac{14}{9y}=1\left(2\right)\end{matrix}\right.\)
Nhân cả hai vế (1) cho \(\dfrac{2}{3}\) ta có: \(\left\{{}\begin{matrix}\dfrac{2}{3x}+\dfrac{2}{3y}=\dfrac{5.2}{6.3}\\\dfrac{2}{3x}+\dfrac{14}{9y}=1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2}{3x}+\dfrac{2}{3y}=\dfrac{10}{18}\left(3\right)\\\dfrac{2}{3x}+\dfrac{14}{9y}=1\left(4\right)\end{matrix}\right.\)
Lấy (4) trừ (3) ta có:
\(\dfrac{14}{9y}-\dfrac{2}{3y}=1-\dfrac{10}{18}\)\(\Leftrightarrow\dfrac{8}{9y}=\dfrac{4}{9}\)\(\Leftrightarrow y=2\Rightarrow x=\dfrac{1}{\dfrac{5}{6}-\dfrac{1}{2}}=3\)
LM
2
23 tháng 2 2022
\(\Leftrightarrow3x+6+x^2-3x+2=9\)
\(\Leftrightarrow x^2+8=9\)
hay \(x\in\left\{1;-1\right\}\)
23 tháng 2 2022
ĐKXĐ:\(x\ne\pm2\)
\(\dfrac{3}{x-2}+\dfrac{x-1}{x+2}=\dfrac{9}{x^2-4}\\ \Leftrightarrow\dfrac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{\left(x-1\right)\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}-\dfrac{9}{\left(x-2\right)\left(x+2\right)}=0\\ \Leftrightarrow\dfrac{3\left(x+2\right)+\left(x-1\right)\left(x-2\right)-9}{\left(x-2\right)\left(x+2\right)}=0\\ \Rightarrow3\left(x+2\right)+\left(x-1\right)\left(x-2\right)-9=0\\ \Leftrightarrow3x+6+x^2-x-2x+2-9=0\\ \Leftrightarrow x^2-1=0\\ \Leftrightarrow\left(x-1\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\left(tm\right)\\x=1\left(tm\right)\end{matrix}\right.\)
LN
4
2
11 tháng 5 2022
`9/[x^2-4]=[x-1]/[x+2]+3/[x-2]` `ĐK: x \ne +-2`
`<=>9/[(x-2)(x+2)]=[(x-1)(x-2)+3(x+2)]/[(x-2)(x+2)]`
`=>9=x^2-2x-x+2+3x+6`
`<=>x^2=1`
`<=>x=+-1` (t/m)
Vậy `x=+-1`
11 tháng 5 2022
\(\dfrac{9}{x^2-4}=\dfrac{x-1}{x+2}+\dfrac{3}{x-2}\left(đkxđ:x\ne\pm2\right)\\ \Leftrightarrow\dfrac{9}{\left(x-2\right)\left(x+2\right)}=\dfrac{\left(x-1\right)\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\\ \Rightarrow9=x^2-3x+2+3x+6\\ \Leftrightarrow x^2=1\\ \Leftrightarrow x^2=\pm1\left(TM\right)\)
Vậy PT có tập nghiệm \(S=\left\{-1;1\right\}\)
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
24 tháng 4 2023
\(\dfrac{5}{x-3}+\dfrac{4}{x+3}=\dfrac{x-5}{x^2-9}\left(ĐKXĐ:x\ne\pm3\right)\\ \Leftrightarrow\dfrac{5\left(x+3\right)+4\left(x-3\right)}{x^2-9}=\dfrac{x-5}{x^2-9}\\ \Leftrightarrow5x+15+4x-12=x-5\\ \Leftrightarrow5x+4x-x=-5-15+12\\ \Leftrightarrow8x=-8\\ \Leftrightarrow x=-1\left(TM\right)\\ Vậy:S=\left\{-1\right\}\)
\(\dfrac{x}{9}-\dfrac{3}{y}=\dfrac{1}{18}\left(ĐKXĐ:y\ne0\right)\)
\(\Rightarrow\dfrac{xy-27}{9y}=\dfrac{1}{18}\)
\(\Rightarrow18\left(xy-27\right)=9y\)
\(\Rightarrow2\left(xy-27\right)=y\)
\(\Rightarrow2xy-54=y\)
\(\Rightarrow2xy-y=54\Rightarrow y\left(2x-1\right)=54\)
\(\Rightarrow y=\dfrac{54}{2x-1}\)
- Suy ra 54 chia hết cho 2x - 1
\(\Rightarrow2x-1\inƯ\left(54\right)\)
\(\Rightarrow2x-1\in\left\{1;-1;2;-2;3;-3;9;-9;27;-27\right\}\)
Cho 2x - 1 bằng từng giá trị ở trên, ta tìm được :
\(x\in\left\{1;0;\dfrac{3}{2};-\dfrac{1}{2};2;-1;5;-4;14;-13\right\}\). Mà x không có giá trị ngoài tập số nguyên.
\(\Rightarrow x\in\left\{-13;-4;-1;0;1;2;5;14\right\}\)
Thay các giá trị x trên vừa tìm được vào y :
\(\Rightarrow y\in\left\{54;-54;18;-18;6;-6;2;-2\right\}\)
Vậy : Các số x và y thỏa mãn đề bài là : \(\left(x;y\right)\in\left\{\left(1;54\right),\left(0;-54\right),\left(2;18\right),\left(-1;-18\right),\left(5;6\right),\left(-4;-6\right),\left(14;2\right),\left(-13;-2\right)\right\}\)
cảm ơn ạ