Cho tam giác $A B C$ biết $a=2 \sqrt{3}, b=2 \sqrt{2}, c=\sqrt{6}-\sqrt{2}$. Tính góc lớn nhất của tam giác.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(tanB=\sqrt{2}\Rightarrow\dfrac{AC}{AB}=\sqrt{2}\Rightarrow\dfrac{AC^2}{AB^2}=2\)
\(\Rightarrow\dfrac{AC^2}{AB^2}+1=3\Rightarrow\dfrac{AC^2+AB^2}{AB^2}=3\Rightarrow\dfrac{BC^2}{AB^2}=3\)
\(\Rightarrow\dfrac{AB}{BC}=\dfrac{1}{\sqrt{3}}\)
Mà \(sinC=\dfrac{AB}{BC}\Rightarrow sinC=\dfrac{1}{\sqrt{3}}\)
\(sin^2C+cos^2C=1\Rightarrow\dfrac{1}{3}+cos^2C=1\Rightarrow cosC=\dfrac{\sqrt{6}}{3}\)
\(tanC=\dfrac{sinC}{cosC}=\dfrac{\sqrt{2}}{2}\)
b.
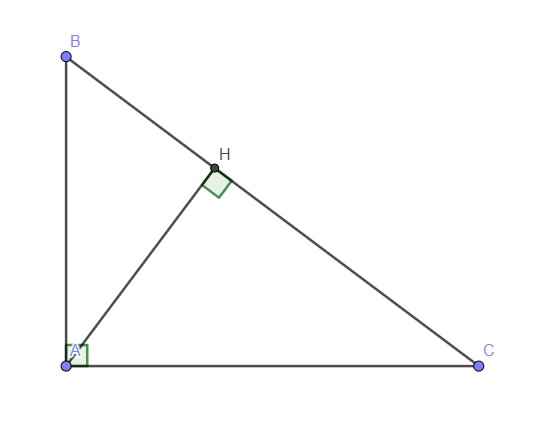
Trong tam giác vuông ACH:
\(sinC=\dfrac{AH}{AC}\Rightarrow AC=\dfrac{AH}{sinC}=\dfrac{2\sqrt{3}}{\dfrac{1}{\sqrt{3}}}=6\left(cm\right)\)
Trong tam giác vuông ABC:
\(tanB=\dfrac{AC}{AB}\Rightarrow AB=\dfrac{AC}{tanB}=\dfrac{6}{\sqrt{2}}=3\sqrt{2}\)
Áp dụng Pitago:
\(BC=\sqrt{AB^2+AC^2}=3\sqrt{6}\left(cm\right)\)

1.
Gọi $L$ là giao $BM, CN$ thì $L$ là trọng tâm tam giác $ABC$.
Áp dụng công thức đường trung tuyến:
$BM^2=\frac{c^2+a^2}{2}-\frac{b^2}{4}$
$CN^2=\frac{a^2+b^2}{2}-\frac{c^2}{4}$$BL^2=\frac{4}{9}BM^2=\frac{2}{9}(c^2+a^2)-\frac{1}{9}b^2$
$NL^2=\frac{1}{9}CN^2=\frac{1}{18}(a^2+b^2)-\frac{1}{36}c^2$
Theo cong thức Pitago:
$BN^2=BL^2+NL^2$
$\Rightarrow \frac{c^2}{4}=\frac{2}{9}(c^2+a^2)-\frac{1}{9}b^2+\frac{1}{18}(a^2+b^2)-\frac{1}{36}c^2$
$\Rightarrow $5a^2=b^2+c^2$ hay $b^2+c^2=45$
Áp dụng công thức cos:
$a^2=b^2+c^2-2bc\cos A=b^2+c^2-\sqrt{3}bc$
$\Rightarrow 9=45-\sqrt{3}bc\Rightarrow bc=12\sqrt{3}$
$S_{ABC}=\frac{1}{2}bc\sin A=\frac{1}{2}.12\sqrt{3}.\sin 30=3\sqrt{3}$
Đáp án A.
$b=
2.
\(R_{ABC}=\frac{abc}{4S_{ABC}}=\frac{3bc}{4S}=\frac{3.12\sqrt{3}}{4.3\sqrt{3}}=3\)
Đáp án B.