Tính giá trị của biểu thức 15x2 - 25x +18 biết 3x2 -5x + 6 =...
Đọc tiếp
Tính giá trị của biểu thức 15x2 - 25x +18 biết 3x2 -5x + 6 = 2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TQ
3
HH
0
9 tháng 4 2022
3x2-5x-6=0
(a=3 ; b = -5 ; c=-6)
Vì a=3 trái dấu với c=-6 nên phương trình co1v 2 nghiệm phân biệt
S= x1+x2=\(\dfrac{-b}{a}\)=\(\dfrac{-\left(-5\right)}{3}\)=\(\dfrac{5}{3}\)
P= x1*x2=\(\dfrac{c}{a}\)=\(\dfrac{-6}{3}\)=-2
A=\(\dfrac{x_1}{x_2}\)-\(\dfrac{2}{x_1^2}\)
A=\(\dfrac{x_1^3\cdot x_2}{x_1^2\cdot x_2^2}-\dfrac{x_2^2+2}{x_1^2\cdot x_2^2}\)
A=\(\dfrac{x_1^3\cdot x_2-x_2^2-2}{x_1^2\cdot x_2^2}\)
A=\(\dfrac{x^2_1-x^2_2-2}{x_1\cdot x_2}\)
A=\(\dfrac{\left(x_1+x_2\right)\cdot\left(x_1-x_2\right)-2}{x_1\cdot x_2}\)
A=\(\dfrac{S\cdot\sqrt{S2-4P}-2}{P}\)
(Giải thích thêm x1-x2 = \(\sqrt{S^2-4P}\) vì (x1-x2)^2=x1^2 - 2x1x2 + x2^2=(x1^2+x2^2) -2x1x2 = (S^2-2P)*2P=S^2-4P)
( Công thức x1^2+x2^2 = x1^2 + 2x1x2 + x2^2 -2x1x2 = (x1+x2)^2 - 2x1x2 = S^2 -2P)
Thế vào ta có :
A=\(\dfrac{\dfrac{5}{3}\cdot\sqrt{\left(\dfrac{5}{3}\right)^2-4\cdot\left(-2\right)}-2}{-2}\)
A= \(\dfrac{19-5\sqrt{97}}{18}\)
Vậy giá trị của biểu thức A=\(\dfrac{19-5\sqrt{97}}{18}\)
( chỗ tui không cần kết luận mà bài chỗ bác đẹp y như chỗ tui vậy )
NH
3
19 tháng 8 2023
\(E=-4x^2+x+1\)
\(\Rightarrow E=-4\left(x^2-\dfrac{x}{4}\right)+1\)
\(\Rightarrow E=-4\left(x^2-\dfrac{x}{4}+\dfrac{1}{64}\right)+1+\dfrac{1}{16}\)
\(\Rightarrow E=-4\left(x-\dfrac{1}{8}\right)^2+\dfrac{17}{16}\)
mà \(-4\left(x-\dfrac{1}{8}\right)^2\le0,\forall x\)
\(\Rightarrow E=-4\left(x-\dfrac{1}{8}\right)^2+\dfrac{17}{16}\le\dfrac{17}{16}\)
\(\Rightarrow GTLN\left(E\right)=\dfrac{17}{16}\left(tạix=\dfrac{1}{8}\right)\)
19 tháng 8 2023
\(F=5x-3x^2+6\)
\(\Rightarrow F=-3\left(x^2-\dfrac{5x}{3}\right)+6\)
\(\Rightarrow F=-3\left(x^2-\dfrac{5x}{3}+\dfrac{25}{36}\right)+6+\dfrac{25}{12}\)
\(\Rightarrow F=-3\left(x-\dfrac{5}{6}\right)^2+\dfrac{97}{12}\)
mà \(-3\left(x-\dfrac{5}{6}\right)^2\le0,\forall x\)
\(\Rightarrow F=-3\left(x-\dfrac{5}{6}\right)^2+\dfrac{97}{12}\le\dfrac{97}{12}\)
\(\Rightarrow GTLN\left(F\right)=\dfrac{97}{12}\left(tạix=\dfrac{5}{6}\right)\)
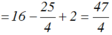
Đề bài sai kìa.