3x3 - 48x = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
4 tháng 1 2022
Bài 2:
a: \(\Leftrightarrow2x^2-10x-3x-2x^2=26\)
=>-13x=26
hay x=-2
b: \(\Leftrightarrow\left(x-1\right)\left(5x-1\right)=0\)
hay \(x\in\left\{1;\dfrac{1}{5}\right\}\)
c: \(\Leftrightarrow\left(x+5\right)\left(2-x\right)=0\)
hay \(x\in\left\{-5;2\right\}\)
HT
1
10 tháng 9 2018
\(3x^3-48x=0\)
\(3x\cdot\left(x^2-16\right)=0\)
\(\Rightarrow\orbr{\begin{cases}3x=0\\x^2-16=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=0\\x=\left\{\pm4\right\}\end{cases}}\)
Vậy,............
CM
17 tháng 6 2019
Ta có
x 3 – 12 x 2 + 48 x – 64 = 0 ⇔ x 3 – 3 . x 2 . 4 + 3 . x . 4 2 – 4 3 = 0 ⇔ ( x – 4 ) 3 = 0
ó x – 4 = 0 ó x = 4
Vậy x = 4
Đáp án cần chọn là: B
13 tháng 4 2018
\(\Delta=b^2-4ac=\left(-48\right)^2-4.1.\left(-25\right)=2400>0\)
do đó pt có 2 nghiệm phân biệt là:
\(•x_1=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{48-\sqrt{2400}}{2}=24-10\sqrt{6}\\ •x_2=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{48+\sqrt{2400}}{2}=24+10\sqrt{6}\)
ND
13 tháng 4 2018
\(x^2-48x-25=0\)
\(\Leftrightarrow x^2-2.x.24+24^2-601=0\)
\(\Leftrightarrow\left(x-24\right)^2-601=0\)
\(\Leftrightarrow\left(x-24\right)^2=601\)
\(\Leftrightarrow x-24=\sqrt{601}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-24=\sqrt{601}\\x-24=-\sqrt{601}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=24+\sqrt{601}\\x=24-\sqrt{601}\end{matrix}\right.\)
27 tháng 7 2021
\(3x^3-75x=0\Leftrightarrow3x\left(x^2-25\right)=0\Leftrightarrow3x\left(x-5\right)\left(x+5\right)=0\Leftrightarrow x=0;x=-5;x=5\)
4 tháng 10 2021
\(x^3+3x^2+2x=0\)
\(\Leftrightarrow x\left(x^2+3x+2\right)=0\)
\(\Leftrightarrow x\left(x+1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-1\\x=-2\end{matrix}\right.\)
T
26 tháng 8 2023
\(x^4+3x^3-x-3=0\)
\(\Leftrightarrow x^3\left(x+3\right)-\left(x+3\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^3-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x^3-1=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=1\end{matrix}\right.\)
Vậy \(x\in\left\{-3;1\right\}\)
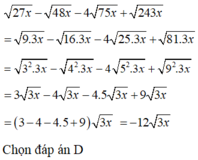
3x3 - 48x = 0
=> 3x( x2 - 16) = 0
=> x = 0 hoặc x2 -16 = 0
x2 - 16 = 0 => x2 = 16 => x = 4 hoặc x =-4