30+5x-12=0 giải phương trình
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HV
1
23 tháng 3 2020
\(\hept{\begin{cases}5x+5y=30\\y+5z=12\\3x+5z=22\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=6-y\\y+5z=12\\3x+5z=22\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}y+5z=12\\3\left(6-y\right)+5z=22\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}y+5z=12\\-3y+5z=4\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}y+5z=12\\3y-5z=-4\end{cases}}\)
\(\Rightarrow4y=8\Rightarrow y=2\)
Thay giá trị của y vào phương trình: -3y + 5z = 4
\(-3\times2+5z=4\)
\(\Rightarrow z=2\)
Thế giá trị của y vào phương trình: x = 6 - y
\(\Rightarrow x=4\)
23 tháng 4 2021
Đặt t=x^2>=0(1)
(1)<=>12t^2-5t+30=0
∆=(-5)^2-4.12.30=-1415<0
Vậy phương trình vô nghiệm
9 tháng 2 2021
Ta có : \(\dfrac{5x-150}{50}+\dfrac{5x-102}{49}+\dfrac{5x-56}{48}+\dfrac{5x-12}{47}+\dfrac{5x-660}{46}=0\)
\(\Leftrightarrow\dfrac{5x-150}{50}-1+\dfrac{5x-102}{49}-2+\dfrac{5x-56}{48}-3+\dfrac{5x-12}{47}-4+\dfrac{5x-660}{46}+10=0\)
\(\Leftrightarrow\dfrac{5x-200}{50}+\dfrac{5x-200}{49}+\dfrac{5x-200}{48}+\dfrac{5x-200}{47}+\dfrac{5x-200}{46}=0\)
\(\Leftrightarrow\left(5x-200\right)\left(\dfrac{1}{50}+\dfrac{1}{49}+\dfrac{1}{48}+\dfrac{1}{47}+\dfrac{1}{46}\right)=0\)
\(\Leftrightarrow5x-200=0\)
\(\Leftrightarrow x=40\)
Vậy ...
9 tháng 2 2021
Ta có: \(\dfrac{5x-150}{50}+\dfrac{5x-102}{49}+\dfrac{5x-56}{48}+\dfrac{5x-12}{47}+\dfrac{5x-660}{46}=0\)
\(\Leftrightarrow\dfrac{5x-150}{50}-1+\dfrac{5x-102}{49}-2+\dfrac{5x-56}{48}-3+\dfrac{5x-12}{47}-4+\dfrac{5x-660}{46}+10=0\)
\(\Leftrightarrow\dfrac{5x-200}{50}+\dfrac{5x-200}{49}+\dfrac{5x-200}{48}+\dfrac{5x-200}{47}+\dfrac{5x-200}{46}=0\)
\(\Leftrightarrow\left(5x-200\right)\left(\dfrac{1}{50}+\dfrac{1}{49}+\dfrac{1}{48}+\dfrac{1}{47}+\dfrac{1}{46}\right)=0\)
mà \(\dfrac{1}{50}+\dfrac{1}{49}+\dfrac{1}{48}+\dfrac{1}{47}+\dfrac{1}{46}>0\)
nên 5x-200=0
\(\Leftrightarrow5x=200\)
hay x=40
Vậy: S={40}
25 tháng 2 2018
\(x^4+2x^3+5x^2+4x-12=0\)
\(\Leftrightarrow\)\(x^4-x^3+3x^3-3x^2+8x^2-8x+12x-12=0\)
\(\Leftrightarrow\)\(x^3\left(x-1\right)+3x^2\left(x-1\right)+8x\left(x-1\right)+12\left(x-1\right)=0\)
\(\Leftrightarrow\)\(\left(x^3+3x^2+8x+12\right)\left(x-1\right)=0\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x-1=0\\x^3+3x^2+8x+12=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=1\\x^3+2x^2+x^2+2x+6x+12=0\end{cases}}}\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=1\\\left(x+2\right)\left(x^2+x+6\right)=0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\x=-2\\x^2+x+6=0\left(1\right)\end{cases}}\)
Giải pt ( 1 ) \(x^2+\frac{1}{2}x.2+\frac{1}{4}+\frac{23}{4}=0\)
\(\Leftrightarrow\)\(\left(x+\frac{1}{2}\right)^2+\frac{23}{4}=0\)suy ra pt ( 1 ) vô nghiệm
Vậy pt có 2 nghiệm là x = 1 ; x = -2
25 tháng 2 2018
x4 + 2x3 + 5x2 + 4x - 10 = 0
x4 - x3 + 3x3 - 3x2 + 8x2 - 8x + 12x - 12 = 0
<=> x3(x - 1) + 3x2(x - 1) + 8x(x - 1) + 12(x - 1) = 0
\(\Leftrightarrow\orbr{\begin{cases}x-1=0\\x^3+3x^2+8x+12=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1\\x^3+2x^2+x^2+2x+6x+10=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1\\\left(x+2\right)+\left(x^2+x+6\right)=0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=1\\x=-2\\x^2+x+6=0\left(1\right)\end{cases}}\)
Giải (1) \(x^2+\frac{1}{2}x.2+\frac{1}{4}+\frac{23}{4}=0\)
\(\Leftrightarrow\left(x+\frac{1}{2}\right)^2+\frac{23}{4}>0\Rightarrow\text{PT}\left(1\right)\)Vô nghiệm
=> PT có 2 nghiệm: \(\hept{\begin{cases}x=1\\x=-2\end{cases}}\)
PA
0
1 tháng 1 2018
\(pt\Leftrightarrow\frac{5x-150}{50}+\frac{5x-102}{49}+\frac{5x-56}{48}+\frac{5x-12}{47}+\frac{5x-16}{46}-14=0\)
\(\Leftrightarrow\frac{5x-150}{50}-1+\frac{5x-102}{49}-2+\frac{5x-56}{48}-3+\frac{5x-12}{47}-4+\frac{5x-16}{46}-4=0\)
\(\Leftrightarrow\frac{5x-200}{50}+\frac{5x-200}{49}+\frac{5x-200}{48}+\frac{5x-200}{47}+\frac{5x-200}{46}=0\)
\(\Leftrightarrow\left(5x-200\right)\left(\frac{1}{50}+\frac{1}{49}+\frac{1}{48}+\frac{1}{47}+\frac{1}{46}\right)=0\)
Do \(\frac{1}{50}+\frac{1}{49}+\frac{1}{48}+\frac{1}{47}+\frac{1}{46}\ne0\) nên \(5x-200=0\Rightarrow x=\frac{200}{5}=40\)
Vậy x= 40
KT
1 tháng 1 2018
\(\frac{5x-150}{50}+\frac{5x-102}{49}+\frac{5x-56}{48}+\frac{5x-12}{47}+\frac{5x-660}{46}=0\)
\(\Leftrightarrow\)\(\left(\frac{5x-150}{50}-1\right)+\left(\frac{5x-102}{49}-2\right)+\left(\frac{5x-56}{48}-3\right)+\left(\frac{5x-12}{47}-4\right)+\left(\frac{5x-660}{46}+10\right)=0\)
\(\Leftrightarrow\)\(\frac{5x-200}{50}+\frac{5x-200}{49}+\frac{5x-200}{48}+\frac{5x-200}{47}+\frac{5x-200}{46}=0\)
\(\Leftrightarrow\)\(\left(5x-200\right)\left(\frac{1}{50}+\frac{1}{49}+\frac{1}{48}+\frac{1}{47}+\frac{1}{46}\right)=0\)
\(\Leftrightarrow\)\(5x-200=0\)
\(\Leftrightarrow\)\(5x=200\)
\(\Leftrightarrow\)\(x=40\)
Vậy x = 40
CM
20 tháng 2 2018
a)
3 x 2 − 5 x + 1 x 2 − 4 = 0 ⇔ 3 x 2 − 5 x + 1 = 0
hoặc x 2 – 4 = 0 ( 2 )
+ Giải (1): 3 x 2 – 5 x + 1 = 0
Có a = 3; b = -5; c = 1 ⇒ Δ = ( - 5 ) 2 – 4 . 3 = 13 > 0
Phương trình có hai nghiệm: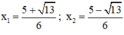 
+ Giải (2): x 2 – 4 = 0 ⇔ x 2 = 4 ⇔ x = 2 hoặc x = -2.
Vậy phương trình có tập nghiệm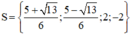 
b)
2 x 2 + x − 4 2 − ( 2 x − 1 ) 2 = 0 ⇔ 2 x 2 + x − 4 − 2 x + 1 2 x 2 + x − 4 + 2 x − 1 = 0 ⇔ 2 x 2 − x − 3 2 x 2 + 3 x − 5 = 0 ⇔ 2 x 2 − x − 3 = 0 ( 1 )
hoặc 2 x 2 + 3 x – 5 = 0 ( 2 )
+ Giải (1): 2 x 2 – x – 3 = 0
Có a = 2; b = -1; c = -3 ⇒ a – b + c = 0
⇒ Phương trình có hai nghiệm x = -1 và x = -c/a = 3/2.
+ Giải (2): 2 x 2 + 3 x – 5 = 0
Có a = 2; b = 3; c = -5 ⇒ a + b + c = 0
⇒ Phương trình có hai nghiệm x = 1 và x = c/a = -5/2.
Vậy phương trình có tập nghiệm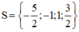 
NN
1
12 tháng 2 2023
\(\Leftrightarrow\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}+...+\dfrac{1}{\left(x+5\right)\left(x+6\right)}=\dfrac{1}{8}\)
=>\(\dfrac{1}{x+2}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+4}+...+\dfrac{1}{x+5}-\dfrac{1}{x+6}=\dfrac{1}{8}\)
=>1/x+2-1/x+6=1/8
=>\(\dfrac{x+6-x-2}{\left(x+2\right)\left(x+6\right)}=\dfrac{1}{8}\)
=>x^2+8x+12=32
=>x^2+8x-20=0
=>(x+10)(x-2)=0
=>x=-10 hoặc x=2
30 + 5x - 12 = 0
=> 30 + 5x = 0 + 12 = 12
=> 5x = 12 - 30 = -18
=> x = -18 / 5 = -3.6
Vậy x = -3.6
Võ Thị Mỹ Duyên đề bảo giải phương trình chứ đâu dễ như zậy đâu