Giải phương trình
a) 2x4-7x2+4=0
b) (x2-9)(x2+7x+6)=0
c) 5x4+2x2–16=10–x2
d)√x-5 =x-7
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) 3 x 2 − 7 x − 10 ⋅ 2 x 2 + ( 1 − 5 ) x + 5 − 3 = 0
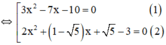
+ Giải (1):
3 x 2 – 7 x – 10 = 0
Có a = 3; b = -7; c = -10
⇒ a – b + c = 0
⇒ (1) có hai nghiệm x 1 = - 1 v à x 2 = - c / a = 10 / 3 .
+ Giải (2):
2 x 2 + ( 1 - √ 5 ) x + √ 5 - 3 = 0
Có a = 2; b = 1 - √5; c = √5 - 3
⇒ a + b + c = 0
⇒ (2) có hai nghiệm:
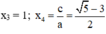
Vậy phương trình có tập nghiệm 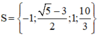
b)
x 3 + 3 x 2 - 2 x - 6 = 0 ⇔ x 3 + 3 x 2 - ( 2 x + 6 ) = 0 ⇔ x 2 ( x + 3 ) - 2 ( x + 3 ) = 0 ⇔ x 2 - 2 ( x + 3 ) = 0
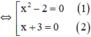
+ Giải (1): x 2 – 2 = 0 ⇔ x 2 = 2 ⇔ x = √2 hoặc x = -√2.
+ Giải (2): x + 3 = 0 ⇔ x = -3.
Vậy phương trình có tập nghiệm S = {-3; -√2; √2}
c)
x 2 − 1 ( 0 , 6 x + 1 ) = 0 , 6 x 2 + x ⇔ x 2 − 1 ( 0 , 6 x + 1 ) = x ⋅ ( 0 , 6 x + 1 ) ⇔ x 2 − 1 ( 0 , 6 x + 1 ) − x ( 0 , 6 x + 1 ) = 0 ⇔ ( 0 , 6 x + 1 ) x 2 − 1 − x = 0
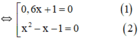
+ Giải (1): 0,6x + 1 = 0 ⇔ 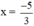
+ Giải (2):
x 2 – x – 1 = 0
Có a = 1; b = -1; c = -1
⇒ Δ = ( - 1 ) 2 – 4 . 1 . ( - 1 ) = 5 > 0
⇒ (2) có hai nghiệm 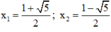
Vậy phương trình có tập nghiệm 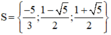
d)
x 2 + 2 x − 5 2 = x 2 − x + 5 2 ⇔ x 2 + 2 x − 5 2 − x 2 − x + 5 2 = 0 ⇔ x 2 + 2 x − 5 − x 2 − x + 5 ⋅ x 2 + 2 x − 5 + x 2 − x + 5 = 0 ⇔...

Bài 2:
a: =>2x^2-4x+1=x^2+x+5
=>x^2-5x-4=0
=>\(x=\dfrac{5\pm\sqrt{41}}{2}\)
b: =>11x^2-14x-12=3x^2+4x-7
=>8x^2-18x-5=0
=>x=5/2 hoặc x=-1/4

\(a.x^2-4x+4=0\)
\(\left(x-2\right)^2=0\)
=>x=2
b) \(2x^2-x=0\)
\(x\left(2x-1\right)=0\)
=> \(\left[{}\begin{matrix}x=0\\x=\dfrac{1}{2}\end{matrix}\right.\)
c) \(x^2-5x+6=0\)
\(x^2-2x-3x+6=0\)
\(\left(x-2\right)\left(x-3\right)=0\)
=> \(\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)
d) \(x^2+y^2=0\)
Vì \(x^2,y^2\ge0\forall x,y\)
=>x=y=0
e) \(x^2+6x+10=0\)
\(\left(x+3\right)^2+1=0\)
Vì \(\left(x+3\right)^2\ge0\forall x\)
=> VT>0 \(\forall x\)
=> phương trình vô nghiệm

\(a,\Leftrightarrow2x^2+10x-2x^2=12\Leftrightarrow x=\dfrac{12}{10}=\dfrac{6}{5}\\ b,\Leftrightarrow\left(5-2x-4\right)\left(5-2x+4\right)=0\\ \Leftrightarrow\left(1-2x\right)\left(9-2x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\dfrac{9}{2}\end{matrix}\right.\\ c,\Leftrightarrow3x^2-3x^2+6x=36\Leftrightarrow x=6\\ d,\Leftrightarrow2\left(x+5\right)-x\left(x+5\right)=0\\ \Leftrightarrow\left(2-x\right)\left(x+5\right)=0\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-5\end{matrix}\right.\\ e,\Leftrightarrow4x^2-4x+1-4x^2+196=0\\ \Leftrightarrow-4x=-197\Leftrightarrow x=\dfrac{197}{4}\)
\(f,\Leftrightarrow x^2+8x+16-x^2+1=16\Leftrightarrow8x=-1\Leftrightarrow x=-\dfrac{1}{8}\\ g,Sửa:\left(3x+1\right)^2-\left(x+1\right)^2=0\\ \Leftrightarrow\left(3x+1-x-1\right)\left(3x+1+x+1\right)=0\\ \Leftrightarrow2x\left(4x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{1}{2}\end{matrix}\right.\\ h,\Leftrightarrow x^2+8x-x-8=0\\ \Leftrightarrow\left(x+8\right)\left(x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-8\end{matrix}\right.\\ i,\Leftrightarrow2x^2-13x+15=0\\ \Leftrightarrow2x^2+2x-15x-15=0\\ \Leftrightarrow\left(x+1\right)\left(2x-15\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\dfrac{15}{2}\end{matrix}\right.\)

a)
3 · x 2 + x 2 - 2 x 2 + x - 1 = 0 ( 1 )
Đặt t = x 2 + x ,
Khi đó (1) trở thành : 3 t 2 – 2 t – 1 = 0 ( 2 )
Giải (2) : Có a = 3 ; b = -2 ; c = -1
⇒ a + b + c = 0
⇒ (2) có hai nghiệm t 1 = 1 ; t 2 = c / a = - 1 / 3 .
+ Với t = 1 ⇒ x 2 + x = 1 ⇔ x 2 + x – 1 = 0 ( * )
Có a = 1; b = 1; c = -1 ⇒ Δ = 1 2 – 4 . 1 . ( - 1 ) = 5 > 0
(*) có hai nghiệm
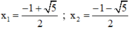
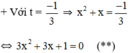
Có a = 3; b = 3; c = 1 ⇒ Δ = 3 2 – 4 . 3 . 1 = - 3 < 0
⇒ (**) vô nghiệm.
Vậy phương trình (1) có tập nghiệm 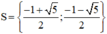
b)
x 2 − 4 x + 2 2 + x 2 − 4 x − 4 = 0 ⇔ x 2 − 4 x + 2 2 + x 2 − 4 x + 2 − 6 = 0 ( 1 )
Đặt x 2 – 4 x + 2 = t ,
Khi đó (1) trở thành: t 2 + t – 6 = 0 ( 2 )
Giải (2): Có a = 1; b = 1; c = -6
⇒ Δ = 1 2 – 4 . 1 . ( - 6 ) = 25 > 0
⇒ (2) có hai nghiệm
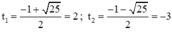
+ Với t = 2 ⇒ x 2 – 4 x + 2 = 2
⇔ x 2 – 4 x = 0
⇔ x(x – 4) = 0
⇔ x = 0 hoặc x = 4.
+ Với t = -3 ⇒ x 2 – 4 x + 2 = - 3
⇔ x2 – 4x + 5 = 0 (*)
Có a = 1; b = -4; c = 5 ⇒ Δ ’ = ( - 2 ) 2 – 1 . 5 = - 1 < 0
⇒ (*) vô nghiệm.
Vậy phương trình ban đầu có tập nghiệm S = {0; 4}.
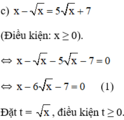
Khi đó (1) trở thành: t 2 – 6 t – 7 = 0 ( 2 )
Giải (2): Có a = 1; b = -6; c = -7
⇒ a – b + c = 0
⇒ (2) có nghiệm t 1 = - 1 ; t 2 = - c / a = 7 .
Đối chiếu điều kiện chỉ có nghiệm t = 7 thỏa mãn.
+ Với t = 7 ⇒ √x = 7 ⇔ x = 49 (thỏa mãn).
Vậy phương trình đã cho có nghiệm x = 49.
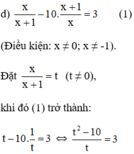
⇔ t 2 – 10 = 3 t ⇔ t 2 – 3 t – 10 = 0 ( 2 )
Giải (2): Có a = 1; b = -3; c = -10
⇒ Δ = ( - 3 ) 2 - 4 . 1 . ( - 10 ) = 49 > 0
⇒ (2) có hai nghiệm:
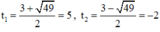
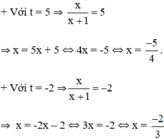
Cả hai nghiệm đều thỏa mãn điều kiện xác định.
Vậy phương trình đã cho có tập nghiệm 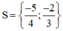

\(a,x^3+x^2+x+1=0\\ \Rightarrow x^2\left(x+1\right)+\left(x+1\right)=0\\ \Rightarrow\left(x^2+1\right)\left(x+1\right)=0\\ \Rightarrow\left[{}\begin{matrix}x^2=-1\left(vô.lí\right)\\x=-1\end{matrix}\right.\)
Vậy pt có tập nghiệm \(S=\left\{-1\right\}\)
\(b,x^3+x^2-x-1=0\\ \Rightarrow x^2\left(x+1\right)-\left(x+1\right)=0\\ \Rightarrow\left(x^2-1\right)\left(x+1\right)=0\\ \Rightarrow\left(x-1\right)\left(x+1\right)^2=0\\ \Rightarrow\left[{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)
Vậy pt có tập nghiệm \(S=\left\{-1;1\right\}\)
\(c,\left(x+1\right)^2\left(x+2\right)+\left(x+1\right)^2\left(x-2\right)=-24\\ \Rightarrow\left(x+1\right)^2\left(x+2+x-2\right)=-24\\ \Rightarrow2x\left(x^2+2x+1\right)=-24\\ \Rightarrow x^3+2x^2+x+12=0\\ \Rightarrow\left(x^3+3x^2\right)-\left(x^2+3x\right)+\left(4x+12\right)=0\\ \Rightarrow x^2\left(x+3\right)-x\left(x+3\right)+4\left(x+3\right)=0\\ \Rightarrow\left(x^2-x+4\right)\left(x+3\right)=0\\ \Rightarrow\left[{}\begin{matrix}\left(x-\dfrac{1}{2}\right)^2+\dfrac{15}{4}=0\left(vô.lí\right)\\x=-3\end{matrix}\right.\)
Vậy pt có tập nghiệm \(S=\left\{-3\right\}\)

Bài 3:
\(\Leftrightarrow x^3+64-x^3+25x=264\)
hay x=8
\(1,C=6x^2+23x-55-6x^2-23x-21=-76\\ 2,=\left(2x^4-x^2+2x^3-x-6x^2+6-3\right):\left(2x^2-1\right)\\ =\left[\left(2x^2-1\right)\left(x^2+x-6\right)-3\right]:\left(2x^2-1\right)\\ =x^2+x-6\left(dư.-3\right)\\ 3,\Leftrightarrow x^3+64-x^3+25x=264\\ \Leftrightarrow25x=200\Leftrightarrow x=8\)
giup mình voi huhu
a) Đặt \(x^2=a\left(a\ge0\right)\)
Ta có: \(2x^4-7x^2+4=0\)
Suy ra: \(2a^2-7a+4=0\)
\(\Delta=49-4\cdot2\cdot4=49-32=17\)
Vì \(\Delta>0\) nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}a_1=\dfrac{7-\sqrt{17}}{4}\left(nhận\right)\\a_2=\dfrac{-7+\sqrt{17}}{4}\left(loại\right)\end{matrix}\right.\)
Suy ra: \(x^2=\dfrac{7-\sqrt{17}}{4}\)
\(\Leftrightarrow x=\pm\dfrac{\sqrt{7-\sqrt{17}}}{2}\)
Vậy: \(S=\left\{\dfrac{\sqrt{7-\sqrt{17}}}{2};-\dfrac{\sqrt{7-\sqrt{17}}}{2}\right\}\)