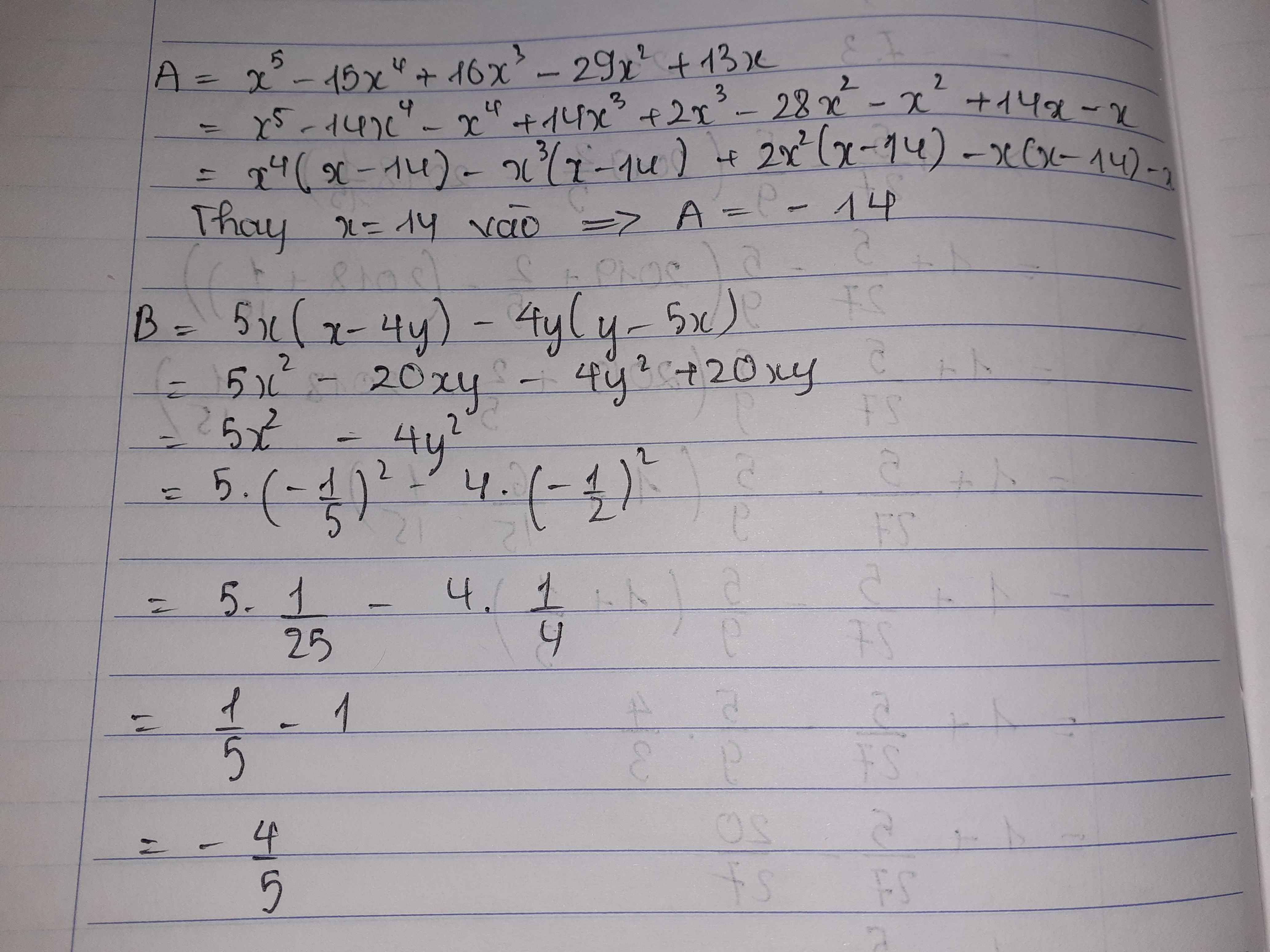( y + 1 )5 - x5 = 5y4 + 10y2 - 15y
Tìm x, y
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Sửa đề: \(2x^2+10y^2-6xy-2y-6x+10=0\)
\(\Leftrightarrow\left(x^2-6xy+9y^2\right)+\left(y^2-2y+1\right)+\left(x^2-6x+9\right)=0\\ \Leftrightarrow\left(x-3y\right)^2+\left(y-1\right)^2+\left(x-3\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=3y\\y=1\\x=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=1\end{matrix}\right.\)
Thay vào \(A\)
\(A=\dfrac{\left(3+1-4\right)^{2018}-1^{2018}}{4}=-\dfrac{1}{4}\)


a, y \(\times\) \(\dfrac{4}{3}\) = \(\dfrac{16}{9}\)
y = \(\dfrac{16}{9}\) : \(\dfrac{4}{3}\)
y = \(\dfrac{4}{3}\)
b, ( y - \(\dfrac{1}{2}\)) + 0,5 = \(\dfrac{3}{4}\)
y - 0,5 + 0,5 = \(\dfrac{3}{4}\)
y = \(\dfrac{3}{4}\)
c, \(\dfrac{4}{5}-\dfrac{2}{5}y\) = 0,2
0,8 - 0,4y = 0,2
0,4y = 0,8 - 0,2
0,4y = 0,6
y = 1,5
d, (y + \(\dfrac{3}{4}\)) \(\times\) \(\dfrac{5}{7}\) = \(\dfrac{10}{9}\)
y + \(\dfrac{3}{4}\) = \(\dfrac{10}{9}\) : \(\dfrac{5}{7}\)
y + \(\dfrac{3}{4}\) = \(\dfrac{14}{9}\)
y = \(\dfrac{14}{9}\) - \(\dfrac{3}{4}\)
y = \(\dfrac{29}{36}\)
e, y : \(\dfrac{5}{4}\) = \(\dfrac{9}{5}\) + \(\dfrac{1}{2}\)
y : \(\dfrac{5}{4}\) = \(\dfrac{23}{10}\)
y = \(\dfrac{23}{10}\)
y = \(\dfrac{23}{8}\)
f, y \(\times\) \(\dfrac{1}{2}\) + \(\dfrac{3}{2}\) \(\times\) y = \(\dfrac{4}{5}\)
y \(\times\) ( \(\dfrac{1}{2}+\dfrac{3}{2}\)) = \(\dfrac{4}{5}\)
2y = \(\dfrac{4}{5}\)
y = \(\dfrac{2}{5}\)

b) Ta có: \(B=5x\left(x-4y\right)-4y\left(y-5x\right)\)
\(=5x^2-20xy-4y^2+20xy\)
\(=5x^2-4y^2\)
\(=5\cdot\left(-\dfrac{1}{5}\right)^2-4\cdot\left(-\dfrac{1}{2}\right)^2\)
\(=5\cdot\dfrac{1}{25}-4\cdot\dfrac{1}{4}\)
\(=\dfrac{1}{5}-1=\dfrac{-4}{5}\)

Đáp án cần chọn là: A
x + y = 16 ⇒ x = 16 − y thay vào 3 + x 5 + y = − 3 − 5 ta được:
3 + 16 − y 5 + y = − 3 − 5 19 − y y + 5 = 3 5 3 y + 5 = 5 19 − y 3 y + 15 = 95 − 5 y 3 y + 5 y = 95 − 15 8 y = 80 y = 10 ⇒ x = 16 − 10 = 6
Vậy x = 6;y = 10

\(Tacó:f\left(x\right)+g\left(x\right)=x^5-x^3+x^2-2x+5+x^2-3x+1+x^2-x^4+x^5\)
Ta có : j(x) + g(x) = (x5 - x3 - x2 - 2x +5 )+( x2 - 3x + 1 + x2 - x4 + x5)
= x5 - x3 - x2 - 2x +5+x2 - 3x + 1 + x2 - x4 + x5
=(x5 + x5) + (-3x - 3x) + (-2x+2x-2x)+ (5 +1) -4x
= 10x - 6x - 2x +6 - 4x
= -2x +6
Vậy j(x) + g(x) = -2x +6

Bài 2:
a.
\(3x(x-4y)-\frac{12}{5}y(y-5x)=3x^2-12xy-\frac{12}{5}y^2+12xy\)
\(=3x^2-\frac{12}{5}y^2=3.4^2-\frac{12}{5}.(-5)^2=-12\)
b.
\(u=\frac{-1}{3}; v=\frac{-2}{3}\Rightarrow u+v+1=0\)
\(2u(1+u-v)-v(1-2u+v)=2u(1+u+v-2v)+v(1+u+v-3u)\)
\(=2u.(-2v)+v(-3u)=-4uv-3uv=-7uv=-7.\frac{-1}{3}.\frac{-2}{3}=\frac{-14}{9}\)
Bài 1:
\(A=x^6-(x^6-x^5)-(x^5+x^4)+(x^4-x^3)+(x^3+x^2)-(x^2+x)+1\)
\(=-x+1=-(x-1)=-(999-1)=-998\)

a,\(x^5+x-1=x^5+x^4-x^2-x^4-x^3+x+x^3+x^2-1=\left(x^5+x^4-x^2\right)-\left(x^4+x^3-x\right)+\left(x^3+x^2-1\right)=x^2\left(x^3+x^2-1\right)+x\left(x^3+x^2-1\right)+\left(x^3+x^2-1\right)=\left(x^2+x+1\right)\left(x^3+x^2-1\right)\)b,\(y\left(y-2\right)-5=y^2-2y-5=\left(y^2-2y+1\right)-6=\left(y-1\right)^2-\sqrt{6^2}=\left(y-1-\sqrt{6}\right)\left(y-1+\sqrt{6}\right)\)