Giải các phương trình sau: 13 24 3 x + 7 = 13 24 - 2 x + 3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TM
11 tháng 8 2021
1/ \(7x-5=13-5x\)
\(\Leftrightarrow12x=18\)
\(\Leftrightarrow x=\dfrac{3}{2}\)
Vậy: \(S=\left\{\dfrac{3}{2}\right\}\)
==========
2/ \(19+3x=5-18x\)
\(\Leftrightarrow21x=-14\)
\(\Leftrightarrow x=-\dfrac{2}{3}\)
Vậy: \(S=\left\{-\dfrac{2}{3}\right\}\)
==========
3/ \(x^2+2x-4=-12+3x+x^2\)
\(\Leftrightarrow-x=-8\)
\(\Leftrightarrow x=8\)
Vậy: \(S=\left\{8\right\}\)
===========
4/ \(-\left(x+5\right)=3\left(x-5\right)\)
\(\Leftrightarrow-x-5=3x-15\)
\(\Leftrightarrow-4x=-10\)
\(\Leftrightarrow x=\dfrac{5}{2}\)
Vậy: \(S=\left\{\dfrac{5}{2}\right\}\)
==========
5/ \(3\left(x+4\right)=\left(-x+4\right)\)
\(\Leftrightarrow3x+12=-x+4\)
\(\Leftrightarrow4x=-8\)
\(\Leftrightarrow x=-2\)
Vậy: \(S=\left\{-2\right\}\)
[----------]
NT
11 tháng 8 2021
1. \(7x-5=13-5x\) \(\Leftrightarrow12x=18\Leftrightarrow x=\dfrac{3}{2}\)
2. \(19+3x=5-18x\Leftrightarrow21x=-14\Leftrightarrow x=-\dfrac{2}{3}\)
3. \(x^2+2x-4=-12+3x+x^2\Leftrightarrow-x=-8\Leftrightarrow x=8\)
4. \(-\left(x+5\right)=3\left(x-5\right)\Leftrightarrow-x-5=3x-15\Leftrightarrow4x=10\Leftrightarrow x=\dfrac{5}{2}\)
5. \(3\left(x+4\right)=-x+4\Leftrightarrow3x+12=-x+4\Leftrightarrow4x=-8\Leftrightarrow x=-2\)
CM
27 tháng 6 2017
a) (1) ⇔ 45x – 6 – 4x2 + 4 ≥ 2x – 4x2 + 6x – 18
⇔ 37x ≥ –16 ⇔ x ≥ -16/37
Tập nghiệm: S = {x|x ≥ -16/37}
b) (2) ⇔ x2 – 6x + 9 + 2x – 2 ≤ x2 + 3 ⇔ –4x ≤ –4 ⇔ x ≥ 1
Tập nghiệm: S = {x | x ≥ 1}.
28 tháng 1 2022
\(\dfrac{x^2-26}{10}+\dfrac{x^2-25}{11}\ge\dfrac{x^2-24}{12}+\dfrac{x^2-23}{13}\)
\(\Leftrightarrow\left(\dfrac{x^2-26}{10}-1\right)+\left(\dfrac{x^2-25}{11}-1\right)\ge\left(\dfrac{x^2-24}{12}-1\right)+\left(\dfrac{x^2-23}{13}-1\right)\)
\(\Leftrightarrow\dfrac{x^2-36}{10}+\dfrac{x^2-36}{11}\ge\dfrac{x^2-36}{12}+\dfrac{x^2-36}{13}\)
\(\Leftrightarrow\dfrac{x^2-36}{10}+\dfrac{x^2-36}{11}-\dfrac{x^2-36}{12}-\dfrac{x^2-36}{13}\ge0\)
\(\Leftrightarrow\left(x^2-36\right)\left(\dfrac{1}{10}+\dfrac{1}{11}-\dfrac{1}{12}-\dfrac{1}{13}\right)\ge0\)
Vì \(\dfrac{1}{10}+\dfrac{1}{11}-\dfrac{1}{12}-\dfrac{1}{13}>0\Rightarrow x^2-36\ge0\Leftrightarrow\left[{}\begin{matrix}x\le-6\\x\ge6\end{matrix}\right.\)
28 tháng 1 2022
Bất phương trình đó tương đương với:
\(\left(\dfrac{x^2-26}{10}-1\right)+\left(\dfrac{x^2-25}{11}-1\right)\ge\left(\dfrac{x^2-24}{12}-1\right)+\left(\dfrac{x^2-23}{13}-1\right)\)
⇔ \(\dfrac{x^2-36}{10}+\dfrac{x^2-36}{11}\ge\dfrac{x^2-36}{12}+\dfrac{x^2-36}{13}\)
⇔ \(\dfrac{x^2-36}{10}+\dfrac{x^2-36}{11}-\dfrac{x^2-36}{12}-\dfrac{x^2-36}{13}\ge0\)
⇔ \(\left(x^2-36\right)\left(\dfrac{1}{10}+\dfrac{1}{11}-\dfrac{1}{12}-\dfrac{1}{13}\right)\ge0\)
+)Vì \(\dfrac{1}{10}>\dfrac{1}{11}>\dfrac{1}{12}>\dfrac{1}{13}\) nên \(\dfrac{1}{10}+\dfrac{1}{11}-\dfrac{1}{12}-\dfrac{1}{13}>0\)
⇔ \(x^2-36\ge0\)
⇔ \(x^2\ge36\)
⇔ \(\sqrt{x^2}\ge6\)
⇔ \(\left|x\right|\ge6\)
⇔ \(\left[{}\begin{matrix}x\ge6\\x\le-6\end{matrix}\right.\)
➤ Vậy \(\left[{}\begin{matrix}x\ge6\\x\le-6\end{matrix}\right.\)
CM
21 tháng 8 2017
Chọn D
Cách giải : Vì d song song với hai mặt phẳng (P) và (Q) nên nhận
PG
3
6 tháng 4 2018
1, \(_{\left|x^2-5x-6\right|=x^2+x-24}\) (1)
Điều kiện \(x^2+x-24\ge0\) <=> \(\orbr{\begin{cases}x\ge\frac{-1+\sqrt{97}}{2}\\x\le\frac{-1-\sqrt{97}}{2}\end{cases}}\)
Khi đó (1) <=> \(\orbr{\begin{cases}x^2-5x-6=x^2+x-24\\x^2-5x-6=-x^2-x+24\end{cases}}\)
<=> \(\orbr{\begin{cases}-6x=-18\\2x^2-4x-30=0\end{cases}}\)
<=> \(\orbr{\begin{cases}x=3\\x^2-2x-15=0\end{cases}}\)
<=> \(x\in\left\{-3;3;5\right\}\)
Loại 2 giá trị x = -3 và x = 3 do ko t/m đk bên trên, ta đc x = 5 là nghiệm duy nhất của pt
Vậy tập nghiệm của pt là S = {5}
6 tháng 4 2018
|x^2-5x-6|=x^2+x-24
=>x= 5
|x-1|-2|x-2|+3|x-3|=4
=> x= 5 hoac bang 1
PT
1
CM
11 tháng 4 2017
ĐKXĐ: x ≠ - 1;x ≠ 3.
Ta có: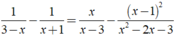
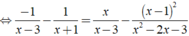
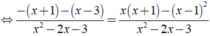
⇔ -2x + 2 = 3x - 1
⇔ 5x = 3 ⇔ x = 3/5.
Kết hợp điều kiện, vậy phương trình đã cho có nghiệm là x = 3/5.
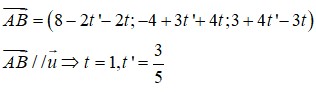
Phương trình đã cho tương đương với
13 24 3 x + 7 = 13 24 - 2 x + 3
⇔ 3x + 7 = –2x – 3 ⇔ x = –2