tính góc tạo bởi giữa hai vectơ trong trường hợp a(3;4),b(-4;3)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
nên 
HQ
Hà Quang Minh
Giáo viên
26 tháng 9 2023
a) \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}} = \frac{{2.6 + ( - 3).4}}{{\sqrt {{2^2} + {{\left( { - 3} \right)}^2}} .\sqrt {{6^2} + {4^2}} }} = 0 \Rightarrow \overrightarrow a \bot \overrightarrow b \)
b) \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}} = \frac{{3.5 + 2.( - 1)}}{{\sqrt {{3^2} + {2^2}} .\sqrt {{5^2} + {{\left( { - 1} \right)}^2}} }} = \frac{{\sqrt 2 }}{2} \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = 45^\circ \)
c) \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}} = \frac{{\left( { - 2} \right).3 + ( - 2\sqrt 3 ).\sqrt 3 }}{{\sqrt {{{\left( { - 2} \right)}^2} + {{\left( { - 2\sqrt 3 } \right)}^2}} .\sqrt {{3^2} + {{\sqrt 3 }^2}} }} = - \frac{{\sqrt 3 }}{2} \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = 150^\circ \)
HQ
Hà Quang Minh
Giáo viên
24 tháng 9 2023
a)
\(\overrightarrow a .\overrightarrow b = ( - 3).2 + 1.6 = 0\)
\( \Rightarrow \overrightarrow a \bot \overrightarrow b \) hay \(\left( {\overrightarrow a ,\overrightarrow b } \right) = {90^o}\).
b)
\(\left\{ \begin{array}{l}\overrightarrow a .\overrightarrow b = 3.2 + 1.4 = 10\\|\overrightarrow a |\, = \sqrt {{3^2} + {1^2}} = \sqrt {10} ;\;\,|\overrightarrow b |\, = \sqrt {{2^2} + {4^2}} = 2\sqrt 5 \end{array} \right.\)
\(\begin{array}{l} \Rightarrow \cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{10}}{{\sqrt {10} .2\sqrt 5 }} = \frac{{\sqrt 2 }}{2}\\ \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = {45^o}\end{array}\)
c) Dễ thấy: \(\overrightarrow a \) và \(\overrightarrow b \) cùng phương do \(\frac{{ - \sqrt 2 }}{2} = \frac{1}{{ - \sqrt 2 }}\)
Hơn nữa: \(\overrightarrow b = \left( {2; - \sqrt 2 } \right) = - \sqrt 2 .\left( { - \sqrt 2 ;1} \right) = - \sqrt 2 .\overrightarrow a \;\); \( - \sqrt 2 < 0\)
Do đó: \(\overrightarrow a \) và \(\overrightarrow b \) ngược hướng.
\( \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = {180^o}\)
Chú ý:
Khi tính góc, ta kiểm tra các trường hợp dưới đây trước:
+ \(\left( {\overrightarrow a ,\overrightarrow b } \right) = {90^o}\): nếu \(\overrightarrow a .\overrightarrow b = 0\)
+ \(\overrightarrow a \) và \(\overrightarrow b \) cùng phương:
\(\left( {\overrightarrow a ,\overrightarrow b } \right) = {0^o}\) nếu \(\overrightarrow a \) và \(\overrightarrow b \) cùng hướng
\(\left( {\overrightarrow a ,\overrightarrow b } \right) = {180^o}\) nếu \(\overrightarrow a \) và \(\overrightarrow b \) ngược hướng
Nếu không thuộc các trường hợp trên thì ta tính góc dựa vào công thức \(\cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{\overrightarrow a .\overrightarrow b }}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}}\).
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 4 2020
\(\overrightarrow{a}.\overrightarrow{b}=\left|\overrightarrow{a}\right|.\left|\overrightarrow{b}\right|.cos\left(\overrightarrow{a};\overrightarrow{b}\right)\)
a/ \(\overrightarrow{a}.\overrightarrow{b}=8.\sqrt{3}.cos30^0=12\)
b/ \(\overrightarrow{a}.\overrightarrow{b}=\sqrt{2}.6.cos45^0=6\)
c/ \(\overrightarrow{a}.\overrightarrow{b}=9.10.cos60^0=45\)
d/ \(\overrightarrow{a}.\overrightarrow{b}=5.6.cos120^0=-15\)
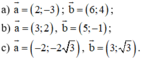
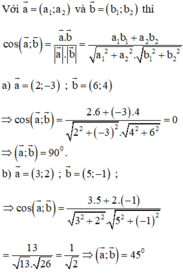
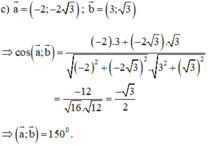
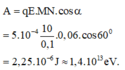
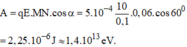
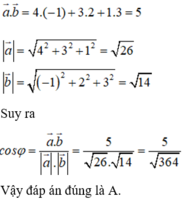
\(cos\left(\overrightarrow{a};\overrightarrow{b}\right)=\frac{3.\left(-4\right)+4.3}{\sqrt{3^2+4^2}.\sqrt{\left(-4\right)^2+3^2}}=0\)
\(\Rightarrow\left(\overrightarrow{a};\overrightarrow{b}\right)=90^0\)