Bài tập 3: Cho hàm số
f( x )=c o s x. Chứng minh rằng:
2f'(x+pi/3).f'(x-pi/6)=f'(0)-f(2x+pi/6)
Bài tập 4: Cho hàm số y=3(sin^4 x +cos^4 )-2(sin^6 x +cos^6 x). Chứng minh rằng: y'=0 \-/ x€ Z
Bài tập 5: Cho hàm số
Y= (sin x/ 1+cos x)^3. CMR: y'.sinx-3y=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn D
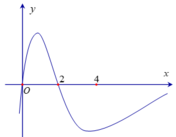
Từ đồ thị của hàm số y = f'(x) ta suy ra bảng biến thiên của hàm số y = f(x) trên đoạn như sau:
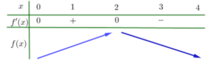
Từ bảng biến thiên, ta có nhận xét sau: ![]()
![]()
Ta lại có: f(0) + f(1) - 2f(2) = f(4). - f(3)
![]()
![]()

Bài 4:
\(f\left(5\right)-f\left(4\right)=2019\)
=>\(125a+25b+25c+d-64a-16b-4c-d=2019\)
=>\(61a+9b+21c=2019\)
\(f\left(7\right)-f\left(2\right)\)
\(=343a+49b+7c+d-8a-4b-2c-d\)
\(=335a+45b+5c\)
\(=5\left(61a+9b+21c\right)=5\cdot2019\) là hợp số

Ta có ![]()
![]()
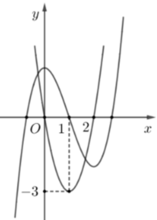
Quan sát đồ thị có 
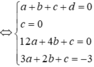

![]()
Đặt ![]() phương trình trở thành:
phương trình trở thành:
![]()
![]()
![]()
Khi đó ![]()
Phương trình này có 3 nghiệm phân biệt
![]()
Tổng các phần tử củaS bằng 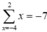
Chọn đáp án C.

b) f(1) + 2f(-2) = a - 1 + 2(a - 1).(-2) = a - 1 - 4a + 4 = -3a + 3 = -3(a - 1) = f(-3) (đpcm)

Chọn A
Dựa vào đồ thị của hàm f'(x) ta có bảng biến thiên.
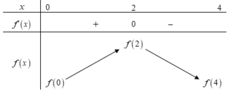
Vậy giá trị lớn nhất M = f(2)
Hàm số đồng biến trên khoảng (0;2) nên f(2) > f(1) => f(2) - f(1) > 0 .
Hàm số nghịch biến trên khoảng (2;4) nên f(2) > f(3) => f(2) - f(3) > 0.
Theo giả thuyết: f(0) + f(1) - 2f(2) = f(4) - f(3).
![]()
=> f(0) > f(4)
Vậy giá trị nhỏ nhất m = f(4)
3.
\(f\left(x+\frac{\pi}{3}\right)=cos\left(x+\frac{\pi}{3}\right)\Rightarrow f'\left(x+\frac{\pi}{3}\right)=-sin\left(x+\frac{\pi}{3}\right)\)
\(f'\left(x-\frac{\pi}{6}\right)=-sin\left(x-\frac{\pi}{6}\right)\)
\(f'\left(0\right)=-sin\left(0\right)=0\)
\(2f'\left(x+\frac{\pi}{3}\right).f'\left(x-\frac{\pi}{6}\right)=2sin\left(x+\frac{\pi}{3}\right)sin\left(x-\frac{\pi}{6}\right)\)
\(=cos\left(\frac{\pi}{2}\right)-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)=0-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(\Rightarrow2f'\left(x+\frac{\pi}{3}\right)f'\left(x-\frac{\pi}{6}\right)=f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)\) (đpcm)
4.
\(y=3\left(sin^4x+cos^4x\right)-2\left(sin^6x+cos^6x\right)\)
\(=3\left(sin^2x+cos^2x\right)^2-6sin^2x.cos^2x-2\left(sin^2x+cos^2x\right)^3+6sin^2x.cos^2x\left(sin^2x+cos^2x\right)\)
\(=3-2=1\)
\(\Rightarrow y'=0\) ; \(\forall x\)
5.
\(y=\left(\frac{sinx}{1+cosx}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{1-cos^2x}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{sin^2x}\right)^3=\left(\frac{1-cosx}{sinx}\right)^3\)
\(y'=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{sin^2x-cosx\left(1-cosx\right)}{sin^2x}\right)=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{1-cosx}{sin^2x}\right)=\frac{3\left(1-cosx\right)^3}{sin^4x}\)
\(\Rightarrow y'.sinx-3y=\frac{3\left(1-cosx\right)^3}{sin^3x}-3\left(\frac{1-cosx}{sinx}\right)^3=0\) (đpcm)