Giải phương trình: \(\left(x-3\right)^5+\left(5-x\right)^2=32\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
RK
28 tháng 8 2021
a) \(x^4-x^2+\dfrac{1}{4}-\dfrac{225}{4}=0\\ \left(x^2-\dfrac{1}{2}\right)^2-\dfrac{15}{2}^2=0\\ \left(x+7\right)\left(x-8\right)=0\\ \left[{}\begin{matrix}x=8\\x=-7\end{matrix}\right.\)
Vậy x = 8 hoặc x = -7
28 tháng 8 2021
a: Ta có: \(x^4-x^2-56=0\)
\(\Leftrightarrow x^4-8x^2+7x^2-56=0\)
\(\Leftrightarrow\left(x^2-8\right)\left(x^2+7\right)=0\)
\(\Leftrightarrow x^2-8=0\)
hay \(x\in\left\{2\sqrt{2};-2\sqrt{2}\right\}\)
TA
0
CD
2
22 tháng 2 2017
Câu này cách làm tương tự câu mũ 4 ở trên nhé, đặt ẩn phụ và biến đổi như vậy
KV
15 tháng 12 2023
x(x + 2)(x + 3)(x + 5) = 72
⇔ (x² + 5x)(x² + 5x + 6) - 72 = 0 (1)
Đặt u = x² + 5x
⇒ x² + 5x + 6 = u + 6
(1) ⇔ u.(u + 6) - 72 = 0
⇔ u² + 6u - 72 = 0
⇔ u² + 12u - 6u - 72 = 0
⇔ (u² + 12u) - (6u + 72) = 0
⇔ u(u + 12) - 6(u + 12) = 0
⇔ (u + 12)(u - 6) = 0
⇔ u + 12 = 0 hoặc u - 6 = 0
*) u + 12 = 0
⇔ u = -12
⇒ x² + 5x = -12
⇔ x² + 5x + 12 = 0
⇔ x² + 2.5x/2 + 25/4 + 23/4 = 0
⇔ (x + 5/2)² + 23/4 = 0 (vô lý)
*) u - 6 = 0
⇔ u = 6
⇒ x² + 5x = 6
⇔ x² + 5x - 6 = 0
⇔ x² - x + 6x - 6 = 0
⇔ (x² - x) + (6x - 6) = 0
⇔ x(x - 1) + 6(x - 1) = 0
⇔ (x - 1)(x + 6) = 0
⇔ x - 1 = 0 hoặc x + 6 = 0
**) x - 1 = 0
⇔ x = 1
**) x + 6 = 0
⇔ x = -6
Vậy S = {-6; 1}
H
1 tháng 6 2023
\(\left|x-2\right|=\left|2x-3\right|\)
Nếu : \(\left\{{}\begin{matrix}2x-3\ge0\Leftrightarrow2x\ge3\Leftrightarrow x\ge\dfrac{3}{2}\\2x-3< 0\Leftrightarrow2x< 3\Leftrightarrow x< \dfrac{3}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=2x-3\\x-2=-\left(2x-3\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}-x=-3+2\\x-2=-2x+3\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}-x=-1\\3x=5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\left(ktm\right)\\x=\dfrac{5}{3}\left(ktm\right)\end{matrix}\right.\)
Vậy pt vô nghiệm
__
\(\left|5-x\right|=\left|x+2\right|\)
Nếu : \(\left\{{}\begin{matrix}x+2\ge0\Leftrightarrow x\ge-2\\x+2< 0\Leftrightarrow x< -2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}5-x=x+2\\5-x=-\left(x+2\right)\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}2x=2-5\\5-x=-x-2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}2x=-3\\0=-7\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{3}{2}\left(ktm\right)\\0=-7\left(ktm\right)\end{matrix}\right.\)
Vậy pt vô nghiệm
1N
1
NH
15 tháng 3 2023
`(x-5)^2 +3(x-5)=0`
`<=>(x-5)(x-5+3)=0`
`<=>(x-5)(x-2)=0`
\(< =>\left[{}\begin{matrix}x-5=0\\x-2=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=5\\x=2\end{matrix}\right.\)
24 tháng 1 2021
a) Ta có: \(\left\{{}\begin{matrix}2\left(x+1\right)-3\left(y-2\right)=5\\-4\left(x-2\right)+5\left(y-3\right)=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x+2-3y+6=5\\-4x+8+5y-15=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-3y=-3\\-4x+5y=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x-6y=-6\\-4x+5y=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-y=0\\2x-3y=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\2x-3\cdot0=-3\end{matrix}\right.\)
hay \(\left\{{}\begin{matrix}x=-\dfrac{3}{2}\\y=0\end{matrix}\right.\)
Vậy: hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=-\dfrac{3}{2}\\y=0\end{matrix}\right.\)
b) Ta có: \(\left\{{}\begin{matrix}8\left(x-3\right)-3\left(y+1\right)=-2\\3\left(x+2\right)-2\left(1-y\right)=5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}8x-24-3y-3=-2\\3x+6-2+2y=5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}8x-3y=25\\3x+2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}24x-9y=75\\24x+16y=8\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-25y=67\\3x+2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{-67}{25}\\3x=1-2y\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x=1-2\cdot\dfrac{-67}{25}=\dfrac{159}{25}\\y=-\dfrac{67}{25}\end{matrix}\right.\)
hay \(\left\{{}\begin{matrix}x=\dfrac{53}{25}\\y=-\dfrac{67}{25}\end{matrix}\right.\)
Vậy: Hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=\dfrac{53}{25}\\y=-\dfrac{67}{25}\end{matrix}\right.\)
24 tháng 1 2021
a) HPT \(\Leftrightarrow\left\{{}\begin{matrix}2x-3y=-3\\-4x+5y=6\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}4x-6y=-6\\-4x+5y=6\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}-y=0\\x=\dfrac{3y-3}{2}\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}y=0\\x=-\dfrac{3}{2}\end{matrix}\right.\)
Vậy hệ phương trình có nghiệm \(\left(x;y\right)=\left(-\dfrac{3}{2};0\right)\)
b) HPT \(\Leftrightarrow\left\{{}\begin{matrix}8x-3y=25\\3x+2y=1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}16x-6y=50\\9x+6y=3\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}25x=53\\y=\dfrac{1-3x}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{53}{25}\\y=-\dfrac{67}{25}\end{matrix}\right.\)
Vậy hệ phương trình có nghiệm \(\left(x;y\right)=\left(\dfrac{53}{25};-\dfrac{67}{25}\right)\)
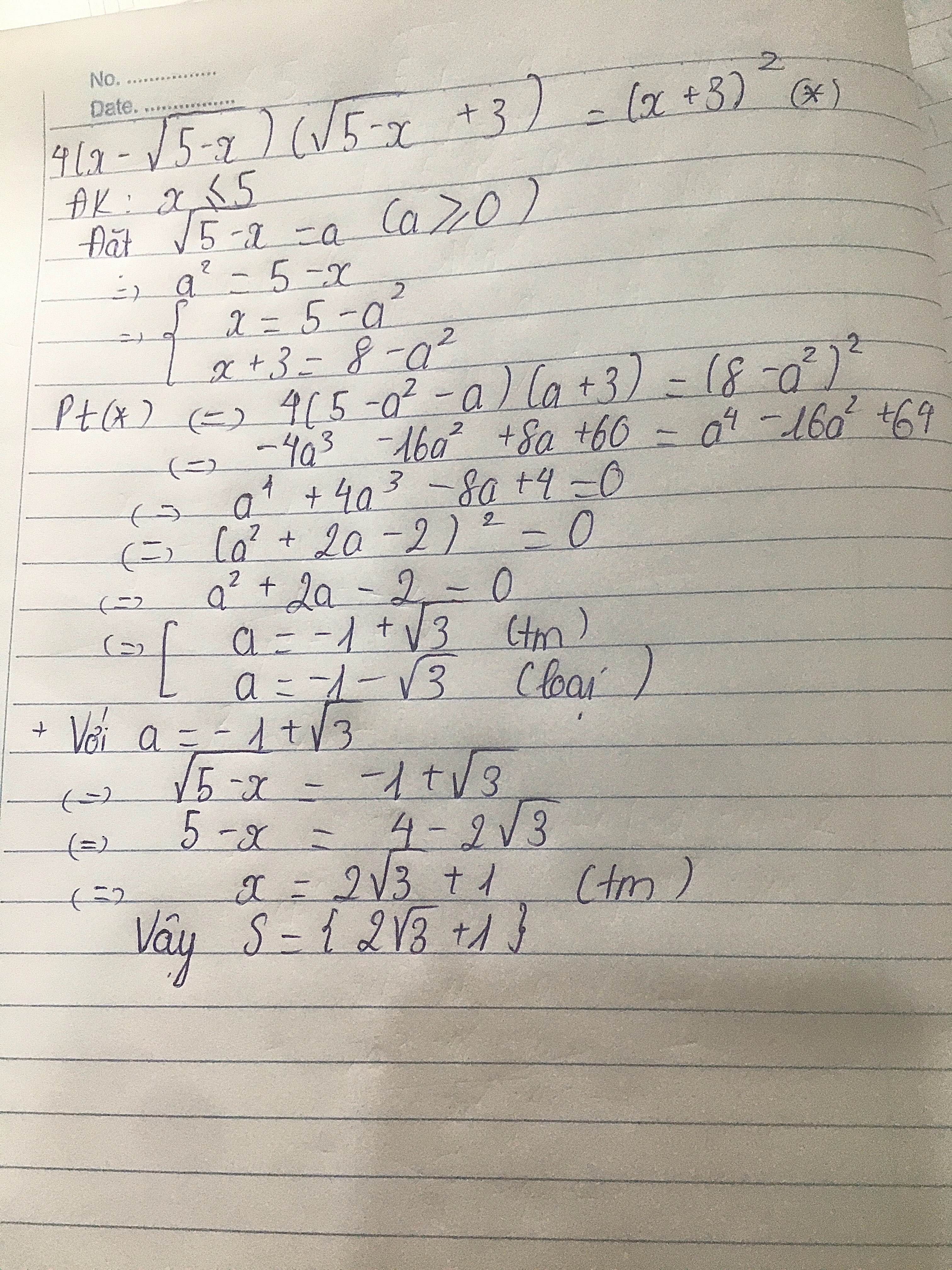
x=5
cách lm bn ơi