1) tìm giá trị lớn nhất của A = -x2+6x+7
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
VV
1
30 tháng 1 2018
A+1 = x^2+6x+9/x^2+1 = (x+3)^2/x^2+1 >= 0
=> A >= -1
Dấu "=" xảy ra <=> x+3=0 <=> x=-3
Vậy GTNN của A = -1 <=> x=-3
Tk mk nha
C
3
20 tháng 7 2021
Ta có:A=-x2+6x+8=-(x2-6x+9)+17=-(x-3)2+17\(\le\)17
Dấu "=" xảy ra <=> x=3
TA
20 tháng 7 2021
`A=-x^2+6x+8=-(x^2-6x-8)`
`=-(x^2-2.x.3 +3^2 -17)`
`=-(x-3)^2+17`
Vì: `(x-3)^2 >= 0 forall x`
`=> -(x-3)^2 <=0 `
`<=>-(x-3)^2+17 <=17`
`=> A_(max) = 17 <=> x-3=0<=>x=3`
13 tháng 6 2023
1:
=x^2-6x+9-4=(x-3)^2-4>=-4
Dấu = xảy ra khi x=3
3: =-y^2-4y-4+13
=-(y+2)^2+13<=13
Dấu = xảy ra khi y=-2
4: D=x^2-8>=-8
Dấu = xảy ra khi x=0
KV
1 tháng 10 2023
A = -x² - 6x + 1
= -(x² + 6x - 1)
= -(x² + 6x + 9 - 10)
= -[(x + 3)² - 10]
= -(x + 3)² + 10
Do (x + 3)² ≥ 0 với mọi x ∈ R
⇒ -(x + 3)² ≤ 0 với mọi x ∈ R
⇒ -(x + 3)² + 10 ≤ 10 với mọi x ∈ R
Vậy GTLN của A là 10 khi x = -3
DN
1 tháng 10 2023
\(A=-x^2-6x+1\)
\(A=-\left(x^2+6x-1\right)\)
\(A=-\left(x^2+6x+9-10\right)\)
\(A=-\left(x^2+2\cdot x\cdot3+3^2\right)+10\)
\(A=-\left(x+3\right)^2+10\)
Có: \(\left(x+3\right)^2\ge0\forall x\Rightarrow-\left(x+3\right)^2\le0\)
\(\Rightarrow-\left(x+3\right)^2+10\le10\)
\(\Rightarrow A\le10\)
Dấu "=" xảy ra khi \(\left(x+3\right)^2=0\Leftrightarrow x+3=0\)
\(\Leftrightarrow x=-3\)
Vậy: \(A_{min}=10\Leftrightarrow x=-3\)
22 tháng 11 2023
Bài 1:
a: \(A=x^2+2x+4\)
\(=x^2+2x+1+3\)
\(=\left(x+1\right)^2+3>=3\forall x\)
Dấu '=' xảy ra khi x+1=0
=>x=-1
Vậy: \(A_{min}=3\) khi x=-1
b: \(B=x^2-20x+101\)
\(=x^2-20x+100+1\)
\(=\left(x-10\right)^2+1>=1\forall x\)
Dấu '=' xảy ra khi x-10=0
=>x=10
Vậy: \(B_{min}=1\) khi x=10
c: \(C=x^2-2x+y^2+4y+8\)
\(=x^2-2x+1+y^2+4y+4+3\)
\(=\left(x-1\right)^2+\left(y+2\right)^2+3>=3\forall x\)
Dấu '=' xảy ra khi x-1=0 và y+2=0
=>x=1 và y=-2
Vậy: \(C_{min}=3\) khi (x,y)=(1;-2)
Bài 2:
a: \(A=5-8x-x^2\)
\(=-\left(x^2+8x\right)+5\)
\(=-\left(x^2+8x+16-16\right)+5\)
\(=-\left(x+4\right)^2+16+5=-\left(x+4\right)^2+21< =21\forall x\)
Dấu '=' xảy ra khi x+4=0
=>x=-4
b: \(B=x-x^2\)
\(=-\left(x^2-x\right)\)
\(=-\left(x^2-x+\dfrac{1}{4}-\dfrac{1}{4}\right)\)
\(=-\left(x-\dfrac{1}{2}\right)^2+\dfrac{1}{4}< =\dfrac{1}{4}\forall x\)
Dấu '=' xảy ra khi \(x-\dfrac{1}{2}=0\)
=>\(x=\dfrac{1}{2}\)
c: \(C=4x-x^2+3\)
\(=-x^2+4x-4+7\)
\(=-\left(x^2-4x+4\right)+7\)
\(=-\left(x-2\right)^2+7< =7\forall x\)
Dấu '=' xảy ra khi x-2=0
=>x=2
d: \(D=-x^2+6x-11\)
\(=-\left(x^2-6x+11\right)\)
\(=-\left(x^2-6x+9+2\right)\)
\(=-\left(x-3\right)^2-2< =-2\forall x\)
Dấu '=' xảy ra khi x-3=0
=>x=3
30 tháng 8 2021
a) \(A=x^2-4x+1=\left(x-2\right)^2-3\ge-3\)
\(minA=-3\Leftrightarrow x=2\)
b) \(B=-x^2-8x+5=-\left(x+4\right)^2+21\le21\)
\(maxB=21\Leftrightarrow x=-4\)
c) \(C=2x^2-8x+19=2\left(x-2\right)^2+11\ge11\)
\(minC=11\Leftrightarrow x=2\)
d) \(D=-3x^2-6x+1=-3\left(x+1\right)^2+4\le4\)
\(maxD=4\Leftrightarrow x=-1\)
CM
15 tháng 1 2018
Ta có: A = x 2 - 6 x + 11 = x 2 - 2 . 3 x + 9 + 2 = x - 3 2 + 2
Vì x - 3 2 ≥ 0 nên x - 3 2 + 2 ≥ 2
Suy ra: A ≥ 2.
A = 2 khi và chỉ khi x - 3 = 0 suy ra x = 3
Vậy A = 2 là giá trị nhỏ nhất của biểu thức tại x =3.
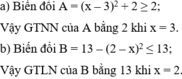
\(A=-x^2+6x+7\)
\(A=-\left(x^2-6x-7\right)\)
\(A=-\left(x^2-2\cdot x\cdot3+3^2-16\right)\)
\(A=-\left[\left(x-3\right)^2-16\right]\)
\(A=16-\left(x-3\right)^2\le16\forall x\)
Dấu "=" xảy ra \(\Leftrightarrow x-3=0\Leftrightarrow x=3\)
\(A=-x^2+6x+7\)
\(A=-\left(x^2-2.x.3+3^2-16\right)\)
\(A=-\left(x^2-2.x.3+3^2\right)+16\)
\(A=-\left(x-3\right)^2+16\)
Ta có :\(\left(x-3\right)^2\ge0\)
\(\Rightarrow-\left(x-3\right)^2\le0\)
\(\Rightarrow A\le16\)
\(\Rightarrow Max\)\(A=16\)
\(Khi:\left(x-3\right)=0\)
\(\Rightarrow x=3\)