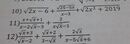
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a: ĐKXĐ: \(x\ge2\)
b: ĐKXĐ: \(x< 5\)
c: ĐKXĐ: \(\left\{{}\begin{matrix}-3< x\le2\\x\ne-1\end{matrix}\right.\)

ĐKXĐ: \(x\ge0\)
Khi đó \(2x+1+\sqrt{x}\) hiển nhiên dương nên ko cần tìm điều kiện cho căn to nữa


ĐKXĐ:
\(\left\{{}\begin{matrix}x^2-2x-3\ge0\\1-x^2\ge0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x\le-1\\x\ge3\end{matrix}\right.\\-1\le x\le1\end{matrix}\right.\) \(\Rightarrow x=-1\)
để H xác định thì:
\(x^2-2x-3\ge0\) và \(1-x^2\ge0\)
\(\Rightarrow x^2-2x\ge3\) \(\Rightarrow-x^2\ge-1\)
\(\Rightarrow x\left(x-2\right)\ge3\) \(\Rightarrow x^2\le1\)
\(\Rightarrow x\ge3\) hoặc \(x-2\ge3\) \(\Rightarrow x\le1\)
\(\Rightarrow x\ge5\)
