Tìm x thuộc Z để biểu thức sau thuộc Z:
a) \(\dfrac{x^2+2x^2+6x+8}{x+1}\)
b) \(\dfrac{2x^2+x-2}{x-3}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NL
Nguyễn Lê Phước Thịnh
21 tháng 5 2022
Đúng(0)
Những câu hỏi liên quan
21 tháng 5 2022
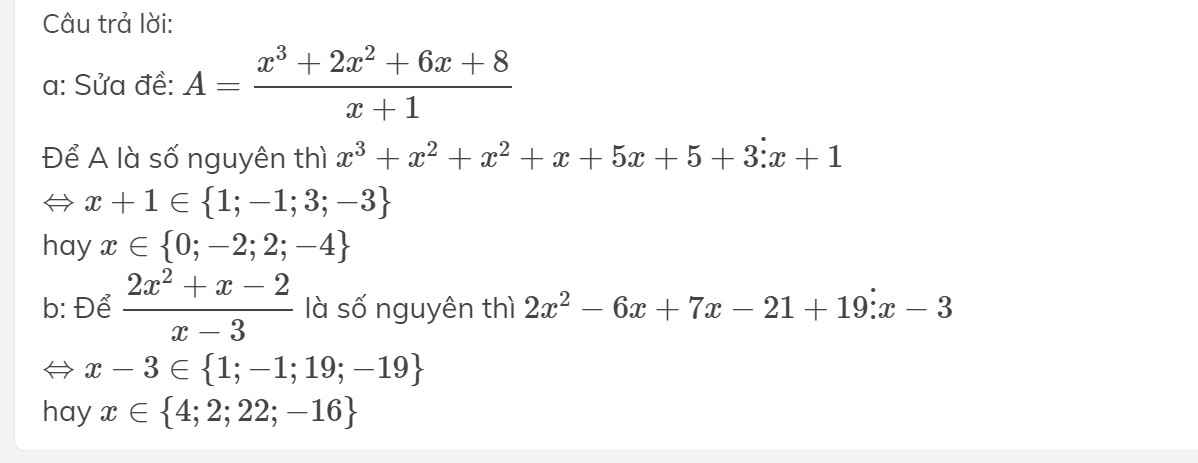
a: Sửa đề: \(A=\dfrac{x^3+2x^2+6x+8}{x+1}\)
Để A là số nguyên thì \(x^3+x^2+x^2+x+5x+5+3⋮x+1\)
\(\Leftrightarrow x+1\in\left\{1;-1;3;-3\right\}\)
hay \(x\in\left\{0;-2;2;-4\right\}\)
b: Để \(\dfrac{2x^2+x-2}{x-3}\) là số nguyên thì \(2x^2-6x+7x-21+19⋮x-3\)
\(\Leftrightarrow x-3\in\left\{1;-1;19;-19\right\}\)
hay \(x\in\left\{4;2;22;-16\right\}\)
14 tháng 3 2022
a. \(A=\left(\dfrac{2-3x}{x^2+2x-3}-\dfrac{x+3}{1-x}-\dfrac{x+1}{x+3}\right):\dfrac{3x+12}{x^3-1}\left(ĐKXĐ:x\ne1;x\ne-3\right)\)
\(=\left(\dfrac{2-3x}{\left(x-1\right)\left(x+3\right)}+\dfrac{x+3}{x-1}-\dfrac{x+1}{x+3}\right):\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\left(\dfrac{2-3x}{\left(x-1\right)\left(x+3\right)}+\dfrac{\left(x+3\right)^2}{\left(x-1\right)\left(x+3\right)}-\dfrac{\left(x-1\right)\left(x+1\right)}{\left(x-1\right)\left(x+3\right)}\right):\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{2-3x+x^2+6x+9-x^2+1}{\left(x-1\right)\left(x+3\right)}:\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{3x+12}{\left(x-1\right)\left(x+3\right)}:\dfrac{3x+12}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{3x+12}{\left(x-1\right)\left(x+3\right)}.\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{3x+12}=\dfrac{x^2+x+1}{x+3}\)
\(M=A.B=\dfrac{x^2+x+1}{x+3}.\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+x-2}{x+3}\)
b. -Để M thuộc Z thì:
\(\left(x^2+x-2\right)⋮\left(x+3\right)\)
\(\Rightarrow\left(x^2+3x-2x-6+4\right)⋮\left(x+3\right)\)
\(\Rightarrow\left[x\left(x+3\right)-2\left(x+3\right)+4\right]⋮\left(x+3\right)\)
\(\Rightarrow4⋮\left(x+3\right)\)
\(\Rightarrow x+3\in\left\{1;2;4;-1;-2;-4\right\}\)
\(\Rightarrow x\in\left\{-2;-1;1;-4;-5;-7\right\}\)
c. \(A^{-1}-B=\dfrac{x+3}{x^2+x+1}-\dfrac{x^2+x-2}{x^3-1}\)
\(=\dfrac{x+3}{x^2+x+1}-\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{\left(x+3\right)\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{x^2+x-2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^2-x+3x-3-x^2-x+2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x-1}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{1}{x^2+x+1}\)
\(=\dfrac{1}{x^2+2.\dfrac{1}{2}x+\dfrac{1}{4}+\dfrac{3}{4}}=\dfrac{1}{\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}}\le\dfrac{1}{\dfrac{3}{4}}=\dfrac{4}{3}\)
\(Max=\dfrac{4}{3}\Leftrightarrow x=\dfrac{-1}{2}\)
30 tháng 4 2023
a: \(B=\dfrac{3x\left(2x-3\right)-4\left(2x+3\right)-4x^2+23x+12}{\left(2x-3\right)\left(2x+3\right)}\cdot\dfrac{2x+3}{x+3}\)
\(=\dfrac{6x^2-9x-8x-12-4x^2+23x+12}{2x-3}\cdot\dfrac{1}{x+3}\)
\(=\dfrac{2x^2+6x}{\left(2x-3\right)}\cdot\dfrac{1}{x+3}=\dfrac{2x}{2x-3}\)
b: 2x^2+7x+3=0
=>(2x+3)(x+2)=0
=>x=-3/2(loại) hoặc x=-2(nhận)
Khi x=-2 thì \(A=\dfrac{2\cdot\left(-2\right)}{-2-3}=\dfrac{-4}{-7}=\dfrac{4}{7}\)
d: |B|<1
=>B>-1 và B<1
=>B+1>0 và B-1<0
=>\(\left\{{}\begin{matrix}\dfrac{2x+2x-3}{2x-3}>0\\\dfrac{2x-2x+3}{2x-3}< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x-3< 0\\\dfrac{4x-3}{2x-3}>0\end{matrix}\right.\Leftrightarrow x< \dfrac{3}{4}\)
19 tháng 12 2020
a)
ĐKXĐ: \(x\ne-4\)
Để A nguyên thì \(3x+21⋮x+4\)
\(\Leftrightarrow3x+12+9⋮x+4\)
mà \(3x+12⋮x+4\)
nên \(9⋮x+4\)
\(\Leftrightarrow x+4\inƯ\left(9\right)\)
\(\Leftrightarrow x+4\in\left\{1;-1;3;-3;9;-9\right\}\)
\(\Leftrightarrow x\in\left\{-3;-5;-1;-7;5;-13\right\}\)(nhận)
Vậy: Để A nguyên thì \(x\in\left\{-3;-5;-1;-7;5;-13\right\}\)
b) ĐKXĐ: \(x\ne\dfrac{1}{2}\)
Để B nguyên thì \(2x^3-7x^2+7x+5⋮2x-1\)
\(\Leftrightarrow2x^3-x^2-6x^2+3x+4x-2+7⋮2x-1\)
\(\Leftrightarrow x^2\left(2x-1\right)-3x\left(2x-1\right)+2\left(2x-1\right)+7⋮2x-1\)
\(\Leftrightarrow\left(2x-1\right)\left(x^2-3x+2\right)+7⋮2x-1\)
mà \(\left(2x-1\right)\left(x^2-3x+2\right)⋮2x-1\)
nên \(7⋮2x-1\)
\(\Leftrightarrow2x-1\inƯ\left(7\right)\)
\(\Leftrightarrow2x-1\in\left\{1;-1;7;-7\right\}\)
\(\Leftrightarrow2x\in\left\{2;0;8;-6\right\}\)
hay \(x\in\left\{1;0;4;-3\right\}\)(nhận)
Vậy: \(x\in\left\{1;0;4;-3\right\}\)
1 tháng 12 2021
\(a,P=\dfrac{2x^2+2x+2+2x-1+x^2+6x+2}{\left(x-1\right)\left(x^2+x+1\right)}\\ P=\dfrac{3x^2+10x+3}{\left(x-1\right)\left(x^2+x+1\right)}\)
7 tháng 11 2021
\(a,P=\left[\dfrac{x+1}{3x\left(x+1\right)}-\dfrac{2x-1}{3x\left(2x-1\right)}-1\right]\cdot\dfrac{2x}{1-x}\left(x\ne1;x\ne-1;x\ne0\right)\\ P=\left(\dfrac{1}{3x}-\dfrac{1}{3x}-1\right)\cdot\dfrac{2x}{1-x}\\ P=-1\cdot\dfrac{2x}{1-x}=\dfrac{2x}{x-1}\\ b,P=2+\dfrac{2}{x-1}\in Z\\ \Leftrightarrow x-1\inƯ\left(2\right)=\left\{-2;-1;1;2\right\}\\ \Leftrightarrow x\in\left\{2;3\right\}\left(x\ne-1;x\ne0\right)\\ c,P\le1\Leftrightarrow\dfrac{2x}{x-1}-1\le0\\ \Leftrightarrow\dfrac{x+1}{x-1}\le0\\ \Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x+1\le0\\x-1>0\end{matrix}\right.\\\left\{{}\begin{matrix}x+1\ge0\\x-1< 0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow-1\le x< 1\)
7 tháng 11 2021
a: \(P=\left(\dfrac{x+1}{3x\left(x+1\right)}-\dfrac{2x-1}{3x\left(2x-1\right)}-1\right)\cdot\dfrac{2x}{x-1}\)
\(=\dfrac{1-1-3x}{3x}\cdot\dfrac{2x}{x-1}\)
\(=\dfrac{-3x}{3x}\cdot\dfrac{2x}{x-1}=\dfrac{-2x}{x-1}\)
24 tháng 11 2021
1) Xét rằng x > 7 <=> A < 0
Lại xét x < 7 thì mẫu là một số nguyên dương. P/s A có tử và mẫu đều là số dương, mà tử lại bất biến
A(max) <=> mẫu 7 - x nhỏ nhất <=> 7 - x = 1 => x = 7 - 1 = 6 <=> A = 1
Từ những điều trên thì A sẽ có GTLN khi và chỉ khi x = 6
26 tháng 5 2022
a: \(P=\left[\left(x-2\right)\left(x^2+2x+4\right)\cdot\dfrac{x+2}{x^2+2x+4}-\dfrac{\left(x-2\right)\left(x+2\right)}{x^2+2x+4}\cdot\dfrac{\left(x-2\right)\left(x^2+2x+4\right)}{x+2}\right]:\left(x-1\right)\)
\(=\dfrac{\left[x^2-4-\left(x-2\right)^2\right]}{x-1}\)
\(=\dfrac{x^2-4-x^2+4x-4}{x-1}=\dfrac{4x}{x-1}\)
b: Để P là số nguyên thì \(4x-4+4⋮x-1\)
\(\Leftrightarrow x-1\in\left\{1;-1;2;-2;4;-4\right\}\)
hay \(x\in\left\{0;3;-1;5;-3\right\}\)