9 x (-1/3) 3 + 1/3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
23 tháng 8 2023
\(\dfrac{1}{3}+\dfrac{5}{9}=\dfrac{6}{18}+\dfrac{10}{18}=\dfrac{16}{18}=\dfrac{8}{9}\)
\(\dfrac{1}{7}-\dfrac{1}{9}=\dfrac{9}{63}-\dfrac{7}{63}=\dfrac{2}{63}\)
\(3:\dfrac{5}{9}=3.\dfrac{9}{5}=\dfrac{27}{5}\)
\(3.\dfrac{5}{9}=\dfrac{15}{9}=\dfrac{5}{3}\)
\(\dfrac{1}{9}.\dfrac{9}{3}=\dfrac{1}{3}\)
\(\dfrac{1}{3}:\dfrac{1}{7}=\dfrac{7}{3}\)
\(9+\dfrac{9}{3}=9+3=12\)
\(4-\dfrac{2}{4}=4-\dfrac{1}{2}=\dfrac{7}{2}\)
23 tháng 8 2023
\(\dfrac{1}{3}\) \(+\) \(\dfrac{5}{9}\) \(=\) \(\dfrac{3}{9}\) \(+\) \(\dfrac{5}{9}\) \(=\) \(\dfrac{3+5}{9}\) \(=\) \(\dfrac{8}{9}\)
\(\dfrac{1}{7}\) \(-\) \(\dfrac{1}{9}\) \(=\) \(\dfrac{9}{63}\) \(-\) \(\dfrac{7}{63}\) \(=\) \(\dfrac{9-7}{63}\) \(=\) \(\dfrac{2}{63}\)
\(\dfrac{1}{9}\) \(\times\) \(\dfrac{9}{3}\) \(=\) \(\dfrac{1\times9}{9\times3}\) \(=\) \(\dfrac{1}{3}\)
\(\dfrac{1}{3}\) \(\div\) \(\dfrac{1}{7}\) \(=\) \(\dfrac{1}{3}\) \(\times\) \(\dfrac{7}{1}\) \(=\) \(\dfrac{1\times7}{3\times1}\) \(=\) \(\dfrac{7}{3}\)
\(3\) \(\div\) \(\dfrac{5}{9}\) \(=\) \(\dfrac{3}{1}\) \(\div\) \(\dfrac{5}{9}\) \(=\dfrac{3}{1}\times\dfrac{9}{5}=\dfrac{3\times9}{1\times5}=\dfrac{27}{5}\)
\(3\times\dfrac{5}{9}=\dfrac{3}{1}\times\dfrac{5}{9}=\dfrac{3\times5}{1\times9}=\dfrac{5}{3}\)
\(9+\dfrac{9}{3}=\dfrac{9}{1}+\dfrac{9}{3}=\dfrac{27}{3}+\dfrac{9}{3}=\dfrac{27+9}{3}=\dfrac{36}{3}=12\)
\(4\) \(-\dfrac{2}{4}=\dfrac{4}{1}-\dfrac{2}{4}=\dfrac{16}{4}-\dfrac{2}{4}=\dfrac{14}{4}=\dfrac{7}{2}\)
AH
Akai Haruma
Giáo viên
30 tháng 11 2023
Lời giải:
a. $x(3x+1)+(x-1)^2-(2x+1)(2x-1)=0$
$\Leftrightarrow (3x^2+x)+(x^2-2x+1)-(4x^2-1)=0$
$\Leftrightarrow 3x^2+x+x^2-2x+1-4x^2+1=0$
$\Leftrightarrow (3x^2+x^2-4x^2)+(x-2x)+(1+1)=0$
$\Leftrightarrow -x+2=0$
$\Leftrightarrow x=2$
b.
$(x+1)^3+(2-x)^3-9(x-3)(x+3)=0$
$\Leftrightarrow [(x+1)+(2-x)][(x+1)^2-(x+1)(2-x)+(2-x)^2]-9(x-3)(x+3)=0$
$\Leftrightarrow 3[x^2+2x+1-(x-x^2+2)+(x^2-4x+4)]-9(x-3)(x+3)=0$
$\Leftrightarrow 3(3x^2-3x+3)-9(x^2-9)=0$
$\Leftrightarrow 9(x^2-x+1)-9(x^2-9)=0$
$\Leftrightarrow 9(x^2-x+1-x^2+9)=0$
$\Leftrightarrow 9(-x+10)=0$
$\Leftrightarrow -x+10=0\Leftrightarrow x=10$
AH
Akai Haruma
Giáo viên
30 tháng 11 2023
c.
$(x-1)^3-(x+3)(x^2-3x+9)+3x^2=25$
$\Leftrightarrow (x^3-3x^2+3x-1)-(x^3+3^3)+3x^2=25$
$\Leftrightarrow x^3-3x^2+3x-1-x^3-27+3x^2=25$
$\Leftrightarrow (x^3-x^3)+(-3x^2+3x^2)+3x-28=25$
$\Leftrightarrow 3x-28=25$
$\Leftrightarrow x=\frac{53}{3}$
d.
$(x+2)^3-(x+1)(x^2-x+1)-6(x-1)^2=23$
$\Leftrightarrow (x^3+6x^2+12x+8)-(x^3+1)-6(x^2-2x+1)=23$
$\Leftrightarrow x^3+6x^2+12x+8-x^3-1-6x^2+12x-6=23$
$\Leftrightarrow (x^3-x^3)+(6x^2-6x^2)+(12x+12x)+(8-1-6)=23$
$\Leftrightarrow 24x+1=23$
$\Leftrgihtarrow 24x=22$
$\Leftrightarrow x=\frac{11}{12}$
TA
2 tháng 3 2021
1) `x^2+4-2(x-1)=(x-2)^2`
`<=>x^2+4-2x+2=x^2-4x+4`
`<=>-2x+2=-4x`
`<=>2x=-2`
`<=>x=-1`
.
2) ĐKXĐ: `x \ne \pm 3`
`(x+3)/(x-3)-(x-1)/(x+3)=(x^2+4x+6)/(x^2-9)`
`<=>(x+3)^2-(x-1)(x-3)=x^2+4x+6`
`<=>x^2+6x+9-x^2+4x-3=x^2+4x+6`
`<=>10x+6=x^2+4x+6`
`<=>x^2-6x=0`
`<=>x(x-6)=0`
`<=>x=0;x=6`
.
3) ĐKXĐ: `x \ne \pm 3`
`(3x-3)/(x^2-9) -1/(x-3 )= (x+1)/(x+3)`
`<=>(3x-3)-(x+3)=(x+1)(x-3)`
`<=> 2x-6=x^2-2x-3`
`<=>x^2-4x+3=0`
`<=>x^2-x-3x+3=0`
`<=>x(x-1)-3(x-1)=0`
`<=>(x-3)(x-1)=0`
`<=> x=3;x=1`
Vậy...
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 1 2021
\(\left(3^x;3^y;3^z\right)=\left(a;b;c\right)\Rightarrow\left\{{}\begin{matrix}a;b;c>0\\ab+bc+ca=abc\end{matrix}\right.\)
BĐT cần chứng minh trở thành:
\(\dfrac{a^2}{a+bc}+\dfrac{b^2}{b+ca}+\dfrac{c^2}{c+ab}\ge\dfrac{a+b+c}{4}\)
Thật vậy, ta có:
\(VT=\dfrac{a^3}{a^2+abc}+\dfrac{b^3}{b^2+abc}+\dfrac{c^3}{c^2+abc}\)
\(VT=\dfrac{a^3}{\left(a+b\right)\left(a+c\right)}+\dfrac{b^3}{\left(a+b\right)\left(b+c\right)}+\dfrac{c^3}{\left(a+c\right)\left(b+c\right)}\)
Áp dụng AM-GM:
\(\dfrac{a^3}{\left(a+b\right)\left(a+c\right)}+\dfrac{a+b}{8}+\dfrac{a+c}{8}\ge\dfrac{3a}{4}\)
Làm tương tự với 2 số hạng còn lại, cộng vế với vế rồi rút gọn, ta sẽ có đpcm
TA
2
1 tháng 3 2021
1) ĐKXĐ: \(x\notin\left\{-2;2\right\}\)
Ta có: \(\dfrac{x-1}{x+2}-\dfrac{9}{x^2-4}=\dfrac{-3}{x-2}\)
\(\Leftrightarrow\dfrac{\left(x-1\right)\left(x-2\right)}{\left(x+2\right)\left(x-2\right)}-\dfrac{9}{\left(x-2\right)\left(x+2\right)}=\dfrac{-3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\)
Suy ra: \(x^2-3x+2-9=-3x-6\)
\(\Leftrightarrow x^2-3x-7+3x+6=0\)
\(\Leftrightarrow x^2-1=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\left(nhận\right)\\x=-1\left(nhận\right)\end{matrix}\right.\)
Vậy: S={1;-1}
2)
Sửa đề: \(\dfrac{3x-3}{x^2-9}-\dfrac{1}{x-3}=\dfrac{x+1}{x+3}\)
ĐKXĐ: \(x\notin\left\{3;-3\right\}\)
Ta có: \(\dfrac{3x-3}{x^2-9}-\dfrac{1}{x-3}=\dfrac{x+1}{x+3}\)
\(\Leftrightarrow\dfrac{3x-3}{\left(x-3\right)\left(x+3\right)}-\dfrac{x+3}{\left(x-3\right)\left(x+3\right)}=\dfrac{\left(x+1\right)\left(x-3\right)}{\left(x+3\right)\left(x-3\right)}\)
Suy ra: \(3x-3-x-3=x^2-3x+x-3\)
\(\Leftrightarrow x^2-2x-3=2x-6\)
\(\Leftrightarrow x^2-2x-3-2x+6=0\)
\(\Leftrightarrow x^2-4x+3=0\)
\(\Leftrightarrow x^2-x-3x+3=0\)
\(\Leftrightarrow x\left(x-1\right)-3\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\left(nhận\right)\\x=3\left(loại\right)\end{matrix}\right.\)
Vậy: S={1}
1 tháng 3 2021
`1)(x-1)/(x+2)-9/(x^2-4)=-3/(x-2)(x ne 2)`
`<=>x^2-3x+2-9=-3x-6`
`<=>x^2-1=0`
`<=>x=+-1`
AH
Akai Haruma
Giáo viên
16 tháng 12 2021
Ý bạn là \(f(x)=\frac{1}{9+x^2}+\frac{3}{9+x^2}\) hay thế nào? Bạn cần viết đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo để được hỗ trợ tốt hơn).
T
1
26 tháng 7 2023
`@` `\text {Ans}`
`\downarrow`
`1)`
\(x+\dfrac{1}{2}=\dfrac{5}{3}\)
`\Rightarrow` \(x=\dfrac{5}{3}-\dfrac{1}{2}\)
`\Rightarrow`\(x=\dfrac{7}{6}\)
Vậy, `x =`\(\dfrac{7}{6}\)
`2)`
\(\dfrac{3}{5}-x=\dfrac{1}{3}\)
`\Rightarrow`\(x=\dfrac{3}{5}-\dfrac{1}{3}\)
`\Rightarrow`\(x=\dfrac{4}{15}\)
Vậy, `x =`\(\dfrac{4}{15}\)
`3)`
\(\dfrac{3}{4}+x=\dfrac{7}{2}\)
`\Rightarrow`\(x=\dfrac{7}{2}-\dfrac{3}{4}\)
`\Rightarrow`\(x=\dfrac{11}{4}\)
Vậy, \(x=\dfrac{11}{4}\)
`4)`
\(x-\dfrac{4}{3}=\dfrac{7}{9}\)
`\Rightarrow`\(x=\dfrac{7}{9}+\dfrac{4}{3}\)
`\Rightarrow`\(x=\dfrac{19}{9}\)
Vậy, `x=`\(\dfrac{19}{9}\)
`5)`
\(x-\dfrac{5}{6}=\dfrac{7}{3}\)
`\Rightarrow`\(x=\dfrac{7}{3}+\dfrac{5}{6}\)
`\Rightarrow x =`\(\dfrac{19}{6}\)
Vậy, `x=`\(\dfrac{19}{6}\)
`6)`
\(x-\dfrac{1}{5}=\dfrac{9}{10}\)
`\Rightarrow x=`\(\dfrac{9}{10}+\dfrac{1}{5}\)
`\Rightarrow x=`\(\dfrac{11}{10}\)
Vậy, `x=`\(\dfrac{11}{10}\)
13 tháng 2 2016
1.1 Hình vuông có tối đa 4 góc vậy 4 hình vuông có tối đa 20 góc. S
2.1 hình vuông có tối đa 4 góc vậy 4 hình vuông có tối đa 16 góc. Đ
3. 1 hình vuông có tối thiểu 4 góc vậy 4 hình vuông có tối thiểu 16 góc. Đ
4.1 hình vuông có tối thiểu 1 góc vậy 4 hình vuông có tối thiểu 16 góc. S
Nhiêu đó hết tài năng rồi, mình mới lớp 3 thôi.
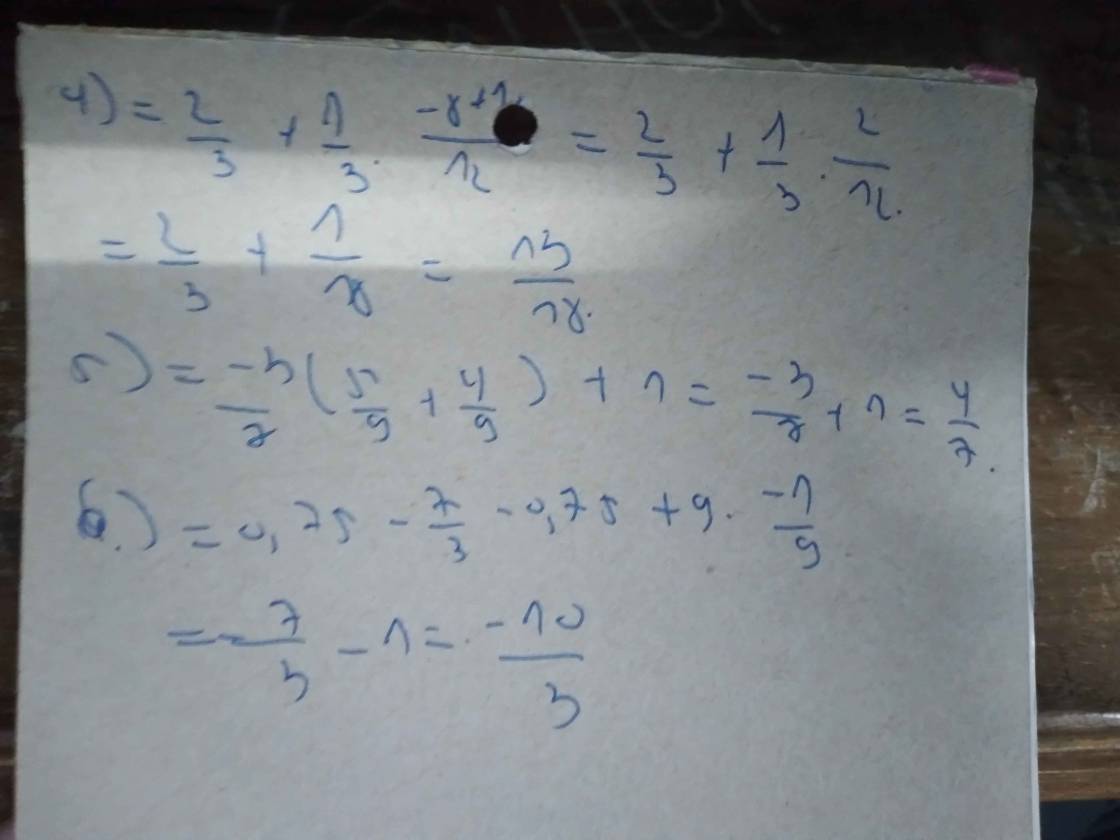
Ta có: \(9.\left(\frac{-1}{27}\right)+\frac{1}{3}\)
\(=\left(\frac{-1}{3}\right)+\frac{1}{3}\)
= 0
9 x ( -1/3 ) ^3 + 1/3
= 9 x ( -1/27 ) + 1/3
= - 1/3 + 1/3
= 0