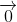Cho hình bình hành ABCD có tâm O. Chứng minh rằng :
a) \(\overrightarrow{CO}-\overrightarrow{OB}=\overrightarrow{BA}\)
b) \(\overrightarrow{AB}-\overrightarrow{BC}=\overrightarrow{DB}\)
c) \(\overrightarrow{DA}-\overrightarrow{DB}=\overrightarrow{OD}-\overrightarrow{OC}\)
d) \(\overrightarrow{DA}-\overrightarrow{DB}+\overrightarrow{DC}=\overrightarrow{0}\)



a) Ta có, theo quy tắc ba điểm của phép trừ:
Mặt khác,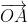 =
=  (2)
(2)
Từ (1) và (2) suy ra:
b) Ta có :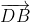 =
= 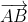 -
- 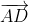 (1)
(1)
Từ (1) và (2) cho ta:
c) Ta có :
Từ (1), (2), (3) suy ra đpcm.
d)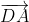 -
- 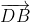 +
+ 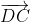 = (
= ( 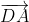 -
- 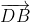 ) +
) + 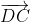 =
= 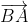 +
+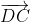 =
= 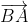 +
+ 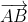 ( vì
( vì 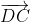 =
= 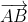 ) =
) =