TÌM x biết (x2-1).(x2-4).(x2-9).(x2-10) \(\ge\)0 với x thuộc Z
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(a,5x\left(x^2-9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x^2=9\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\\ b,3\left(x+3\right)-x^2-3x=0\\ \Leftrightarrow3\left(x+3\right)-x\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left(3-x\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\\ c,x^2-9x-10=0\\ \Leftrightarrow x^2+x-10x-10=0\\ \Leftrightarrow x\left(x+1\right)-10\left(x+1\right)=0\\ \Leftrightarrow\left(x-10\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\\x=10\end{matrix}\right.\)
a, 5\(x\)(\(x^2\) - 9) = 0
\(\left[{}\begin{matrix}x=0\\x^2-9=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\)
Vậy \(x\) \(\in\) { -3; 0; 3}
b, 3.(\(x+3\)) - \(x^2\) - 3\(x\) = 0
3.(\(x+3\)) - \(x\).( \(x\) + 3) = 0
(\(x+3\))( 3 - \(x\)) = 0
\(\left[{}\begin{matrix}x=-3\\x=3\end{matrix}\right.\)
Vậy \(x\) \(\in\){ -3; 3}
c, \(x^2\) - 9\(x\) - 10 = 0
\(x^2\) + \(x\) - 10\(x\) - 10 = 0
\(x.\left(x+1\right)\) - 10.( \(x-1\)) = 0
(\(x+1\))(\(x-10\)) = 0
\(\left[{}\begin{matrix}x+1=0\\x-10=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-1\\x=10\end{matrix}\right.\)
Vậy \(x\) \(\in\){ -1; 10}

b)x2-2x+1=4
⇔(x-1)2=4
\(\Leftrightarrow\left[{}\begin{matrix}x-1=2\\x-1=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-1\end{matrix}\right.\)
c)x2-4x+4=9
⇔ (x-2)2=9
\(\Leftrightarrow\left[{}\begin{matrix}x-2=3\\x-2=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-1\end{matrix}\right.\)
d)4x2-4x+1=4
⇔ (2x-1)2=4
\(\Leftrightarrow\left[{}\begin{matrix}2x-1=4\\2x-1=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=\dfrac{-3}{2}\end{matrix}\right.\)
e)x2-2x-8=0
⇔ x2-4x+2x-8=0
⇔ x(x-4)+2(x-4)=0
⇔(x-4)(x+2)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-2\end{matrix}\right.\)
f)9x2-6x-8=0
⇔ 9x2-12x+6x-8=0
⇔ 3x(3x-4)+2(3x-4)=0
⇔ (3x-4)(3x+2)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{4}{3}\\x=\dfrac{-2}{3}\end{matrix}\right.\)
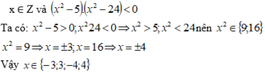
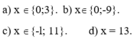
(x2-1).(x2-4).(x2-9).(x2-10) \(\ge\)0 => cả 4 số (x2-1); (x2-4); (x2-9); (x2-10) đều không âm hoặc không dương hoặc có 2 số không dương và 2 số không âm
Nhận xét: x2-1 > x2-4 > x2-9 > x2-10 ( Vì -1 > -4 > -9 > -10). Do đó:
+) Nếu 4 số cùng không âm thì x2-1 > x2-4 > x2-9 > x2-10 \(\ge\) 0 => x2 \(\ge\) 10 . Vì x nguyên => x = 4; 5 ; 6;....hoặc -4;-5;-6;...
+) Nếu 4 số cùng không dương thì 0 \(\ge\)x2-1 > x2-4 > x2-9 > x2-10 => x2 - 1 \(\le\) 0 => x2 \(\le\) 1 Mà x2 \(\ge\) 0 nên x2 = 1 => x =1 hoặc x = -1
+) Nếu có 2 số không âm và số không dương thì x2-1 > x2-4 \(\ge\) 0 \(\ge\) x2-9 > x2-10
=> x2 \(\ge\) 4 và x2 \(\le\) 9. Vì x nguyên => x2 = 4 hoặc 9 => x = -2; 2; hoặc -3; 3
Vậy với mọi x nguyên đều thỏa mãn y/c
Các bn coi m làm đúng hg nhak
Giải
(x2+1)(x2-10)< 0 khi x2+1 và x2-10 khác dấu
Mà x2+1 > x2-10 nên x2+1> 0 và x2-10<0, ta có
x2+1 > 0 => x2>-1
x2-10 < 0 => x2< 10
=> -1 < x2 < 10
=>x = +-1 hoặc +-2 hoặc +-3