K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
18 tháng 8 2021
a) x2 + x = 0
=> x( x+ 1 ) = 0
=> x = 0
hoặc x = -1
b) b, (x-1)x+2 = (x-1)x+4
=> x + 2 = x + 4
=> 0x = 2 ( ktm)
Vậy ko có giá trị x nào thoả mãn đk
18 tháng 8 2021
d) Ta có: x-1/x+5 = 6/7
=>(x-1).7 = (x+5).6
=>7x-7 = 6x+ 30
=> 7x-6x = 7+30
=> x = 37
Vậy x = 37
e, x2/ 6= 24/25
=> x2 . 25 = 6 . 24
⇒x2.25=144⇒x2.25=144
⇒x2=144÷25⇒x2=144÷25
⇒x2=5,76=2,42=(−2,42)⇒x2=5,76=2,42=(−2,42)
⇒x∈{2,4;−2,4}⇒x∈{2,4;−2,4}
Vậy x∈{2,4;−2,4}
BT
0
NQ
2
TT
23 tháng 2 2020
\(8\cdot3^x+3\cdot2^x-6^x=24\)
\(\Leftrightarrow8\cdot3^x+3\cdot2^x-2^x\cdot3^x=24\)
\(\Leftrightarrow2^3\cdot3\left(3^{x-1}+2^{x-3}-2^{x-3}\cdot3^{x-1}\right)=24\)
\(\Leftrightarrow3^{x-1}+2^{x-3}-2^{x-3}\cdot3^{x-1}=1\)
Đặt \(3^{x-1}=a,2^{x-3}=b\)
Khi đó ta có : \(a+b+ab-1=0\)
\(\Leftrightarrow\left(a-1\right)\left(1-b\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}a-1=0\\1-b=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}a=1\\b=1\end{cases}}\)
+) Với \(a=1\Rightarrow3^{x-1}=1\)
\(\Rightarrow x-1=0\Rightarrow x=1\)
+) Với \(b=1\Rightarrow2^{x-3}=1\)
\(\Rightarrow x-3=0\Rightarrow x=3\)
Vậy : \(x\in\left\{3,1\right\}\)
XO
23 tháng 2 2020
Ta có : 8.3x + 3.2x - 6x = 24
=> 8.3x + 3.2x - 3x.2x = 24
=> 8.3x + 2x(3 - 3x) = 24
=> -24 + 8.3x + 2x(3 - 3x) = 24 - 24
=> -8(3 - 3x) + 2x(3 - 3x) = 0
=> (-8 + 2x)(3 - 3x) = 0
=> \(\orbr{\begin{cases}-8+2^x=0\\3-3^x=0\end{cases}\Rightarrow\orbr{\begin{cases}2^x=8\\3^x=3\end{cases}\Rightarrow}\orbr{\begin{cases}x=3\\x=1\end{cases}}}\)
Vậy \(x\in\left\{3;1\right\}\)
VK
1
YN
27 tháng 4 2022
a)
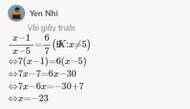
b) \(\dfrac{x^2}{6}=\dfrac{24}{25}\)
\(\Leftrightarrow\left(5x\right)^2=144\)
\(\Leftrightarrow\left(5x\right)^2=12^2\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=12\\5x=-12\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{12}{5}\\x=-\dfrac{12}{5}\end{matrix}\right.\)
c) \(\dfrac{x-2}{x-1}=\dfrac{x+4}{x+7}\)
\(\Leftrightarrow\left(x-2\right)\left(x+7\right)=\left(x-1\right)\left(x+4\right)\)
\(\Leftrightarrow x^2+5x-14=x^2+3x-4\)
\(\Leftrightarrow2x=10\)
\(\Leftrightarrow x=5\)
21 tháng 10 2015
\(\frac{x^2}{6}=\frac{24}{25}\)
=> x2 . 25 = 6 . 24
=> x2 . 25 = 144
=> x2 = 5,76
=> x = \(\sqrt{5,76}\) = 2,4
LT
1
12 tháng 10 2015
a)x-2/5=3/8
(x-2).8=5.3
x-2=5.3:8
x-2=1
=>x=3
b,c )tương tự