Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Theo định lí Bezout ta có:
\(f\left(-5\right)=3.\left(-5\right)^2-5a+27=2\)
\(\Leftrightarrow75-5a+27=2\)
\(\Leftrightarrow102-5a=2\)
\(\Rightarrow a=20\)
b) \(x^3+ax^2+x+b=\left(x^2-x+2\right).\left(x+m\right)\)(Trong đó m là số nguyên)
\(\Leftrightarrow x^3+ax^2+x+b=x^3+x^2.\left(m-1\right)-mx+2m\)
Sử dụng phương pháp đồng nhất hệ số ta có:
\(\hept{\begin{cases}ax^2=m-1\\x=-mx\\2m=b\end{cases}}\Leftrightarrow\hept{\begin{cases}a=m-1\\m=-1\\2m=b\end{cases}}\Leftrightarrow\hept{\begin{cases}a=-2\\b=-2\end{cases}}\Leftrightarrow a=b=-2\)

Bài 209 : đăng tách ra cho mn cùng làm nhé
a,sửa đề : \(A=\left(3x+1\right)^2-2\left(3x+1\right)\left(3x+5\right)+\left(3x+5\right)^2\)
\(=\left(3x+1-3x-5\right)^2=\left(-4\right)^2=16\)
b, \(B=\left(3+1\right)\left(3^2+1\right)\left(3^4+1\right)...\left(3^{32}+1\right)\)
\(2B=\left(3^2-1\right)\left(3^2+1\right)\left(3^4+1\right)...\left(3^{32}+1\right)=\left(3^{32}-1\right)\left(3^{32}+1\right)\)
\(2B=3^{64}-1\Rightarrow B=\frac{3^{64}-1}{2}\)
c, \(C=\left(a+b-c\right)^2+\left(a-b+c\right)^2-2\left(b-c\right)^2\)
\(=2\left(a-b+c\right)^2-2\left(b-c\right)^2=2\left[\left(a-b+c\right)^2-\left(b-c\right)^2\right]\)
\(=2\left(a-b+c-b+c\right)\left(a-b+c+b-c\right)=2a\left(a-2b+2c\right)\)

Bài 2:
\(=\dfrac{3x^4+3x^2+x^3+x-3x^2-3+5x-5}{x^2+1}\)
\(=3x^2+x-3+\dfrac{5x-5}{x^2+1}\)
Bài 3:
\(\dfrac{A}{B}=\dfrac{2x^3-x^2-x+1}{x^2-2x}\)
\(=\dfrac{2x^3-4x^2+3x^2-6x+5x+1}{x^2-2x}\)
\(=2x^2+3+\dfrac{5x+1}{x^2-2x}\)
=>\(2x^3-x^2-x+1=\left(x^2-2x\right)\left(2x^2+3\right)+5x+1\)

a) \(\frac{2x^3+x^2+x+6}{x^2-x+2}=\frac{\left(2x+3\right)\left(x^2-x+2\right)}{x^2-x+2}=2x+3\)
b) \(\frac{x}{x-3}-\frac{5x^2+27}{x^2-9}+\frac{x-9}{x+3}\)
\(=\frac{x}{x-3}-\frac{5x^2+27}{\left(x-3\right)\left(x+3\right)}+\frac{x-9}{x+3}\)
\(=\frac{x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}-\frac{5x^2+27}{\left(x-3\right)\left(x+3\right)}+\frac{\left(x-9\right)\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}\)
\(=\frac{x^2+3x}{\left(x-3\right)\left(x+3\right)}-\frac{5x^2+27}{\left(x-3\right)\left(x+3\right)}+\frac{x^2-12x+27}{\left(x-3\right)\left(x+3\right)}\)
\(=\frac{x^2+3x-\left(5x^2+27\right)+x^3-12x+27}{\left(x-3\right)\left(x+3\right)}\)
\(=\frac{-3x^2-9x}{\left(x-3\right)\left(x+3\right)}\)
\(=\frac{-3x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}\)
\(=\frac{-3x}{x-3}\)

Bài 1:
1 (x+3)2=x2+6x+9
2
a, 2x2(3x-5x3)+10x5-5x3=6x3-10x5+10x5-5x3=x3
b, (x+3)(x2-3x+9)+(x-9)(x+3)=(x3+27)+(x2-6x-27)=x3+x2-6x
Bài 2:
a, x2-25x=0
\(\Leftrightarrow x\left(x-25\right)=0\)
\(\Leftrightarrow\begin{cases}x=0\\x-25=0\end{cases}\)
\(\Leftrightarrow\begin{cases}x=0\\x=25\end{cases}\)
b, (4x-1)2-9=0
\(\Leftrightarrow\left(4x-1-3\right)\left(4x-1+3\right)=0\)
\(\Leftrightarrow\left(4x-4\right)\left(4x+2\right)=0\)
\(\Leftrightarrow4\left(x-1\right)2\left(2x+1\right)=0\)
\(\Leftrightarrow8\left(x-1\right)\left(2x+1\right)=0\)
\(\Leftrightarrow\begin{cases}x-1=0\\2x+1=0\end{cases}\)
\(\Leftrightarrow\begin{cases}x=1\\x=\frac{-1}{2}\end{cases}\)
Bài 3:
a, 3x2-18x+27=3(x2-6x+9)=3(x-3)2
b, xy-y2-x+y=y(x-y)-(x-y)=(y-1)(x-y)
c, x2-5x-6=x2-6x+x-6=x(x-6)+(x-6)=(x+1)(x-6)
Bài 4:
a, ( 12x3y3-3x2y3+4x2y4):6x2y3=(12x3y3:6x2y3)-(3x2y3:6x2y3)+(4x2y4:6x2y3)
=2x-1/2 + 2/3y
b, bạn ơi mình không biết cách vẽ đường kẻ để chia ý , nếu bạn biết thì chỉ cho mình rồi mình làm cho
Bài 5 :
b, A = x(2x-3)
A= 2x2-3x
A= 2(x2-3/2x)
A= 2(x2-2x3/4+9/16-9/16)
A=2[(x-3/4)2-9/16]
A=2(x-3/4)2-9/8
A=2(x-3/4)2+(-9/8)
Vì (x-3/4)2 \(\ge\)0 \(\forall x\)
-> 2(x-3/4)2 \(\ge0\forall x\)
-> 2(x-3/4)2+(-9/8)\(\ge-\frac{9}{8}\forall x\)
Vậy MinA= -9/8
Bài 1:
1. Khai triển hằng đẳng thức
(x+3)2 = x2+6x+9
2. Thực hiện phép tính
a) 2x2(3x-5x3)+10x5-5x3
=6x3-10x5+10x5-5x3
=x3
b)(x+3)(x2-3x+9)+(x-9)(x+3)
=(x3+27)+(x2+3x-9x-27)
=x3+27+x2+3x-9x-27
=x3+x2-6x
Bài 2:
a) x2-25x=0
\(\Leftrightarrow\)x(x-25)=0
\(\Leftrightarrow\) \(\left[\begin{matrix}x=0\\x-25=0\end{matrix}\right.\)
\(\Leftrightarrow\left[\begin{matrix}x=0\\x=25\end{matrix}\right.\)
Vậy x=0 hoặc x=25
b)(4x-1)2 - 9=0
\(\Leftrightarrow\)(4x-1+3)(4x-1-3)=0
\(\Leftrightarrow\)(4x+2)(4x-4)=0
\(\Leftrightarrow\)2(2x+1)(2x-2)=0
\(\Leftrightarrow\left[\begin{matrix}2x+1=0\\2x-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[\begin{matrix}x=\frac{-1}{2}\\x=1\end{matrix}\right.\)
Vậy x=1 hoặc x=\(\frac{-1}{2}\)
Bài 3:
a) 3x2-18x+27
=3(x2-6x+9)
=3(x-3)2
b) xy-y2-x+y
=(xy-y2)-(x-y)
=y(x-y)-(x-y)
=(x-y)(y-1)
c) x2-5x-6
=x2-6x+x-6
=(x2-6x)+(x-6)
=x(x-6)+(x-6
=(x-6)(x+1)
Bài 4:
a) (12x3y3-3x2y3+4x2y4) : 6x2y3
=x2y3(12x-3+4y): 6x2y3
=(12x-3+4y) : 6
= (12x : 6)-(3 : 6)+(4y : 6)
=2x-\(\frac{1}{2}\)+\(\frac{2y}{3}\)
b) (6x3-19x2+23x-12) : (2x-3)
=(3x2-5x+4)(2x-3) : (2x-3)
=3x2-5x+4

Duy Nguyễn, Duy Nguyễn, Duy Nguyễn, Duy Nguyễn, Duy nguyễn, Duy Nguyễn, Duy Nguyễn, Duy Nguyễn, Duy Nguyễn
giúp bn ấy vs

Áp dụng HĐT thôi bạn =)
a) ( a + b )2 + ( a - b )2 - 6a2b
= a2 + 2ab + b2 + a2 - 2ab + b2 - 6a2b
= 2a2 + 2b2 - 6a2b
= 2( a2 + b2 - 3a2b )
b) ( a + 3 )3 - ( a - b )3 - 6a2b
=( a3 + 3a2b + 3ab2 + b3 ) - ( a3 - 3a2b + 3ab2 - b3 ) - 6a2b
= a3 + 3a2b + 3ab2 + b3 - a3 + 3a2b - 3ab2 + b3 - 6a2b
= 2b3

\(a,2x^2-2xt-5x+5y\)
\(=\left(2x^2-5x\right)-\left(2xy-5y\right)\)
\(=x\left(2x-5\right)-y\left(2x-5\right)\)
\(=\left(2x-5\right)\left(x-y\right)\)
\(b,8x^2+4xy-2ax-ay\)
\(=\left(8x^2-2ax\right)+\left(4xy-ay\right)\)
\(=2x\left(4x-a\right)+y\left(4x-a\right)\)
\(=\left(4x-a\right)\left(2x+y\right)\)
\(c,x^3-4x^2+4x\)
\(=x^3-2x^2-2x^2+4x\)
\(=\left(x^3-2x^2\right)-\left(2x^2-4x\right)\)
\(=x^2\left(x-2\right)-2x\left(x-2\right)\)
\(=\left(x-2\right)\left(x^2-2x\right)=x\left(x-2\right)\left(x-2\right)\)
\(=x\left(x-2\right)^2\)
\(d,2xy-x^2-y^2+16\)
\(=-\left(x^2-2xy+y^2-16\right)\)
\(=-\left[\left(x-y\right)^2-4^2\right]\)
\(=-\left(x-y-4\right)\left(x-y+4\right)\)
\(e,x^2-y^2-2yz-z^2\)
\(=x^2-\left(y^2+2yz+z^2\right)\)
\(=x^2-\left(y+z\right)^2=\left(x-y-z\right)\left(x+y+z\right)\)


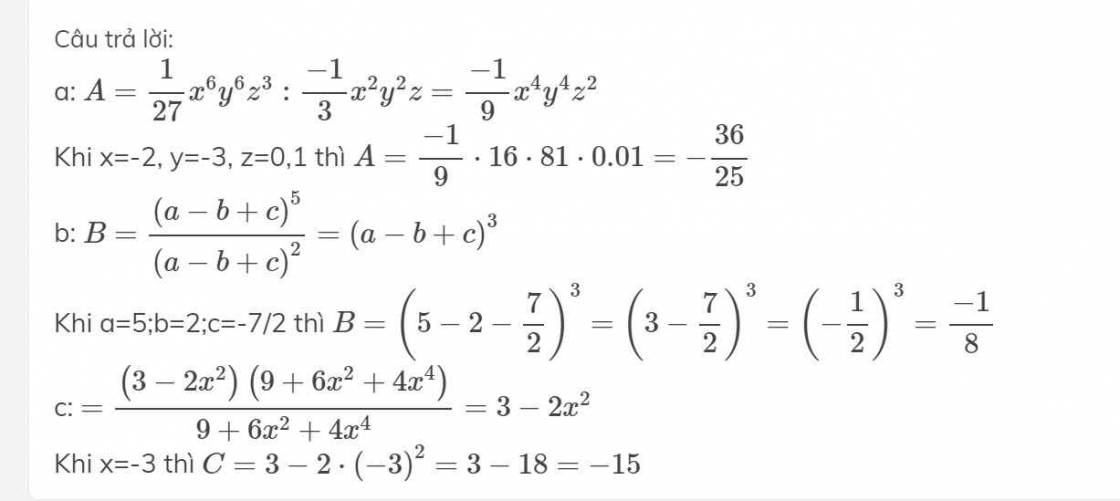
a) Phân tích a 2 – 6ab + 9 b 2 = ( a – 3 b ) 2 ; thực hiện phép chia được kết quả a – 3b.
b) Phân tích a 3 + 9 a 2 b + 27a b 2 – 27 b 3 = ( a – 3 b ) 3 ; thực hiện phép chia được kết quả a – 3b.