
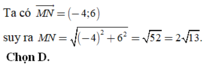
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

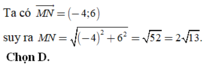

Dù hiểu đề là tìm tọa độ điểm $M$ nhưng lần sau bạn vẫn cần viết đầy đủ yêu cầu của đề ra nhé.
Lời giải:
Giả sử tọa độ điểm $M$ là $(a,b)$
\(\overrightarrow{MA}=(-3-a; 3-b)\)
\(\overrightarrow{BC}=(1;-9)\)
\(\overrightarrow{CM}=(a-2; b+5)\)
Để $2\overrightarrow{MA}-\overrightarrow{BC}=4\overrightarrow{CM}$ thì:
\(\Leftrightarrow 2(-3-a; 3-b)-(1;-9)=4(a-2; b+5)\)
\(\Leftrightarrow \left\{\begin{matrix} 2(-3-a)-1=4(a-2)\\ 2(3-b)+9=4(b+5)\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a=\frac{1}{6}\\ b=\frac{-5}{6}\end{matrix}\right.\)

Hok nhanh phết, chưa j đã đến phần toạ độ vecto r
1/ \(\overrightarrow{MB}=\left(x_B-x_M;y_B-y_M\right)=\left(2-x_M;3-y_M\right)\)
\(\Rightarrow2\overrightarrow{MB}=\left(4-2x_M;6-2y_M\right)\)
\(\overrightarrow{3MC}=\left(3x_C-3x_M;3y_C-3y_M\right)=\left(-3-3x_M;6-3y_M\right)\)
\(\Rightarrow2\overrightarrow{MB}+3\overrightarrow{MC}=\left(4-2x_M-3-3x_M;6-2y_M+6-3y_M\right)=0\)
\(\Leftrightarrow\left(1-5x_M;12-5y_M\right)=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}1-5x_M=0\\12-5y_M=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_M=\frac{1}{5}\\y_M=\frac{12}{5}\end{matrix}\right.\Rightarrow M\left(\frac{1}{5};\frac{12}{5}\right)\)
2/ \(\overrightarrow{m}=2\left(1;2\right)+3\left(3;4\right)=\left(2+9;4+12\right)=\left(11;16\right)\)
3/ \(\overrightarrow{AB}=\left(x_B-x_A;y_B-y_A\right)=\left(-5-3;4+2\right)=\left(-8;6\right)\)
\(\overrightarrow{AC}=\left(x_C-x_A;y_C-y_A\right)=\left(\frac{1}{3}-3;0+2\right)=\left(-\frac{8}{3};2\right)\)
\(\Rightarrow x=\frac{\overrightarrow{AB}}{\overrightarrow{AC}}=\frac{\left(-8;6\right)}{\left(-\frac{8}{3};2\right)}=3\)
Câu 4 tương tự
Câu 5 vt lại đề bài nhé bn, nghe nó vô lý sao á, m,n ở đâu ra vậy, cả A,B,C nx

Ta có M N → = − 4 ; 6 suy ra M N = − 4 2 + 6 2 = 52 = 2 13 .
Chọn D