
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

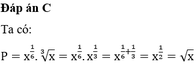

Câu 1 :
Đk: \(x\ge1\)
\(\sqrt{x-1}+\sqrt{2x-1}=5\\ \Leftrightarrow x-1+2\sqrt{\left(x-1\right)\left(2x-1\right)}+2x-1=25\\ \Leftrightarrow2\sqrt{2x^2-3x+1}=27-3x\\ \)
\(\Leftrightarrow\begin{cases}27-3x\ge0\\4\left(2x^2-3x+1\right)=9x^2-162x+729\end{cases}\) \(\Leftrightarrow\begin{cases}x\le9\\x^2-150x+725=0\end{cases}\)
\(\Leftrightarrow\begin{cases}x\le9\\x=145hoặcx=5\end{cases}\)
với x= 5 thoản mãn điều kiện, x=145 loại
Vậy \(S=\left\{5\right\}\)

a) A=x^2+2
b) mình nghĩ x thuộc tập hợp R
c)GTNN của A=1/4 khi x=1/2

a) ĐK: \(x\ge0,x\ne1,x\ne\frac{1}{4}\)
\(A=1+\left(\frac{2x+\sqrt{x}-1}{1-x}-\frac{2x\sqrt{x}-\sqrt{x}+x}{1-x\sqrt{x}}\right)\frac{x-\sqrt{x}}{2\sqrt{x}-1}\)
\(A=1+\left[\frac{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)\left(1-\sqrt{x}\right)}-\frac{\sqrt{x}\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(1-\sqrt{x}\right)\left(x+\sqrt{x}+1\right)}\right]\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{2\sqrt{x}-1}\)
\(A=1+\left[\frac{2\sqrt{x}-1}{1-\sqrt{x}}-\frac{\sqrt{x}\left(2\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(1-\sqrt{x}\right)\left(x+\sqrt{x}+1\right)}\right]\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{2\sqrt{x}-1}\)
\(A=1-\sqrt{x}+\frac{x\left(\sqrt{x}+1\right)}{x+\sqrt{x}+1}\)
\(A=\frac{x+1}{x+\sqrt{x}+1}\)
Để \(A=\frac{6-\sqrt{6}}{5}\Rightarrow\frac{x+1}{x+\sqrt{x}+1}=\frac{6-\sqrt{6}}{5}\)
\(\Rightarrow5x+5=\left(6-\sqrt{6}\right)x+\left(6-\sqrt{6}\right)\sqrt{x}+6-\sqrt{6}\)
\(\Rightarrow\left(1-\sqrt{6}\right)x+\left(6-\sqrt{6}\right)\sqrt{x}+1-\sqrt{6}=0\)
\(\Rightarrow x-\sqrt{6}.\sqrt{x}+1=0\)
\(\Rightarrow\orbr{\begin{cases}\sqrt{x}=\frac{\sqrt{2}+\sqrt{6}}{2}\\\sqrt{x}=\frac{-\sqrt{2}+\sqrt{6}}{2}\end{cases}}\Rightarrow\orbr{\begin{cases}x=2+\sqrt{3}\\x=2-\sqrt{3}\end{cases}}\left(tmđk\right)\)
b) Xét \(A-\frac{2}{3}=\frac{x+1}{x+\sqrt{x}+1}-\frac{2}{3}=\frac{3x+3-2x-2\sqrt{x}-2}{3\left(x+\sqrt{x}+1\right)}\)
\(=\frac{x-2\sqrt{x}+1}{3\left(x+\sqrt{x}+1\right)}=\frac{\left(\sqrt{x}-1\right)^2}{3\left(x+\sqrt{x}+1\right)}\)
Do \(x\ge0,x\ne1,x\ne\frac{1}{4}\Rightarrow\left(\sqrt{x}-1\right)^2>0\)
Lại có \(x+\sqrt{x}+1=\left(\sqrt{x}+\frac{1}{2}\right)+\frac{3}{4}>0\)
Nên \(A-\frac{2}{3}>0\Rightarrow A>\frac{2}{3}\).

2) Ta có:
\(B=x^4+2x^3y-2x^3+x^2y^2-2x^2y-x\left(x+y\right)+2x+3\)
\(=x^4+x^3y-2x^3+x^3y+x^2y^2-2x^2y-x\left(x+y\right)+2x+3\)
\(=\left(x^4+x^3y-2x^3\right)+\left(x^3y+x^2y^2-2x^2y\right)-\left[x\left(x+y\right)-2x\right]+3\)
Do \(x+y-2=0\Rightarrow x+y=2\)
\(\Rightarrow B=\left(x^4+x^3y-2x^3\right)+\left(x^3y+x^2y^2-2x^2y\right)-\left[2x-2x\right]+3\)
\(=x^3.\left(x+y-2\right)+x^2y\left(x+y-2\right)-0+3\)
\(=0+0+3\)
\(=3\)
Vậy \(B=3\)
1) Ta có:
\(A=x^3+x^2y-2x^2-xy-y^2+3y+x-1\)
\(=\left(x^3+x^2y-2x^2\right)-\left(xy+y^2-2y\right)+y+x-1\)
\(=x^2\left(x+y-2\right)-y\left(x+y-2\right)+\left(x+y-2\right)+1\)
\(=0+0+0+1\)
\(=1\)
Vậy \(A=1\)

a: \(F\left(x\right)=x^4+6x^3+2x^2+x-7\)
\(G\left(x\right)=-4x^4-6x^3+2x^2-x+6\)
b: h(x)=f(x)+g(x)
\(=x^4+6x^3+2x^2+x-7-4x^4-6x^3+2x^2-x+6\)
\(=-3x^4+4x^2-1\)
c: Đặt h(x)=0
\(\Leftrightarrow3x^4-4x^2+1=0\)
\(\Leftrightarrow\left(3x^2-1\right)\left(x^2-1\right)=0\)
hay \(x\in\left\{1;-1;\dfrac{\sqrt{3}}{3};-\dfrac{\sqrt{3}}{3}\right\}\)

a) \(4x-7>0\Leftrightarrow4x>7\)\(\Leftrightarrow x>\frac{7}{4}\)
b) \(-5x+8>0\Leftrightarrow5x<8\Leftrightarrow x<\frac{8}{5}\)
c)\(9x-10\le0\Leftrightarrow9x\le10\)\(\Leftrightarrow x\le\frac{10}{9}\)
d) \(\left(x+1\right)^2+4\le x^2+3x+10\)\(\Leftrightarrow x^2-2x+1+4\le x^2+3x+10\)
\(\Leftrightarrow5x\ge-5\Leftrightarrow x\ge-1\)
a,
4x - 7 > 0
↔ 4x > 7
↔ x > \(\dfrac{7}{4}\)
Vậy tập nghiệm của bất phương trình là S = { x / x>\(\dfrac{7}{4}\) }
b,
-5x + 8 > 0
↔ 8 > 5x
↔ \(\dfrac{8}{5}\) > x
Vậy tập nghiệm của bất phương trình là S = { x / \(\dfrac{8}{5}\) > x }
c,
9x - 10 ≤ 0
↔ 9x ≤ 10
↔ x ≤ \(\dfrac{10}{9}\)
Vậy tập nghiệm của bất phương trình là S = { x / x ≤ \(\dfrac{10}{9}\) }
d,
( x - 1 )\(^2\) + 4 ≤ x\(^2\) + 3x + 10
↔ x\(^2\) - 2x +1 +4 ≤ x\(^2\) + 3x + 10
↔ 1 + 4 - 10 ≤ x \(^2\) - x\(^2\) + 3x + 2x
↔ -5 ≤ 5x
↔ -1 ≤ x
Vậy tập nghiệm của bất phương trình là S = { x / -1 ≤ x}

a, Ta có: \(\left|x-\dfrac{2}{7}\right|\ge0\forall x\)
\(\Rightarrow\left|x-\dfrac{2}{7}\right|+0,5\ge0,5\forall x\)
Hay: \(A\ge0,5\forall x\)
=> Min A = 0,5 tại \(\left|x-\dfrac{2}{7}\right|=0\Rightarrow x=\dfrac{2}{7}\)
b, \(B=\left|x-5\right|+\left|x-2\right|=\left|x-5\right|+\left|2-x\right|\ge\left|x-5+2-x\right|\) =3
=> Min B = 3 tại \(\left(x-5\right)\left(2-x\right)>0\)
=)) Làm nốt
c,Tương tự b
=.= hk tốt!!