
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 4:
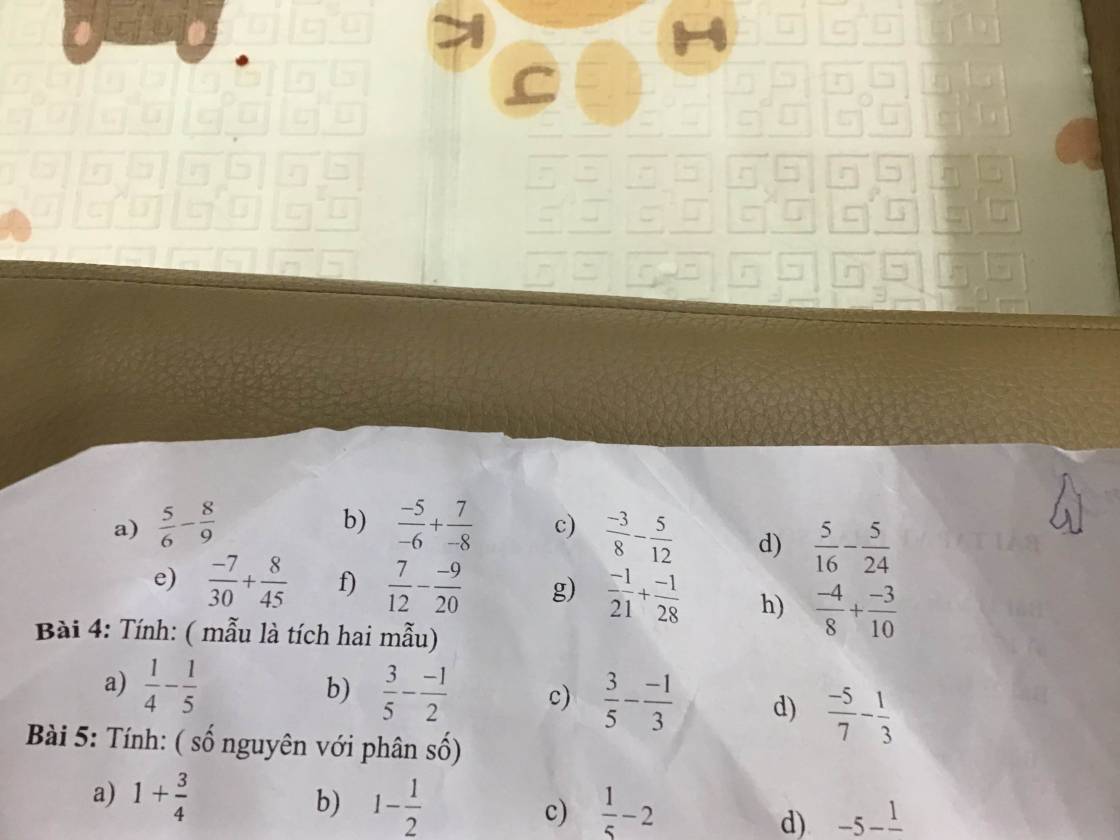
a; \(\dfrac{1}{4}\) - \(\dfrac{1}{5}\) = \(\dfrac{5}{20}\) - \(\dfrac{4}{20}\) = \(\dfrac{1}{20}\)
b; \(\dfrac{3}{5}\) - \(\dfrac{-1}{2}\) = \(\dfrac{6}{10}\) + \(\dfrac{5}{10}\) = \(\dfrac{11}{10}\)
c; \(\dfrac{3}{5}\) - \(\dfrac{-1}{3}\) = \(\dfrac{9}{15}\) + \(\dfrac{5}{15}\) = \(\dfrac{14}{15}\)
d; \(\dfrac{-5}{7}\) - \(\dfrac{1}{3}\)= \(\dfrac{-15}{21}\) - \(\dfrac{7}{21}\)= \(\dfrac{-22}{21}\)
Bài 5
a; 1 + \(\dfrac{3}{4}\) = \(\dfrac{4}{4}\) + \(\dfrac{3}{4}\) = \(\dfrac{7}{4}\) b; 1 - \(\dfrac{1}{2}\) = \(\dfrac{2}{2}\) - \(\dfrac{1}{2}\) = \(\dfrac{1}{2}\)
c; \(\dfrac{1}{5}\) - 2 = \(\dfrac{1}{5}\) - \(\dfrac{10}{5}\) = \(\dfrac{-9}{5}\) d; -5 - \(\dfrac{1}{6}\) = \(\dfrac{-30}{6}\) - \(\dfrac{1}{6}\) = \(\dfrac{-31}{6}\)
e; - 3 - \(\dfrac{2}{7}\)= \(\dfrac{-21}{7}\) - \(\dfrac{2}{7}\)= \(\dfrac{-23}{7}\) f; - 3 + \(\dfrac{2}{5}\) = \(\dfrac{-15}{5}\) + \(\dfrac{2}{5}\)= - \(\dfrac{13}{5}\)
g; - 3 - \(\dfrac{2}{3}\) = \(\dfrac{-9}{3}\) - \(\dfrac{2}{3}\) = \(\dfrac{-11}{3}\) h; - 4 - \(\dfrac{-5}{7}\) = \(\dfrac{-28}{7}\)+ \(\dfrac{5}{7}\) = - \(\dfrac{23}{7}\)

Bài 5:
a. Gọi $d=ƯCLN(n-2, n+1)$
$\Rightarrow n-2\vdots d; n+1\vdots d$
$\Rightarrow (n+1)-(n-2)\vdots d$
$\Rightarrow 3\vdots d\Rightarrow d\in \left\{1; 3\right\}$
Để ps tối giản thì $n-2\not\vdots 3$
$\Leftrightarrow n\neq 3k+2$ với $k$ là số tự nhiên bất kỳ.
b.
Gọi $d=ƯCLN(n+5, n-2)$
$\Rightarrow n+5\vdots d; n-2\vdots d$
$\Rightarrow (n+5)-(n-2)\vdots d$
$\Rightarrow 7\vdots d$
$\Rightarrow d\in \left\{1; 7\right\}$
Để ps tối giản thì $n-2\not\vdots 7$
$\Rightarrow n\neq 7k+2$ với $k$ là số tự nhiên bất kỳ.

Bài 1:
a; 24 ⋮ \(x\); 30 ⋮ \(x\); 48 \(⋮\) \(x\) và \(x\) lớn nhất.
vì 24 \(⋮\) \(x\); 30 ⋮ \(x\); 48 ⋮ \(x\) ⇒ \(x\) \(\in\) ƯC(24; 30; 48)
Vì \(x\) là lớn nhât nên \(x\) \(\in\) ƯCLN(24; 30; 48)
24 = 22.33; 30 = 2.3.5; 48 = 24.3
ƯCLN(24; 30; 48) = 2.3 = 6
⇒ \(x\) = 6
Vậy \(x\) = 6
b; 120 ⋮ \(x\); 180 ⋮ \(x\); 30 ⋮ \(x\)
⇒ \(x\) \(\in\) ƯC(120; 180; 390)
120 = 23.3.5; 180 = 22.32.5; 390 = 2.3.5.13
ƯC(120; 180; 390) = 2.3.5 = 30
⇒ \(x\in\) Ư(30) = {1; 2; 3; 5; 6; 10;15; 30}
Vì 5 ≤ \(x\) ≤ 15 nên \(x\) \(\in\) {5; 6; 10; 15}

Bài 2:
\(\dfrac{12}{-24}=\dfrac{12:12}{-24:12}=\dfrac{1}{-2}\)
\(\dfrac{-39}{75}=\dfrac{-39:3}{75:3}=\dfrac{-13}{25}\)
\(\dfrac{132}{-264}=\dfrac{132:132}{-264:132}=\dfrac{1}{-2}\)
Bài 3:
\(\dfrac{1}{-2}=\dfrac{-1}{2};\dfrac{-3}{-5}=\dfrac{3}{5};\dfrac{2}{-7}=\dfrac{-2}{7}\)
Bài 4:
\(15p=\dfrac{1}{4}h;20p=\dfrac{1}{3}h;45p=\dfrac{3}{4}h;50p=\dfrac{5}{6}h\)

Bài 2:
Chiều cao trung bình của 8 bạn trong tổ 1 là:
115 : 8 = \(\dfrac{115}{8}\) (dm)
Chiều cao trung bình của 10 bạn trong tổ 2 là:
138 : 10 = \(\dfrac{69}{5}\) (dm)
\(\dfrac{115}{8}\) = \(\dfrac{115\times5}{8\times5}\) = \(\dfrac{575}{40}\)
\(\dfrac{69}{5}\) = \(\dfrac{69\times8}{5\times8}\) = \(\dfrac{552}{40}\)
Vì \(\dfrac{575}{40}\) > \(\dfrac{552}{40}\)
Vậy chiều cao trung bình của các bạn tổ 1 lớn hơn chiều cao trung bình của các bạn tổ 2
Bài 3:
a; \(\dfrac{-11}{5}\) < \(\dfrac{-10}{5}\) = -2
\(\dfrac{-7}{4}\) > \(\dfrac{-8}{4}\) = - 2
\(\Rightarrow\) \(\dfrac{-11}{5}\) < \(\dfrac{-7}{4}\)
b; \(\dfrac{2020}{-2021}\) > - 1
\(\dfrac{-2022}{2021}\) < -1
Vậy \(\dfrac{2020}{-2021}\) > \(\dfrac{-2022}{2021}\)

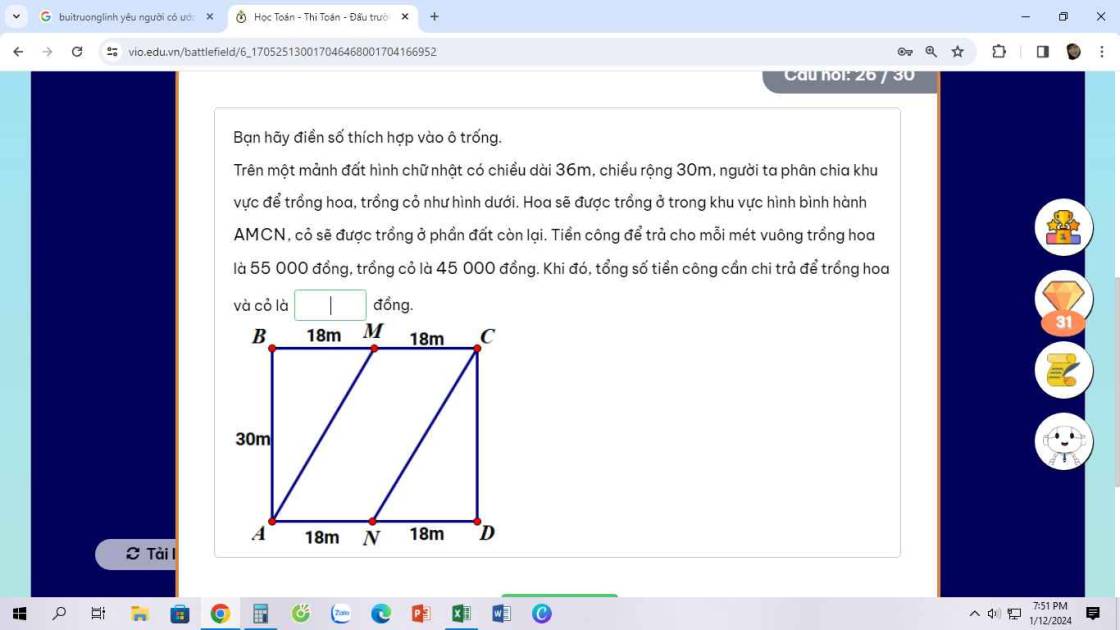
Diện tích mảnh đất là:
\(30\times\left(18+18\right)=1080\left(m^2\right)\)
Diện tích trồng hoa là:
\(30\times18=540\left(m^2\right)\)
Diện tích trồng cỏ là:
\(1080-540=540\left(m^2\right)\)
Tổng tiền cần chi trả là:
\(55000\times540+45000\times540=54000000\) (đồng)
Giải
Diện tích mảnh đất là:
30x(18+18)=1080(m vuông)
Diện tích trồng hoa là:
30x18=540(m vuông)
Diện tích trồng cỏ là:1080-540=540(m vuông)
Tổng số tiền cần chị trả là:
55000x540+45000x540=54000000(đồng)
Chúc bạn học tốt!

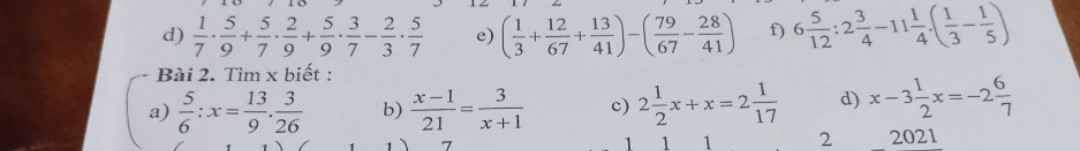





Bài 2:
a) \(\dfrac{5}{6}:x=\dfrac{13}{9}\cdot\dfrac{3}{26}\)
\(\Rightarrow\dfrac{5}{6}:x=\dfrac{3}{9\cdot2}\)
\(\Rightarrow\dfrac{5}{6}:x=\dfrac{1}{6}\)
\(\Rightarrow x=\dfrac{5}{6}:\dfrac{1}{6}\)
\(\Rightarrow x=5\)
b) \(\dfrac{x-1}{21}=\dfrac{3}{x+1}\)
\(\Rightarrow\left(x-1\right)\left(x+1\right)=3\cdot21\)
\(\Rightarrow x^2-x+x-1=63\)
\(\Rightarrow x^2-1=63\)
\(\Rightarrow x^2-64=0\)
\(\Rightarrow x^2-8x+8x-64=0\)
\(\Rightarrow x\left(x-8\right)+8\left(x-8\right)=0\)
\(\Rightarrow\left(x+8\right)\left(x-8\right)=0\)
TH1: `x+8=0=>x=-8`
TH2: `x-8=0=>x=8`
c) \(2\dfrac{1}{2}x+x=2\dfrac{1}{17}\)
\(\Rightarrow\dfrac{5}{2}x+x=\dfrac{35}{17}\)
\(\Rightarrow x\cdot\left(\dfrac{5}{2}+1\right)=\dfrac{35}{17}\)
\(\Rightarrow x\cdot\dfrac{7}{2}=\dfrac{35}{17}\)
\(\Rightarrow x=\dfrac{35}{17}:\dfrac{7}{2}\)
\(\Rightarrow x=\dfrac{10}{17}\)
d) \(x-3\dfrac{1}{2}x=-2\dfrac{6}{7}\)
\(\Rightarrow x-\dfrac{7}{2}x=-\dfrac{20}{7}\)
\(\Rightarrow x\cdot\left(1-\dfrac{7}{2}\right)=\dfrac{-20}{7}\)
\(\Rightarrow x\cdot\dfrac{-5}{2}=\dfrac{-20}{7}\)
\(\Rightarrow x=\dfrac{-20}{7}:\dfrac{-5}{2}\)
\(\Rightarrow x=\dfrac{8}{7}\)
Bài 2:
a: \(\dfrac{5}{6}:x=\dfrac{13}{9}\cdot\dfrac{3}{26}\)
=>\(\dfrac{5}{6}:x=\dfrac{13}{26}\cdot\dfrac{3}{9}=\dfrac{1}{6}\)
=>\(x=\dfrac{5}{6}:\dfrac{1}{6}=\dfrac{5}{6}\cdot\dfrac{6}{1}=5\)
b:
ĐKXĐ: x<>-1
\(\dfrac{x-1}{21}=\dfrac{3}{x+1}\)
=>\(\left(x-1\right)\left(x+1\right)=3\cdot21\)
=>\(x^2-1=63\)
=>\(x^2=64\)
=>\(\left[{}\begin{matrix}x=8\left(nhận\right)\\x=-8\left(nhận\right)\end{matrix}\right.\)
c: \(2\dfrac{1}{2}\cdot x+x=2\dfrac{1}{17}\)
=>\(x\left(2,5+1\right)=\dfrac{35}{17}\)
=>\(x\cdot\dfrac{7}{2}=\dfrac{35}{17}\)
=>\(x=\dfrac{35}{17}:\dfrac{7}{2}=\dfrac{35}{17}\cdot\dfrac{2}{7}=\dfrac{10}{17}\)
d: \(x-3\dfrac{1}{2}x=-2\dfrac{6}{7}\)
=>\(x\left(1-3,5\right)=-\dfrac{20}{7}\)
=>\(x\cdot2,5=\dfrac{20}{7}\)
=>\(x=\dfrac{8}{7}\)